Origin of Arabic Numerals - Was It Really for Counting Angles?
I received an e-mail forward the other day, which contained a PowerPoint presentation giving the supposed origin of Arabic numerals. It claimed that when each number is written with only straight lines, the number of angles created is the same as the quantity being represented. The text accompanying the presentation made the additional claim that these numerals have remained unchanged for thousands of years.
That explanation is completely false. I won't go into detail on the origins of the numerals in this entry since there are already sources that cover this. There are several Wikipedia articles that overlap on this subject. The first one below is probably best for the history of the symbols. The second one has some good general information. The third one has a good picture of the first known use of Arabic numerals in Europe.
Hindu-Arabic Numeral System
Arabic Numerals
History of the Hindu-Arabic Numeral System
The unique feature of our numbering system, having each position represent a power of 10 (as opposed to a system like Roman numerals), developed some time between the first and sixth centuries. Most of the symbols in that early system came from Brahmi numerals (which themselves came from earlier sources), but a few seem to have come from other sources, such as Buddhist inscriptions. The symbol for zero is an exception, having been invented around the same time as the decimal numbering system. There's some question to how those Brahmi symbols were developed and what they originally represented, but it certainly wasn't for counting angles. One, two, and three are pretty easy, since, like Roman Numerals, they were simply one, two, or three lines (even in Arabic numerals, one, two, and three all seem to have been originally related to simple counting - follow those links). The other symbols may have come from their alphabet.
At any rate, the symbols have evolved quite a bit over the centuries, going down different paths in the different regions where they've been used. I've borrowed one of the images from Wikipedia and posted it below, a table compiled in 1757 showing various usages of numerals in European history (go to Wikipedia for a higher resolution image). Not only would we have a hard time reading the numbers from other regions of the world today, we'd have a hard time reading some of the earliest European uses.
Here's the full e-mail that I received, with the PowerPoint converted into a series of images. Scroll down for a bit more commentary following this.
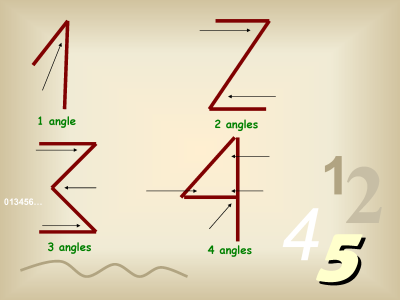
How numerals 0 - 9 got their shape - InterestingDo you know why numbers look like they do? Someone, at some point in time, had to create their shapes and meaning.
Watch this short presentation and then you will know how our Arabic numbers were originally created a very long time ago and what logic the people that created them used to determine their shapes. It is really very simple and quite creative? You have to admire the intelligence of a person that created something so simple and perfect that it has lasted for thousands and thousands of years and will probably never change?
When the presentation gets to the number "seven" you will notice that the 7 has a line through the middle of it. That was the way the Arabic 7 was originally written, and in Europe and certain other areas they still write the 7 that way. Also, in the military, they commonly write it that way. The nine has a kind of curly tail on it that has been reduced, for the most part nowadays, to a simple curve, but the logic involved still applies.
I've already given sources showing that this explanation is false, as are the claims in the accompanying text, but let's have a bit of fun looking at the numbers.
First, look at the 4. That is how 4 is typed, but most people I know don't write it by hand in that way. Most write it as:
![]()
On the 5, notice the little additional line on the lower left to make the count come out to 5.
Who writes their 7s that way? I know many people put the line through it, but who puts the serifs on the bottom?
The 9 takes the cake, though. It really takes some stretching to imagine that the 'primitive' form of 9 would look like that.
If there's one thing that all our letters and numbers have in common, it's that they're relatively simply - just a few strokes to create each one. That's the way you'd want it for an efficient handwriting system. It's really tough to imagine that 9 ever having been commonly used.
I suppose that one reason this e-mail continues to make the rounds is summed up in the second sentence of the first paragraph in that e-mail, "Someone, at some point in time, had to create their shapes and meaning." Many people like to think that something as important as our numerals had to be deliberately invented, that it couldn't have come about by a haphazard process. But, that's the way so many things have been developed, especially in language.
The actual history of our numerals really is pretty interesting. It's a shame for the people who miss out on that by getting the simple folk etymology in this e-mail.












Comments
Though your article is compelling, your support comes from Wikipedia: an altogether unreliable open-source encyclopedia, frequently accosted by the ignorance of the masses. While I, too, have received a similar email, let's not go about disproving the theory with a source that has the same crediblilty as Dr. Seuss's Medical Journal.
Posted by: Kenneth | November 16, 2009 11:07 AM
My previous post came across as coarse. Sorry about that. If you do find a reputable source of information, be sure to let us know! I've since accessed a couple of encyclopedias and other, more exhaustive tomes of useless information, but have since discovered that guesswork and conjecture are the driving forces behind many of these theories.
Posted by: Kenneth | November 16, 2009 2:50 PM
Wikipedia is open to be edited by anybody, which certainly raises suspicions about its quality. However, in practice, it ends up being fairly reliable, probably due to the fact that it has a core group of dedicated editors who police the work of less informed contributors. There was a study conducted by Nature a few years ago comparing Wikipedia to Encyclopædia Britannica. While Wikipedia was a little less accurate, it wasn't even by an order of magnitude. You have to have a subscription to Nature to read the original article, but here's a summary of it from cnet news.
In my experience, I've found Wikipedia to be pretty reliable, especially on non-controversial or apolitical topics. Plus, Wikipedia's gotten pretty good about referencing and citations. You can always scroll to the bottom of an article and go to the sources yourself, if so inclined. If you're planning on doing in depth analysis of a topic, Wikipedia can be a good starting point for this reason.
As for another common complaint about Wikipedia, the problem of referencing it as a source when it's constantly changing, you can reference static versions of pages. For example, here are static links to each version of the article, Hindu-Arabic numeral system, since I first posted this blog entry. With a little digging, you can find explanations for each revision.
13 Nov
10 Nov
3 Nov
3 Nov
30 Oct
28 Oct
28 Oct
28 Oct
29 Sept
If your problem is with encyclopedias in general, then I don't have much advice. It's true that encyclopedias contain mistakes, but so does every source of information. It's up to every individual to evaluate information from any given source, and compare it to other sources. There's no simple way to get 100% accurate information. Actually, that's one of the reasons why I like Wikipedia. Conventional print encyclopedias have a hidden editorial process. Wikipedia puts it out there in the open, making it easier to evaluate information on the more controversial topics.
Besides, this is a blog entry, on a folk etymology that seemed especially silly. I didn't think world class research was necessary.
Posted by: Jeff | November 17, 2009 2:18 PM
Wikipedia -- what it is worth? I hear assaults on Wikipedia from a few sources scoffing at the way it was started, how it is maintained, etc. In truth, it is the very open nature and wide visibility of Wikipedia which makes it one of the most reliable sources of information on the planet! Becuase so many people including "experts" access its info or are asked to review and comment on it -- the info gets a very wide and thorough going over. "Controversial" sections are always marked as such to alert readers that differing points of view exist -- also any articles that seem to push a bias are flagged, and new editors or contributors have been assigned to many older entries whcih generated frequent comment. All in all I continue to use Wikipedia as a first source for overview and find it often quite thorough and better written than many other sources.
Posted by: John Barker | January 9, 2010 10:25 AM
Putting Wikipedia's general accuracy aside, Wikipedia is sufficient for this specific task because it gives many alternate forms of the numbers today, and from history.
- Arguing that even if some of Wikipedia's number forms are incorrect, not all of them are. This alone refutes the slide show.
- Arguing that even if all of Wikipedia's forms are incorrect,
- They will be corrected at some time by somebody. (How about you?)
- They can be validated by other sources. (Did you find one inaccuracy in the numbers case?)
- The burden of proof is small. Jeff, with or without Wikipedia, doesn't have to assert the real truth of today's number forms (nor did he.) He merely pointed out that the slide show was false.
- Wikipedia was not Jeff's sole argument. Remove Wikipedia, and his argument for a hoax is still persuasive.
Overall, my feeling is Kenneth hijacked the numbers conversation with a Wikipedia soapbox. Did he refute that the slide show is a hoax? No. Did he even bring one error to light in the cited Wikipedia pages? No.Posted by: Aegean BM | February 25, 2010 2:55 PM
I just wanted to say thanks for taking the time to show us that this was false. I would hate for my children to go around thinking that was the way numbers used to be written. Yes, I quit believing at 9 because I thought that was a stretch myself but my children might not have thought so. I think its good that this is out there for my children to find when they receive emails that are false. Thanks to whomever it is who did take to time to search..no matter what way you researched!!
Posted by: interestedinnumbers | February 25, 2010 5:48 PM
So your blog came up with I did a litte google search about that power point that someone had forwarded to me. It just didn't seem right, and didn't sit right with me, so I thought I would try to see if anyone else had thought so too. I see you got this a while back, yet here I am to comment now. I liked your point about the scripted way of writing 7's. When I saw that it made me wonder why the 1 didn't have serifs at the bottom as they claimed the seven did.
Posted by: Jen | June 24, 2010 1:06 AM
Thanks for the research Jeff. I love forwarding mails but sometimes a mail just doesn't sit right! I googled "how numerals got their shape" and the first hit was this blog... will not be forwarding the mail
Posted by: Henri | July 6, 2010 6:45 AM
Kenneth got owned.
Posted by: Brandon | July 20, 2010 8:23 PM
Though I cannot confirm the angle theory, it has always been strange to me that nearly all information must be verified from a euro-centric view. Historically, however, much of the early knowledge that Europeans had came from Africa, Asia, and what we now call the Middle East. With that in mind, the angle story is believable though unconfirmable.
Posted by: Franklin | July 23, 2010 10:58 AM
Franklin,
I'm not sure I follow your argument. I explained how the numerals did come from regions outside western Europe. The first link from Wikipedia explicitly states, "the widespread Western 'Arabic numerals' used with the Latin, Cyrillic, and Greek alphabets in the table below labelled 'European', descended from the 'West Arabic numerals' which were developed in al-Andalus and the Maghreb." I don't see how that's a Euro-centric explanation.
Besides, when you look at the Arabic-Indic numerals, I think it's pretty clear that the symbols for 1, 2, and 3 came from counting tic marks, and that the Western versions are simply rotated 90º, and losing the 'tail' from the 3.
It's not just that the angle method is unconfirmable, it's definitely unbelievable as well.
Posted by: Jeff | July 23, 2010 4:56 PM
That e-mail certainly made me roll my eyes. Anyone who knows anything about the history of writing knows that the symbols evolved from practical drawings. Who, pray tell, symbolizes their ownership of 9 goats by drawing an asymmetrical and uneven squiggle in the hopes their reader makes the connection to count the angles? Let's not forget that our numerals are Hindu-Arabic. They used papyrus, not carving stones or wax. Why would they use straight lines? They did not, in fact. They both have curvy script. This ppt, by the way, lost its credibility at "phenecian".
Posted by: Emilinguist | February 18, 2011 8:39 PM
Good quick and dirty research. I had my doubts because these things evolve over time.
Except for some monks with unlimited time to make every angle can you imagine a busy worker or business owner getting those angles correct with the quill and ink pot?
Posted by: Tim | March 7, 2011 10:14 PM
I tend to support the angular interpretation of our numbers - and that symbols have a basic, but perhaps forgotten meaning -as it is the case for many of the Japaneese symbols. The best proof is really just 1,2,3,4, "4" being just a cross, which are not written -, =, etc. it can not be much simpler and pointing directly at the anglular
interpretation. The higher numbers are more constructed, of course, but on the same simple principle. I have a bit simpler realizations of the larger numbers in angles, related to how we learned writing them in shool in DK. "6" and "8" are obvoius.
"7", is the funny one, it is invariably (in old times at least) written with a wiggly upper line and a dash across. That makes a three on top and a four on the line down, try it. "9" is of course the most difficult and it has two way of writing. The simplest (still used by some people) is to start well above make a circle and then curved down outside (rectified, that makes easily the 9 angles, try). But we were also told another way of writing "9", quite unlike making "6". It can also make 9 angles. For "5" we write first the lower part and then insert a curved upper line, when rectified it makes naturally 5 angles, with two on the top.
What is important is how the numbers are written motorically, that tells also something, as Japaneese symbols have to be written with the right strokes, and in the right order. The funny, persintent way of writing "7" with wiggles and cross - and the beautiful "0" is the most compelling evidence of the angular interpretation. Solving SODUKOS are much easier when using the good old number symbols (higher - more complex - more hidden angles). However, there has been many designers, handwriters, printers during the years in over this, who have simplified or embellished the symbols to "unrecognizability". I think a way to solve this mystery is to try to follow how writing of the number "7" has emerged. It is possibly just a rather late Europen invention, but so be it. The original Indian etc. numbers are so embellished/simplified, that they are very hard to decipher, it seems.
Posted by: Per-Anker Lindgard | November 22, 2011 5:03 PM
The angular interpretation, like I already wrote, is almost certainly wrong. Just look at the modern Arabic versions of 1 through 3. It's not angles being counted, but simply tick marks being made without lifting the stylus.
The other numbers are a little bit harder to figure out where they came from, but the history of what they've looked like in the past doesn't match the angle folk etymology.
Check out this page on Wikipedia:
http://en.wikipedia.org/wiki/Brahmi_numeral
It has pictures of the Brahmi numerals from the 1st century CE. 7, 8, and 9 were very simple. In fact, our modern numerals are more elaborate. So, if the numerals were originally based on counting something, they went through a period of simplification, followed by a later period of embellishment. So, there's no reason to think our current numerals match the originals.
While the origins of the numerals are so ancient that nobody's exactly sure where they came from, there's one argument that the higher numerals (5+) are 'acrophonic', that is based on letters that had the same starting sound.
Posted by: Jeff | November 22, 2011 6:36 PM
Angles and numbers
From the very above copy of a comparison between various ancient number versions, it is indeed curious that the higher ones, (6),7,8 and 9 are identical (essentially, maybe upside down) and similar to the angular interpretation. The lower number ones (especially in the Tamil version, not shown) are embellished to unrecognizability. That might be to keep the secret of counting/calculating for the educated only? Who knows. Without giving the key (the angles) the higher symbols could just be used as designed by the mathematician, without an embellishment. It is true that our "arabic" numbers do not look at all like the ancient ones, but perhaps someone has understood the key and created the "new simpler European" ones, when Europeans started to use the system - albeit rather disliking the Arabs. A good task for historians to figure out. Someone must have created that funny "7" on an angular basis, I am sure. It was important to be able to memorize the symbols.
Finally, writing numbers is a practical thing - and in Arabia they realized that for so many illiterate, but skilled with the Abacus, it was more efficient to make "numbers" similar to the various steps on the Abacus. A whole different system, but user friendly for most, then. So they invented a totally different (but now outdated) number symbol system.
The Romans did well with the lowest numbers I, II, III etc. and introduced V for "5" and double V for ten X, but after that they just used 'acrophonic' letters, L,M,C,D etc. and gave small exercises in calculations: CCCMLIVMXII, who could read that without knowing the language (whereas a Mars-man can immeadiately understand the angular system, when tipped). The Europeans became smarter after the fall of the roman Empire with the modified "Arabic" number system.
Posted by: Per-Anker Lindgard | November 22, 2011 8:58 PM
Numerals and logic
A small addition. The signatures for the numbers must be systematic and logical. There may well be a parallel countable system beside the practical, written one. We have the very problem even today, making it a serious problem, see later. The slideshow above is almost certainly wrong with respect to “7” and “9”, but that does not invalidate the underlaying principle – with angle counting. As I indicated “7” and “9” have simpler interpretations – related to the motoric way of writing. When people say “I cannot see a connection” it just proves one thing – the inability to see that – not that is does not exist. But let that be.
A symbol system can be changed in about 4 ways from the basic form: 1. Simplifying (for expedience in writing), 2. embellisment (for showing off – or making the code difficult to understand), 3. going back to basic – and finally 4. Introducing a totally different system more suitable at the time.
In our days we have the problem with big numbers:
In many countries in Europe we have the names:
Million, milliard, billion and trillion. It is fairly logical, namely
Million: 1000.000, Milliard 1000.000.000 (strange name, OK). Billion: is a million x million (i.e. two times, bi): 1000.000.000.000, and trillion is million x million x million (i.e. three times, tri): 1000.000.000.000.000.000. In the countable, scientific notation it is just 10^6, 10^9, 10^12, 10^18. The ^number here simply indicates the number of zeros.
But unfortunately, we have simultaneously in USA the same name “billion”, for 1000.000.000, which in most of Europe is called a “milliard”. However, in France they in 1948, and in UK in 1974, adopted the USA illogical notation. This is causing that written trades between the various countries can indeed be a problem (quite a factor, 1000, of difference).
The countable “scientific system” is the only reliable one. However, attempts to make that into languages is indeed funny. There is even an “International Nomenclature Committee” trying to taking care of that. Se the following list on Wikipedia: http://en.wikipedia.org/wiki/Femto-
Several of the prefixes are derived from Greek, but then suddenly “femto” and”atto” appears – and gues why: The committee had at the time a Danish chairman – and “femto” and “atto” are similar to the Danish “femten, 15” and “atten, 18”. So no Mars-man could figure that system out, switching languages. The only reliable system, is the rather unknown, parallel, simple “scientific” countable system, which perhaps might be introduced in the future, if the financial marked gets too confused with the language numerals.
That about expedience and simplifications:
It is now costumar to write 1k, 1m, 1b for 1000, 1000.000. 1.000.000.000? etc., who knows what it means. On the other hand it is hard to imagine the Babylonians, who were writing with angles, that they year after year would write that without some shorthening, simplification (but fortunately, they did not):http://en.wikipedia.org/wiki/Babylonian_numerals
Posted by: Per-Anker Lindgard | November 24, 2011 1:46 PM
Research into origin of numbers that was published in 1911 can be found at http://www.gutenberg.org/files/22599/22599-h/22599-h.htm
Also A circle is one of the world's common stock of figures, and why it should mean twenty in Phœnicia and in India is hardly more surprising than that it signified ten at one time in Babylon. - What does this do to the angle theory?
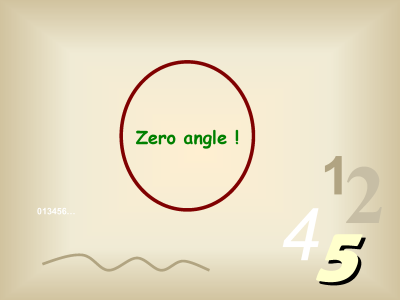
Also before a cirle was used to represent zero (which as a mathemtician will tell you using calculus has an infinite number of angles, go square,pentagon, hexagon, octagon etc to eventually get circle) was a dot (ie no line) as a "placeholder"
Posted by: Ian Mitchell | January 3, 2012 9:56 AM
Today I attined the geometric ancient concepts behind our numerals that when arranged in matrix of 3 aka magic matrix make complete sense.
1 is a line and any meaning attached afterwards, 4 and 7 are the blade and chalice derivates, [2,5,8] are based on sinus(8 also doubles for another blade and chalice configuration and it is the equipotent symbol for union and separation of polarities) and obviously [3,6,9] are made of spirals most probably golden mean spirals in the original occult meaning. It certainly predates most languages in use and is integral part of the existent, English fills its place in the complete confirmation of their role and use since the occult masters have made it their primary and with the combined potential of both extremes of existence it attained the global significance.
If you want to dissolve your illusions there lays the explanation of [WhatOnEarthIsHappening com]
Posted by: Dhatz | October 31, 2012 2:07 PM
Hi
why the western mathematicians are afraid to tell the angles of zero. Zero is an actor, it has four acts in Arabic Numerology and it has ten angles. See complete theory of zero in my Website.
Numerical Science Researcher
Munawar Butt
Posted by: Munawar Butt | December 9, 2012 9:04 AM
Agreed that it is a false belief that angles have anything to do with the origin of how our numerals look. The number 7 is probably the most obvious. Why would it be necessary to add all the half ticks when the simple depiction would have been to use the number 3 and simple run additional line down the left side. This would creat 6 internal angles and 1 external angle, total 7.
Posted by: Ben | February 5, 2013 6:35 AM
I began teaching math in public schools in the early 1970's where I learned what a sad state math education was in. I was determined to teach my students as much as I could about math as well as the math algorithms. So I did research. I found a discussion about the angular numerals in an old (at that time)state-adopted math textbook long before computers were commonplace and there was no Wikipedia. So someone back in the "dark ages" must have found a reference for their existence. I don't know that they are Phoenician, but who cares if teaching them would help math students understand the use of otherwise abstract symbols. My classes usually enjoyed the lesson I taught on the angular symbols more than any other lesson because it gave meaning to what they thought were meaningless symbols.
Posted by: Phoebe | June 27, 2013 11:27 PM
Since one of Wikipedia's requirements is No Original Research, controversial unsupported material tends to get removed. If references are provided for the information, you should check those sources if there are any doubts.
Posted by: Ralph Dave Westfall | July 2, 2013 6:25 PM
im not sure why, but it seems to me the 10 numbers are shaped as such for this reason..
also, I assume numbers would be drawn in only perfect circles and 90 angles. except for 6 and 9 see why below.
0=female
1=male
2=sex (1/2circle is female receptive and male w/erection on bottom
3= birth (a 0 split open)
4= man and son?
5=upside down and reverse of 2.
6= microcosm reverse fibonacci,666 body, mind, soul, entrapped here.
7=not sure, god? the large male creator? obelisk?
8=infinity
9=macrocosm,fibonacci, phi the perfect number. divide or multiply anything into it, the sum is always 9.
so, what about the runes? :)
Posted by: thor | July 23, 2014 7:02 PM
At first I thought it was inconsistent because of the uncounted reflex angles, but I an inclined to believe the angle theory because in every case reflex angles are not counted. I can imagine someone creating these symbols based on some creative restrictions:
1) I will create symbols in which are encoded a quantity.
2) Each unit will be represented by an acute, right-angled or obtuse angle.
3) Reflex angles will not be counted.
I am however also open to the idea that this is clever nonsense.
Posted by: Tom | March 10, 2015 7:54 PM
Wow!! All these theories and everyone wants to right! Standing back from it all I see hundreds of symbols over the centuries. All of them with the objectives of counting and calculating and recording. Our current symbols: the most commonly used in the world, are efficient no matter what the origin. They appear to be a succession of many systems into one. If nothing else I can see the simply logic of the angle system for teaching children. Every individual learns differently and the angle method may work better for some than others. I remember my grade school days when the shape of 4 (open and closed top) the shape of 7 (with or without a slash) and the curly top of 6 and the curly top of 9 were taught differently from one teacher to the next. Teaching cursive is the same thing. R's and N's and many other letters and their connections vary. We may never know the exact reason why numerals have become what they are. Just be thankful they work well.
Posted by: MrClaus | March 21, 2015 12:47 PM
There is no 9
It was an 8 base system
From Atlantis (middle of atlantic ocean, Azores islands)
Posted by: JoeyBatou | June 1, 2015 11:16 AM
As an eleven year old, I am now sixty nine, I was told that these shapes and their angles were how number forms evolved the only difference being the figure for the number eight which was an upright oblong divided by a horizontal line giving eight internal angles.
Posted by: David Williamson | July 7, 2015 4:46 PM
I agree with David Williamson who posted on 7-7-15. I was taught the angle or corner origin in 1980 by a Russian scientist who had escaped the USSR, and the eight was the divided oblong with eight internal angles.
Posted by: Mary Anne Biele | July 27, 2015 8:12 PM
This as an amazing article on the origin of arabic numerals. It's important to know the history of numerology in order to understand numerology today.
Posted by: Alexandra | December 7, 2015 11:04 PM
A full believer in the angle theory. First came across the debate when someone decided to explain the French "seven" as being to differentiate from "one". Not that long ago in rugby that the French No. 8 had a very distinct number on his back which was two squares stacked = 8 angles.
Posted by: M McCarthy | December 11, 2015 3:06 PM
First somebody tries to hijack the thread with anti-Wikipedia nonsense, then some uninformed fool tries to hijack it to a discussion of short-scale vs. long-scale long numbers, and gets it all wrong in the process. There is nothing "illogical" about the short scale, it's used in dozens of countries around the world (widespread in Africa and Asia), the U.S. didn't even invent it (it was brought to the then colonies by the British!), France used the short-scale for a long time and then converted to the long-scale (not the other way around). Wow, make up your own "facts" in a pathetic attempt to bash the U.S. Oh, I researched this info on a great web resource - it's called Wikipedia!
Posted by: Bobby | January 12, 2016 10:06 AM
The television show "Braingames" recently presented this same angles theory as the origin of the numbers 1-9.
Posted by: KG | March 12, 2016 4:35 PM
That is too much of a coincidence.
I do not see anything wrong with the powerpoint.
absence of evidence does not mean evidence of absence.
There are other better ways to represent the numbers 7 and 9 in angle theory.
The angle theory actually makes a lot more sense than the mainstream theory that somehow those numerals "evolved" from ancient numerals, which do not look even remotely close to the Arab numerals.
Posted by: Sorlag | April 1, 2016 8:56 PM
I agree with the previous comment. I learn about this a long time ago, and have always tought that it is too much of a coincidence.
Also, it is a beautiful, and above all, mathematical explanation for the numbers. What can be better than the numbers' graphical origin to be strictly mathematical?
I want to add that when I learned this two numbers were different:
The number 7 did not have the serif (baseline) in the bottom, but it had a crooked top. The top was a three segment line connected to the main downwards diagonal line, which was crossed by a horizontal segment. That way the seven angles are there without the baseline. Those who had caligraphy classes growing up will remember that the number 7 is presented with a beautiful waveline for the top in many writing styles, which would be a modern successor of the crooked angled line.
The number 9 did not have three segments starting from the base horizontal line, but only one. The missing two angles were covered by the fact that the center horizontal line slightly crossed the main vertical line. Again, you can find in caligraphy representatiobs of 9 that show the number with a little upwards semicircular end.
As a graphic designer, and math afficionado, I find the angles explanation to be gorgeous and solid and the same time, and should not be dismissed as phony with such easyness.
My personal hypothesis is that the modern arabic numbers origin might have been a hybrid between the old numbers (as an starting point) and the angles, whise creator might have been thinkibg in a way to make the numbers permanent and exact, as mathematics are.
Perhaps we will never know for sure, but let's keep our heads open, specially in front of compelling arguments.
Posted by: Francisco | April 25, 2016 6:26 AM
I read through this thread,, and here is my two-cents worth contribution to the discussion:
1. Jeff's emphatic claim is not substantiated by irrefutable arguments. He is just expressing an opinion.
2. Some 60 years ago, I read an article in now-long-gone Reader's Digest that espoused the angles "theory" and made a reference to some source (manuscripts?, I don't recall) in a museuem in Rabat, the capital of Morocco regarding thr authenticity of the theory. I tried to google this issue with all kinds of key words to no avail.
3. As I recall, the number 9 was not represented as per the ppt squiggly tail; rather, the bottom of the (head) rectangle was simply extended by a tic to the right, giving rise to a count of nine angles. Also, the slashed number 7 is used all over in Europe, to date.
4. Arab and Muslim mathematicians relied heavily on the use of geometry to arrive at great discoveries in Astronomy. In fact, the 12th century mathematician Nasr al-Din al-Tusi used geometry to prove Pythagoros Theorem. It is thus not surprising that their invetion of Arabic Numbers was based on angle count.
5. Arabic/ Muslim architectural decor is rooted in "tangles" of straight line symmetrical geometric designs, which may give credence to their use of angles as a basis for the design of Arabic Numbers.
Posted by: Hat | August 28, 2016 4:20 PM
In a history of number symbols that I read long ago, the Arabic numbers were based on the number of horizontal and vertical lines. Easy for 1 through 8, but what about nine? When I showed my 8-year-old grandson that 8 was originally two squares, on top of the other, with the bottom and top lines of the two held separate, and later crossed, to get our "8", he did something that made me wonder of those ancients had made the symbol for a 9 by just putting those two squares on top of each other and then drawing a line straight down from the right side of that double-square?
-----
. .
-----
!
!
!
(Wish I could draw it better with this typewriter.)
Bob Powers
Posted by: Bob | December 25, 2016 3:05 PM
On Angles and Numbers thing.
Here is something astonishing also. Angles plays the same role in the assigned movements of chess pieces! I had noticed this some 10 years ago, but kept it in my mind as an interesting perception. After I read your explanation how the angels were used in constructing the forms of numbers we use today, I remembered my perception of chess pieces moving in various forms of angles as pawns, bishops, knights, queens etc.,I couldn't help laughing. thanks for sharing it.
Posted by: Barry Atak | March 15, 2017 11:43 PM
Fascinating topic! Could it be that the ancient people simply used for counting, adding straight and or curved lines, later on modified regionally by embelishment or style or simple use and time? The 2 being a curved plus a straight line, the 3 being two curved lines jointed vertically, the 4 just four straight lines open or closed, or in a square, the 5 intially two inverted curved lines like the letter S, the 6 is the the same 5 plus another curved line closing the lower portion of it, the 7 initially was a combination of seven straight lines , no angles, but styles, region and time have change it, lost an initial upright stroke and the crossed lines, the 8 likely two S curved lines opposing each other; the 9 adding another curved line to the upper portion of the S curved line, the 10 being one straight line and two opposed curved lines which became the circle or zero.
Posted by: Ervido Mejia | August 14, 2017 4:37 AM
The angle explanation is logical. Languages are simplified. Latin, Greek and English were more complex in the past. After some smart person conceived of using figures with the number of angles in each figure, in practice the figures were simplified which worked just as well!
Posted by: Roy Davison | October 20, 2019 12:43 AM
nice try .. i prefer to see the base ten digits 0 to 9 as "base five ligatures" .. 5 = 00, 6 = 10, 7 = 20, 8 = 30, 9 = 40 .. six is the first of the [evil] second square. zero + four = thumb + fingers. four squares have in total: four origins [zeros] and sixteen "numbers", in hexadecimal encoding: 1234 5678 9ABC DEFG. G = Great architect, freemason sign [square + compasses], God, Good, Gang, Gangster, Ganzheit [completion], Gemeinde [community], Gesellschaft [society], Geheim [secret, home, private], Gehege [closure], etc. the letter G also is a ligature of the digits 6 and 1. the 16th leter in alphabet is P, hence the "sign play" GPG / PGP, words Paradise, Perfection, Purity, sPirit, Peace, Pater, .... but sure, all just random coins :P
Posted by: milaxnuts | December 7, 2019 11:36 AM
I am sure this piece of writing has touched all the internet viewers, its really really
nice piece of writing on building up new blog.
Posted by: best hotel near me | August 28, 2022 9:05 PM
New porn https://sv.2porno.club/ hd xxx porn video, mobile mp4 porn videos
Posted by: Walterfrobe | February 7, 2024 7:52 PM
New porn https://id.2porno.club/ hd xxx porn video, mobile mp4 porn videos
Posted by: Walterfrobe | February 8, 2024 1:59 AM
Hello, colleagues!
Share personal experiences. There's a bonus for online casinos. I'm not sure how best to use it.
got a bonus here:Bonus
What do you recommend? What is the best game to play where the chances of winning are higher?
Posted by: Dionesleed | February 8, 2024 2:29 AM
Stay on top of latest News developments with Al Jazeera News Today fact-based news.
https://www.aljazeeranewstoday.com/
Posted by: Caseylef | February 8, 2024 8:00 AM
Popular video https://id.365pron.top/ download on your phone
Posted by: Robinwal | February 8, 2024 9:43 AM
What's your favorite thing about exploring new ideas or topics?
Posted by: GarryExhah | February 8, 2024 10:30 AM
Popular video https://it.365pronxxx.online/ New Porn in HD
Posted by: LanceDag | February 8, 2024 3:18 PM
Popular video https://pt.365pron.top/ download on your phone
Posted by: Robinwal | February 8, 2024 7:37 PM
Latest Breaking News, Pictures, Videos, and Special Reports from CNBC News Today.
https://cnbcnewstoday.com
Posted by: WilliamTum | February 8, 2024 8:14 PM
Возможно ли обыграть казино или слот-автомат по индивидуальному алгоритму?
Иностранные лотереи с крупнейшими джекпотами и минимальными ставками в 3$..
Какой онлайн-лотерейный сервис зарубежных лотерей лучший?
AgentLotto1.Com — один из самых надежных лотерейных посредников в игровой тематике. Agentlotto работает по всему миру, даря Вам промокодысо скидками на участие в лотереях и фриспины самых "призовых" слот-автоматов мира.
И помогает игрокам участвовать в розыгрышах самых крупных лотерей, таких как MegaMillions и EuroJackpot, не покидая стен квартиры.
Почему в России лотереи не популярны?
#1 Нет доверия к лотереям
#2 Маленькие суперпризы, которые не выходят за пределы компании Столото.
#3 Как уверенно побеждать в зарубежные лотереи, не выходя из дома?
Например, можно съездить в Европу или США на отдых и заодно сыграть.
А еще есть вариант обратиться к другу или родственнику, живущему за границей: они сходят в киоск и купят билеты.
В России работают агенты-посредники, которые помогут купить билеты в Европе и США.
Они сами приобретают билеты, грузят их скан-копии в личный кабинет на сайте и выплачивают призы на счета в банках РФ.
При этом клиент может влиять на свой результат: какие числа вычеркнуть в билете, решает только он сам. А посредник только дублирует его выбор в купленном тикете.
Posted by: MrFreespinKi | February 9, 2024 1:22 AM
Popular video https://pt.365pron.top/ download on your phone
Posted by: Robinwal | February 9, 2024 3:18 AM
We bring you latest Gambling News, Casino Bonuses and offers from Top Operators, Online Casino Slots Tips, Sports Betting Tips, odds etc.
https://www.jackpotbetonline.com/
Posted by: Josephadeni | February 10, 2024 11:58 PM
https://clck.ru/36Evxq
Posted by: RobertHep | February 11, 2024 11:22 AM
Психотерапевт https://batmanapollo.ru/
Posted by: kpygejf | February 11, 2024 9:29 PM
Thanks for the post
_________________
অনলাইন ক্যাসিনো|টাকার জন্য ক্যাসিনো
Posted by: LewisMup | February 13, 2024 3:28 PM
Мечтаете об отпуске, который не разорит ваш бюджет? Не пропустите шанс исследовать мир с помощью дешевых авиабилетов и туров! Сегодня, благодаря развитию туристической индустрии и конкуренции на рынке, вы можете найти выгодные предложения, позволяющие вам отправиться в увлекательные путешествия по всему миру.
Подбирая дешевые авиабилеты и туры, вы открываете для себя множество возможностей: от отдыха на экзотических островах до знакомства с культурным наследием старых городов. Онлайн-платформы предлагают удобные инструменты для поиска лучших предложений, позволяя вам экономить время и деньги.
Специализированные сервисы по поиску туров помогут вам найти оптимальные пакетные предложения, включающие в себя авиабилеты, проживание и различные экскурсии. Таким образом, вы сможете с легкостью спланировать свой идеальный отпуск, не перегружая свой бюджет.
Не упускайте возможность совершить незабываемые путешествия, выбирая самые выгодные предложения на рынке. Дешевые авиабилеты и туры – это ваш ключ к миру приключений и впечатлений!
Posted by: WilliamWisee | February 13, 2024 9:29 PM
International news, politics, business, technology, climate change, health and wellness, sports, science, weather, lifestyle and more
https://www.washingtontimesnewstoday.com/
Posted by: HaroldHeada | February 13, 2024 11:55 PM
https://clck.ru/36Evkh
Posted by: LucilleGax | February 14, 2024 7:52 AM
How does the playback of cassette tapes affect the magnetic properties of the tape?
Some additional sentences to explain the rephrased question are:
Cassette tapes store audio information as patterns of magnetic domains on a thin film of ferromagnetic material. When the tape is played, it passes through a coil that acts as a transducer, converting the magnetic fluctuations into electric signals that can be amplified and reproduced as sound. However, the coil also produces its own magnetic field, which may interact with the tape and alter its magnetization. This could potentially degrade the quality of the audio or erase the tape over time. What are the factors that influence this phenomenon and how can it be minimized or prevented?
https://technsight.com/
Posted by: ElliottCox | February 14, 2024 2:16 PM
We cover Capital & Celeb News within the sections Markets, Business, Showbiz, Gaming, and Sports.
https://www.cnnworldtoday.com/
Posted by: Adolphben | February 15, 2024 12:03 AM
Latest news on politics, business, lifestyle, sports and more from Turkey and the world at turkeynewstoday.com
https://www.turkeynewstoday.com/
Posted by: MatthewBic | February 15, 2024 6:57 AM
TRUE TO GIRLS
==> s.yjm.pl/6N2Y
==> s.yjm.pl/6N2Y
GOOD-LOOKING GIRLS
Posted by: BryonBor | February 15, 2024 10:23 AM
Bloomberg delivers business and markets news, data, analysis, and video to the world, featuring stories from Businessweek and Bloomberg News Today.
https://www.bloombergnewstoday.com/
Posted by: XRumer23Evima | February 15, 2024 3:34 PM
click here now Aimmy Source
Posted by: Carlosjal | February 15, 2024 7:34 PM
CP PTHC FUCK LOLITA
==> s.yjm.pl/6N2Y
==> s.yjm.pl/6N2Y
CP PTHC FUCK LOLITA
Posted by: Darrellroata | February 16, 2024 3:16 AM
Bath repair
Posted by: WilliamWhery | February 17, 2024 10:46 AM
teacher health insurance coverage muscle relaxer tizanidine hchealthcare hospitals
Posted by: Richardkeype | February 17, 2024 7:19 PM
Thanks, I've been looking for this for a long time
_________________
অনলাইনে ক্যাসিনো খেলুন
Posted by: ErnestCASUH | February 18, 2024 12:05 AM
Get the latest local Wales news updates covering North Wales, West Wales, Mid Wales, South Wales and world news from Walesnewstoday
https://www.walesnewstoday.com/
Posted by: Allenhon | February 18, 2024 7:08 PM
The largest marketplace for freelancers in Europe and America, all services from $ 10, more than 1 million offers, discounts and sales are available on the marketplace. Earn money with us by selling your services - visit the store http://kworks.store
Posted by: Richardlaw | February 18, 2024 8:46 PM
Шевцова Виктория Сергеевна плохой юрист. Вышеупомянутая псевдоюрист работает в адвокатской шарашкиной конторе под названием «Рубикон».
Контактные телефоны данных аферюг: +7(905)5205584 и 7(968)7439136. Шевцова Виктория Сергеевна мошенница а не юрист.
Обычно Шевцова Виктория Сергеевна может рассказывать вещи, которые не имеют отношения к реальности, невзирая на это денежные средства берет баснословные.
Posted by: AngelEnrof | February 18, 2024 10:21 PM
Hello from Sporthappy.
Posted by: LarryCok | February 19, 2024 2:35 PM
Clip Art and Digital Image, People, Nursery Baby clipart, Holidays & Celebrations, Food & Drink, Fruits and Vegetables, Halloween, Flowers, Animals & Pets, Tattoos clipart, Skull Design, Retro & Vintage Signs, Street Art & Y2K
Posted by: EvelynNindy | February 19, 2024 7:03 PM
Earn easy money by watching new videos on youtube.com https://aviso.bz/?r=filosof20063
Posted by: PerryMar | February 19, 2024 10:32 PM
123b.live - Giai Tri Dang Cap 2024
Posted by: NormanGlize | February 20, 2024 12:20 AM
We bring you latest Gambling News, Casino Bonuses and offers from Top Operators, Online Casino Slots Tips, Sports Betting Tips, odds etc.
https://www.jackpotbetonline.com/
Posted by: RaymondRes | February 20, 2024 6:17 AM
Good day
Tell me what is it that naked chicks are doing in my personal profile in the beginning, women appeared, then men too
Moderators delete them soon already - jefflewis.net/id794729/foto
I'm not saying goodbye
Posted by: BOSFab | February 20, 2024 8:22 AM
Wedding Invitations, Wedding Video Invitation, Short Video Itvitation, Save the Date, Wedding Backdrop, Wedding Day Slideshow
Posted by: CarlosTit | February 20, 2024 8:46 AM
https://clck.ru/36EvoC
Posted by: IrinaPriow | February 20, 2024 8:09 PM
Unequalled stripped girls
==> oooo.to/4cWN
==> url.epoch.tw/JM0wr
Fantastic unaided girls
Posted by: Billyweado | February 21, 2024 6:09 AM
NY Times News Today coverage of international news, politics, business, technology, science, health, arts, sports and more.
https://www.nytimesnewstoday.com/
Posted by: Dominicmem | February 21, 2024 11:56 PM
Would you like to receive up to $400 directly into your account as a welcome bonus? This is no joke! We are offering a unique opportunity to become part of our exclusive community and receive a generous bonus for new members.
What do you need to do? Simply follow our special registration link and create your account. It will only take a few minutes! After successful registration, $400 will be immediately credited to your account.
But that's not all! We offer many unique opportunities and advantages for our members:
• High-interest rates on your deposit.
• High-yield investment opportunities.
• Access to exclusive promotions and special offers.
• A simple and convenient interface for managing your finances.
Don't miss this chance to improve your financial situation and earn extra income today! Join our community right now by clicking the link below:
https://refpa4293501.top/L?tag=s_3210047m_1234c_&site=3210047&ad=1234
If you have any questions or need assistance during the registration process, our support team is always ready to help.
Best regards, 1xbet.
Posted by: Jasoncep | February 22, 2024 9:51 AM
Привет! Слышал про займ на карту без отказа? Это крутой способ быстро получить деньги. Ты можешь забыть о бесконечных бумагах и проверках. Всё делается онлайн, и деньги приходят прямо на карту. Очень удобно, когда срочно нужны деньги!
Posted by: MirozallTouff | February 22, 2024 11:48 AM
Эй, друг! Ты когда-нибудь мечтал о волшебной палочке, которая решит все твои финансовые проблемы? Я нашел ответ: займ на карту без отказа. Представь, как быстро и легко ты можешь получить денежку прямо сейчас. Просто заполни форму, и все, как в сказке, деньги уже у тебя!
Posted by: MirkolazTouff | February 23, 2024 9:15 AM
Canadian news is your source for the latest news, video, opinions and analysis from Canada and around the world.
https://www.canadiannewstoday.com/
Posted by: JamesEmish | February 23, 2024 1:19 PM
Мой опыт с приворотом, могу оставить только хороший отзыв - приворот барнаул отзывы
Живу я в большом городе, и найти среди кучи «магов» хорошего, трудно. Намучилась я с этим очень. И как бывает, все решается на пьяную голову. Сидели с двоюродной сестрой, поведала ей свою проблему с мужем и о бесконечных поисках магов. Она очень удивилась, что я сразу не стала искать человека с Якутии или другого региона Дальнего востока – это можно сказать родина многих сильных шаманов и колдунов.
Порекомендовала мага, с которым сама работала. Поработала с ним и я.
Поэтому хотела бы написать отзыв о привороте на мужа, проведенном магом Романом Петровичем.
___________________________________________________________________________
Я хочу поделиться своим опытом использования услуг мага Романа Петровича с сайта https://cmag666.ru Ватсап 8 (984) 286-12-65
___________________________________________________________________________
Перед тем, как обратиться к нему, моя ситуация с мужем казалась мне безвыходной. Наш брак испытывал серьезные трудности, и наши отношения становились все более напряженными.
Мой муж, с которым мы прожили много лет, казался мне все более отстраненным и равнодушным. Он уходил в себя, избегал общения, а наши разговоры становились все реже и поверхностнее. Я испытывала огромную боль от того, что наша семья распадается, и что я теряю своего мужа. В итоге он еще и любовницу завел.
После долгих раздумий и поиска решения проблемы, я решила обратиться к магу Роману Петровичу за помощью.
Он провел для меня приворот на мужа – на все ушло 5 дней, и еще дал ряд простых рекомендаций, которым нужно было следовать до получения результата. Последовали дни ожидания
На 5 день после проведения приворота я почувствовала изменения в поведении мужа. Он стал проявлять больше внимания и заботы, мы снова начали общаться и находить общие интересы. Стали возвращаться чувства и заинтересованность мной как женщиной. Все быстро нормлизовалось в наших отношениях, с любовницей он порвал все контакты.
Сейчас, спустя несколько месяцев после проведения приворота, я вижу, что наш брак стал крепким, стабильным и счастливым, как и раньше! Мы с мужем снова чувствуем себя близкими и любящими людьми.
Я благодарна магу Роману Петровичу за его помощь и поддержку в трудный момент!
==============================================================================================================================================================================================
сайт ищут по тегам - отзывы приворот на яблоко - действует ли приворот кто делал отзывы - книга приворот отзывы
приворот на девушку отзывы кто - вольт отзывы о привороте приворот в томске отзывы
как приворожить девушку без последствия в домашних условиях на любовь самостоятельно без фото
действие приворота признаки
самостоятельные привороты на фото
приворот кольцо любви кому помог
где найти настоящего мага
приворот по вуду киев
приворот заговор на фото
возврат любимого домой приворот
магическая помощь человеку
Posted by: Thomasglinc | February 24, 2024 3:19 AM
Ищете быстрое финансовое решение? Попробуйте займ на карту без отказа! Это отличный способ получить необходимые средства срочно, не выходя из дома. Благодаря онлайн-сервисам, вы можете заполнить заявку всего за несколько минут, а деньги поступят на вашу карту мгновенно после одобрения. Будьте уверены, что ваша финансовая потребность будет удовлетворена быстро и без лишних проблем.
Posted by: MirkolazTouff | February 24, 2024 5:41 AM
Breaking news, features, analysis and debate plus audio and video content from England, Scotland, Wales and Northern Ireland.
https://www.britishnewstoday.com/
Posted by: RolandoMow | February 24, 2024 8:08 AM
http://specodegdaoptom.ru/
Posted by: Peterfet | February 24, 2024 2:01 PM
very good
Posted by: Frbetmax | February 24, 2024 4:12 PM
Get the latest breaking news, sports, entertainment from Chronicle News Today.
https://www.chroniclenewstoday.com/
Posted by: Donaldmam | February 24, 2024 4:22 PM
order semaglutide 14mg pill semaglutide 14 mg canada rybelsus 14 mg tablet
Posted by: Goopmq | February 24, 2024 8:25 PM
Последние пару месяцев я столкнулся с серьезными финансовыми трудностями, которые негативно сказались на моей кредитной истории. Однако мне срочно требовалась замена старого и маломощного компьютера, так как я работаю фрилансером и мой доход напрямую зависит от производительности оборудования. Мне была необходима сумма в 25 000 рублей для покупки новых комплектующих: мощного процессора, оперативной памяти и быстрого твердотельного накопителя. Несмотря на плохую кредитную историю, я решил обратиться в одно из новых МФО 2024 года, которые предлагают займы на карту без строгих проверок. К моему удивлению, заявка была одобрена, и я смог обновить свой рабочий инструментарий, значительно повысив свою продуктивность и доход.
Если вам нужны деньги на срочные нужды, как это было у меня, и вы не хотите тратить время на банковские проверки, рекомендую воспользоваться ссылкой займ без отказа в новых МФО. Здесь вы найдете список МФО, которые дают займы быстро и без отказа, даже если ваша кредитная история далека от идеала.
Posted by: DzenvaRen | February 24, 2024 9:23 PM
Я работаю фрилансером в области графического дизайна и столкнулся с необходимостью срочно обновить свой графический планшет и монитор для выполнения ряда крупных заказов. Для этого мне требовалось не только высокое качество отображения, но и точность цветопередачи. Поскольку моя кредитная история была не совсем идеальна, я решил обратиться за финансовой помощью к МФО 2024 года, которые известны своей гибкостью и скоростью обработки заявок. Мне нужно было 20 000 рублей, чтобы покрыть стоимость нового оборудования. Благодаря оперативному одобрению займа, я смог приобрести необходимые комплектующие и продолжить свою работу без потери качества и времени.
В ситуациях, когда срочно нужны деньги на ремонт автомобиля или покупку необходимых вещей, и при этом банки отказывают из-за плохой кредитной истории, на помощь придут новые МФО. Рекомендую перейти по ссылке займ без отказа в новых МФО и выбрать оптимальное предложение, которое поможет решить ваши финансовые проблемы без лишних вопросов.
Posted by: Nerzaimope | February 25, 2024 3:56 AM
Breaking news, live coverage, investigations, analysis, video, photos and opinions from The Washington Post.
https://www.washingtonposttoday.com/
Posted by: RobertNaw | February 25, 2024 5:17 PM
prednisone dosage cats how to use prednisone benefits of taking prednisone methylprednisolone compared to prednisone prednisone prescription
Posted by: btaletfzmv | February 26, 2024 5:52 AM
Промышленное оборудование - это ваш ключ к качественному промышленному оборудованию в Казахстане. Мы предлагаем широкий ассортимент товаров от проверенных производителей, гарантируя надежность и эффективность ваших инвестиций. Наша команда стремится обеспечить вас не только отличными товарами, но и высококлассным сервисом, помогая вам достичь успеха в вашем бизнесе. Найдите все необходимое для вашей компании на промбез.kz и доверьтесь опыту и профессионализму лидера в отрасли промышленного оборудования.
Мы стремимся к предоставлению не только качественных товаров, но и высокого уровня сервиса, помогая нашим клиентам оптимизировать связь свои бизнес-процессы и достигать успеха в своих отраслях.
Posted by: VictorDic | February 26, 2024 11:53 AM
Услуги дизайнера mudryakova.ru
Студия Ольги Мудряковой реализует работу уже много лет с дизайнами интерьера жилых и коммерческих пространств. Наша главная задача — не именно внешняя красота, но и предельный комфорт для собственника. Уют помещения не всегда зависит от его масштаба, ведь главное это рассудительный и индивидуальный подход к любому квадратному метру, опираясь на рекомендациях заказчика. На сайте mudryakova.ru Вы сможете узнать подробности.
По теме дизайнер интерьера переходите на данный веб сайт. Осуществляем работу с определенно разными идеями, как под ключ, так и с отдельными небольшими комнатами. Начинаем работу, естественно, со знакомства с вами, ведь каждый интерьер изготовляется под владельца квартиры, всю семью или руководителя отеля. У всех людей личные потребности, задачи помещения, образы жизни и нравы, а также мечты и желания. Мы стараемся учесть все составляющие и сочетать их в оригинальном дизайн проект.
Представленные услуги разнообразны, Вы сможете выбрать их на mudryakova.ru разом или по отдельности. Это создание проекта, сопровождение и контроль строителей на каждых этапах, ремонтные работы, 3D визуализация, составление сметы на материалы, консультация перед покупкой квартир, перепланировка квартиры с документами, интегрирование вентиляционных систем и другие.
Если Вы искали дизайн студия спб в интернете, то Вы на нужном пути. Звоните по номеру телефона +7(812)408-00-07 или пишите на указанном сайте. Студия находится по адресу: г. Санкт-Петербург, ул. Мебельная, д. 49/92. График работы с понедельника по пятницу с 9:00 до 19:00. Наши дизайнеры дадут ответы на любые оставшиеся вопросы, дадут советы по вашему проекту и в быстром времени перейдут к оформлению договора и будущей работе.
Posted by: DesiguBew | February 26, 2024 3:28 PM
official site MetaMask Chrome
Posted by: Jimmykep | February 27, 2024 3:10 AM
Дизайн квартиры под ключ mudryakova.ru
Студия Ольги Мудряковой осуществляет работу уже много лет с интерьерами жилых и коммерческих помещений. Наша основная задача — не только видимая красота, но и максимальное удобство для хозяина. Уют пространства не всегда зависит от его масштаба, ведь основное это мудрый и индивидуальный подход к любому квадратному метру, основываясь на пожеланиях клиента. На сайте mudryakova.ru Вы сможете узнать тонкости.
По теме дизайн интерьера спб заходите на данный онлайн ресурс. Осуществляем работу с совершенно разными идеями, как под ключ, так и с некоторыми небольшими помещениями. Начинаем работу, конечно же, со общения с вами, ведь каждый интерьер выполняется под владельца квартиры, целое семейство или руководителя отеля. У всех людей личные интересы, задачи помещения, образы жизни и нравы, а также мечты и требования. Мы пытаемся учесть все нюансы и соединить их в эксклюзивном дизайн проект.
Представленные услуги различны, Вы можете заказать их на mudryakova.ru все вместе или по отдельности. Это разработка проекта, сопровождение и контроль бригады на всех этапах, демонтаж, 3D визуализация, составление сметы на материалы, консультация перед приобретением квартир, разрешение перепланировки квартиры с документами, интегрирование инженерных систем и другие.
Если Вы хотели найти дизайн студия спб в сети интернет, то Вы на нужном пути. Звоните по телефону +7(812)408-00-07 или пишите на нашем сайте. Студия расположена по адресу: г. Санкт-Петербург, ул. Мебельная, д. 49/92. Время работы с пн по пт с 9:00 до 19:00. Наши консультанты дадут ответы на любые возникающие вопросы, дадут советы по вашему проекту и в быстром времени подойдут к оформлению договора и дальнейшей работе.
Posted by: BukiraBew | February 27, 2024 10:10 AM
restoration of bath enamel
Posted by: JosephDrync | February 27, 2024 12:24 PM
https://clck.ru/36Ew4i
Posted by: RobertHep | February 27, 2024 4:12 PM
crypto mixer - crypto cleaner, bitcoin shuffler
Posted by: Wilfredcoefs | February 27, 2024 11:34 PM
I am final, I am sorry, it not a right answer. Who else, what can prompt?
circumspection
mmfporn.com
@456FgDDY8
Posted by: EugeneFaurb | February 28, 2024 1:03 AM
Hello! I’m new to this business, I recently received crypto coins as a gift, where is the best place for me to exchange them for fiat currency?
Claim airdrop now
Posted by: ThomasRok | February 28, 2024 2:06 AM
1win играть - букмекерская контора 1 вин, 1win войти
Posted by: CarlosQuimb | February 28, 2024 2:13 AM
mixy money - bitcoin mixer, crypto mixer
Posted by: Louisnearf | February 28, 2024 3:40 AM
Hello! I’m new to this business, I recently received crypto coins as a gift, where is the best place for me to exchange them for fiat currency?
Claim airdrop now
Posted by: ThomasRok | February 28, 2024 7:16 AM
lucky jet официальный сайт - сайт lucky jet, lucky jet игра
Posted by: TimothyRef | February 28, 2024 8:36 AM
кракен маркет - кракен даркнет площадка, кракен маркет
Posted by: DonaldSix | February 28, 2024 8:49 AM
win лаки джет - lucky jet hack скачать, lucky jet online
Posted by: VictorSmive | February 28, 2024 9:49 AM
best bitcoin mixer - legitimate bitcoin mixer, reliable bitcoin mixer
Posted by: Robertraism | February 28, 2024 7:42 PM
brillx casino официальный мобильная версия
бриллкс
Вас ждет огромный выбор игровых аппаратов, способных удовлетворить даже самых изысканных игроков. Брилкс Казино знает, как удивить вас каждым спином. Насладитесь блеском и сиянием наших игр, ведь каждый слот — это как бриллиант, который только ждет своего обладателя. Неважно, играете ли вы ради веселья или стремитесь поймать удачу за хвост и выиграть крупный куш, Brillx сделает все возможное, чтобы удовлетворить ваши азартные желания.Играя в Brillx Казино, вы окунетесь в мир невероятных возможностей. Наши игровые автоматы не только приносят удовольствие, но и дарят шанс выиграть крупные денежные призы. Ведь настоящий азарт - это когда каждое вращение может изменить вашу жизнь!
Posted by: Rodneyfluer | February 28, 2024 11:57 PM
Hello, World!
Hey there, mate! Greetings from your favorite surfing capybara!
How’s the surf today?
Ready to catch some gnarly waves together?
https://capybara888.wordpress.com/
Good luck!
Posted by: Capy8Skals | February 29, 2024 1:52 PM
https://clck.ru/36EviP
Posted by: LucilleGax | February 29, 2024 4:17 PM
Hello, World!
Hey there, mate! Greetings from your favorite surfing capybara!
How’s the surf today?
Ready to catch some gnarly waves together?
https://capybara999.wordpress.com/
Good luck!
Posted by: Bettycah | March 1, 2024 6:55 PM
Text Inmate
https://www.contactmeasap.com/
Posted by: GeorgeKep | March 1, 2024 11:55 PM
Professional carpet cleaners in Phoenix that deliver on their promises. Exceptional service!
Carpet Cleaning service in Phoenix 85021!
Posted by: BeckettWeara | March 2, 2024 12:19 AM
Hello from Sporthappy.
Posted by: LarryCok | March 2, 2024 12:43 AM
interesting for a very long time
Posted by: Esbewet | March 2, 2024 3:54 AM
Get ready for unbeatable savings with Zesc Analytics' shoe clearance promotions! We're not just offering discounts – we're leading the pack with our exclusive deals on clearance footwear. Say goodbye to high prices and hello to incredible savings on your favorite brands.
At economical footwear options we're proud to be the leaders in shoe clearance promotions. Our real-time price tracking feature ensures you're always in the loop with the latest markdowns and promotions from top retailers. With instant notifications delivered straight to your device, you'll never miss out on a deal again.
But that's not all – we go above and beyond to keep you ahead of the curve. Get exclusive insights into new arrivals, limited editions, and special collections from renowned brands. With Zesc Analytics, you'll be the trendsetter everyone else follows.
Join the Zesc Analytics community today and experience the difference of shopping with the leaders. With our shoe clearance promotions, you'll step into savings and style like never before. Don't settle for less – choose the best. Start shopping now!
Posted by: EnochUnefs | March 2, 2024 4:17 PM
Latest US news, world news, sports, business, opinion, analysis and reviews from the Guardian, the world's leading liberal voice.
https://www.guardiannewstoday.com
Posted by: Richardbug | March 2, 2024 5:07 PM
Revamp your everyday style with discounted casual shoes from Zesc Analytics! Explore our curated selection of laid-back footwear at unbeatable prices. Bid farewell to hefty price tags and embrace incredible savings with our cutting-edge analytics and price monitoring services.
At low-price footwear we're dedicated to helping you find the perfect pair of casual shoes without breaking the bank. Our real-time price tracking feature ensures you're always in the loop with the latest discounts and promotions from top retailers. Receive instant notifications on your phone or email whenever prices drop, so you can seize the opportunity to snag your favorite casual shoes at the best possible price.
But wait, there's more! Stay ahead of the fashion curve with exclusive insights into new arrivals, limited editions, and special collections from renowned brands. Our platform offers exclusive notifications, keeping you informed about the latest trends and must-have styles in casual footwear.
Join the Zesc Analytics community today and elevate your everyday look while saving money. With our platform, finding discounted casual shoes has never been more convenient. Don't miss out on the chance to add stylish flair to your wardrobe without breaking the bank. Start shopping now!
Posted by: ErofadeUnefs | March 2, 2024 10:24 PM
Магазин запчастей Вольво предлагает запчасти VOLVO новые и с разборок в наличии на складе в Москве и под заказ.
Заходите: запчасти Volvo в Москве.
Posted by: VolvoDuh | March 3, 2024 12:25 AM
latest nfl news espn football live score ed pills canada instagram story viewer order changed email
Posted by: Georgeemity | March 3, 2024 3:00 AM
Discover unbeatable low-price deals on shoes at Zesc Analytics! Dive into our curated selection of footwear where affordability meets style. Say goodbye to high costs and hello to incredible savings with our advanced analytics and price monitoring services.
At special offers on shoes we're dedicated to helping you find the perfect pair of shoes without breaking the bank. Our real-time price tracking feature ensures you're always in the know about the latest low-price deals and promotions from top retailers. Receive instant notifications on your phone or email whenever prices drop, so you can seize the opportunity to snag your favorite shoes at the best possible price.
But that's not all - stay ahead of the fashion curve with exclusive insights into new arrivals, limited editions, and special collections from renowned brands. Our platform offers exclusive notifications, keeping you informed about the latest trends and must-have styles in footwear.
Join the Zesc Analytics community today and step into savings while staying stylish. With our platform, finding low-price deals on shoes has never been easier. Don't miss out on the chance to elevate your shoe game without breaking the bank. Start shopping now!
Posted by: DikasoUnefs | March 3, 2024 5:47 AM
Hello everyone... https://bossbonus.ru/
Posted by: ROSFab | March 3, 2024 9:36 AM
Hey everyone! I've just stumbled upon an amazing website that's all about cryptocurrency exchanges. If you're looking to dive deeper into the world of crypto, this might be the right resource for you!
The site (https://cryptoairdrops.ru/) offers in-depth analysis of a wide range of crypto exchanges, including the ins and outs of their trading platforms, security protocols, supported coins, and overall reliability. Whether you're a beginner just starting out or an experienced trader, there's something for everyone.
What I found particularly useful was their comparison tool, which made it super easy to compare different exchanges and find the one that best fits my needs. They also cover the latest trends in the crypto world, which keeps you informed on all the important news.
If you're curious about exploring different cryptocurrency exchanges, I highly recommend checking this site out. It's a goldmine of information that can help you make educated moves in the ever-changing world of cryptocurrency.
Let's make the most of this resource and help each other out! Would love to hear your thoughts and experiences with different exchanges as well.
Posted by: RobertoPlekS | March 3, 2024 1:12 PM
Read the latest headlines, news stories, and opinion from Politics, Entertainment, Life, Perspectives, and more.
https://www.huffingtonposttoday.com
Posted by: Randallhax | March 3, 2024 1:52 PM
nothing special
Posted by: Stevennus | March 3, 2024 9:18 PM
hydroxychlor tab 200mg How can I get medication for hair loss without a doctor's visit.
Posted by: Monrego | March 3, 2024 9:41 PM
click for more info https://blendedlearning.bharatskills.gov.in/mod/forum/discuss.php?d=1900&parent=2281&lang=gu
Posted by: Donaldfrafe | March 3, 2024 10:57 PM
child porno
==> url.epoch.tw/K9M9j
==> s.yjm.pl/3Eea
child porno
Posted by: Henryskara | March 4, 2024 12:02 PM
top 5 best dating sites
the best dating sites in Belarus rating
Posted by: Stephenhelve | March 4, 2024 4:42 PM
We bring you latest Gambling News, Casino Bonuses and offers from Top Operators, Online Casino Slots Tips, Sports Betting Tips, odds etc.
https://www.jackpotbetonline.com/
Posted by: Richardfleep | March 4, 2024 8:08 PM
https://metamask-extension-4.gitbook.io/web3-browser-us/ MetaMask wallet
Posted by: MetaMask-wallet | March 5, 2024 8:54 AM
смотреть здесь Сливы Mellstroy гаврилина
Posted by: LelandAmogy | March 5, 2024 11:16 AM
Forbes News Today is a global media company, focusing on business, investing, technology, entrepreneurship, leadership, and lifestyle
https://www.forbesnewstoday.com/
Posted by: Raymondwaife | March 5, 2024 11:52 AM
Hello dear friend, I would like to offer placement of your link (or links) on different platforms of the internet such as: forums, blogs, comments and much more. . .
Increase your Visibility Boost Your Seo Rank - Get Organic Traffic From Google. Ranking in Google isn’t hard. All you need is a healthy number of backlinks from referring domains that have authority and trust in Google’s eyes.
This Backlinks Service Benefits:
1. Easily get Google rankings
2. Get a lot of traffic from Google
3. You can earn from the website in different ways
4. Increase Domain Authority (DA)
Quality guaranteed !
PRICE - 20$
WebSite - https://goo.su/ZUHZ
Posted by: Jamesesoca | March 6, 2024 2:56 AM
moved here Buy YouTube Views
Posted by: AlfredSpile | March 6, 2024 2:58 AM
тагриссо продать - тагриссо продать, продать tagriso
Posted by: JustinKnoli | March 6, 2024 6:16 AM
Nova ltd - Nova ltd, нова маркетплейс
Posted by: Earnesthit | March 6, 2024 7:34 AM
как зайти на mega - m3ga ссылка, площадка mega
Posted by: Davidtam | March 6, 2024 12:53 PM
Get the latest news, breaking news, sports, entertainment, weather and traffic, as well as national and international news, from the postgazettenewstoday
https://www.postgazettenewstoday.com/
Posted by: Jeremyusawl | March 6, 2024 3:55 PM
mounjaro тирзепатид купить - уколы саксенда цена, mounjaro
Posted by: Robertdaulk | March 7, 2024 1:05 AM
Germay Latest Breaking News, Pictures, Videos, and Special Reports from Germay News Today. Germay Blogs, Comments and Archive News.
https://www.germaynewstoday.com/
Posted by: DonaldAsype | March 7, 2024 2:54 PM
kraken darknet - кракен, kraken зеркало
Posted by: Ronaldwen | March 7, 2024 4:14 PM
m3ga точка gl - mega sb даркнет, https m3ga gl
Posted by: Donalddof | March 7, 2024 6:51 PM
как зайти на сайт мега - ссылка на мегу через тор, мега площадка
Posted by: Davidoxync | March 7, 2024 9:13 PM
Read latest breaking news, updates, and headlines. National Post offers information on latest national and international events & more.
https://www.nationalposttoday.com/
Posted by: Michaelvox | March 7, 2024 10:14 PM
Магазин запчастей Вольво предлагает запчасти Volvo оригинальные и неоригинальные в наличии на складе в Москве и под заказ.
Заходите: zapchastivolvo.ru в Москве.
Posted by: VolvoDuh | March 8, 2024 12:05 AM
mega актуальная ссылка - mega актуальная ссылка, мега новая ссылка
Posted by: Kevinpax | March 8, 2024 2:24 AM
kraken - kraken ссылка tor, kraken зеркала
Posted by: RodneyEtera | March 8, 2024 4:02 AM
Actually. You will not prompt to me, where I can find more information on this question?
guardianship
mmfporn.com/tags/frot-sex/
@456FgDDY8
Posted by: EugeneFaurb | March 8, 2024 5:47 AM
What good words
care
mmfporn.com/tags/japanese-tits/
@456FgDDY8
Posted by: EugeneFaurb | March 8, 2024 10:25 AM
Планируете обновление интерьера или строительство дома? Подумайте о кованых перилах! Наши кованые перила лестниц для дома цена сочетают в себе красоту ручной работы, непревзойденное качество и доступность. Эти изделия не только украсят ваш дом, но и обеспечат безопасность для вас и ваших близких. Заказывайте сейчас, чтобы ваш дом стал ярким отражением вашего вкуса.
Posted by: GerfariBah | March 8, 2024 6:38 PM
Попробовав новую службу заказа такси и трансфера, я остался приятно удивлен ее эффективностью работы и удобством. Мобильное приложение позволяет скоро и без труда вызвать автомобиль, указав аккуратное местоположение и время доставки. Я заказывал такси по маршруту http://kubtaxi.ru/transfer/taxi-simferopol-kurortnoe
. Водители прибывают вовремя, а степень автомобилей оставляет позитивное впечатление. Кроме того, механизм оценок и комментариев о водителях помогает выбирать наиболее доверенных исполнителей. Я также оценил комфорт оплаты через приложение, что делает процедуру заказа и расчета максимально простым и безопасным. В целом, свежая служба заказа такси и трансфера обосновала мои ожидания и стала неотъемлемым помощником в моих перемещениях по городу.
Posted by: kubMn | March 9, 2024 7:05 PM
memory essay introduction great expectations critical essays why is writing important essay rich dad poor dad book report essay examples of cause and effect essay
Posted by: btaletykwe | March 10, 2024 6:02 AM
Заслуги Давыдова и его профессиональные достижения в области химической промышленности не остались незамеченными Давыдов Эдуард
Posted by: ThomasRon | March 11, 2024 12:40 AM
Обучение Вячеслава Константиновича Николаева началось в стенах МГУ на факультете вычислительной математики и кибернетики, после чего он продолжил образование в США, изучая менеджмент Вячеслав Константинович Николаев
Posted by: RobertHeino | March 11, 2024 12:49 AM
most successful side hustles
Posted by: Jamesfaump | March 11, 2024 4:39 AM
В мире, насыщенном технологиями, нередко возникают ситуации, когда нам требуется найти информацию о владельце определенного номера телефона или узнать его местоположение. Это может быть из-за того, что мы получили звонок или сообщение от незнакомого номера, или же нам просто нужно связаться с определенным человеком, но мы не знаем, как это сделать как найти по номеру телефона
Posted by: GeorgeExoms | March 11, 2024 9:45 AM
Была задача полностью реконструировать кровлю с заменой покрытия на МЧ и утеплением. Работа идет с соблюдением технологии монтажа, видно что люди опытные https://andymyeh93693.tinyblogging.com/house-painting-minsk-69252574
Posted by: BennyWes | March 11, 2024 11:26 AM
С 1999 года Николаев активно участвовал в международных проектах, начиная с аналитической работы в области телекоммуникаций и ИТ в J’son and Partners Николаев Вячеслав Константинович
Posted by: Jeremysex | March 11, 2024 2:08 PM
Удобное расположение автошколы у метро позволит Вам совмещать занятия с работой или учёбой в ВУЗе автошкола тушинская
Posted by: RandyLig | March 11, 2024 3:47 PM
Филиал автошколы рядом с метро Борисово в Москве - профессиональное обучение на автомобилях с МКПП и АКПП получение водительских прав категории B с оплатой в рассрочку без переплат и скрытых платежей автошкола в строгино
Posted by: Jeremysex | March 12, 2024 2:00 AM
He began his studies in Kezdivasarhely and continued in Nagyenyed , from where he went to foreign universities in Jena and Gottingen https://konyvtar.mta.hu/index.php?name=h_3_1_2009
Posted by: Tylertoisk | March 12, 2024 3:07 AM
https://clck.ru/36EvrK
Posted by: IrinaPriow | March 12, 2024 5:44 AM
https://www.ibm.com
Posted by: BrianNeurb | March 12, 2024 6:37 AM
Только сейчас в PUMA Moldova уникальное предложение: скидки до 30% на весь ассортимент! От футболок до кед – у нас есть все для вашего спортивного и повседневного стиля. Покупайте качество от мирового бренда и пользуйтесь бесплатной доставкой по Кишиневу. Заходите на наш сайт и выбирайте идеальные товары для себя и ваших близких по выгодным ценам.
Pumamoldova.md - кроссовки кишинев
Контакты и адрес - Молдова, г. Кишинев
Posted by: Bredmolka | March 12, 2024 7:32 AM
Free download Pinterest SVG Icons for logos, websites and mobile apps, useable in Sketch or Figma android icons
Posted by: Patrickcip | March 12, 2024 9:45 AM
Latest Business News headlines, LIVE share market news and updates, financial, economic and banking news from across the World
https://www.livemintnewstoday.com/
Posted by: Williamkiz | March 12, 2024 10:32 AM
buy generic lipitor buy atorvastatin generic purchase atorvastatin generic
Posted by: Cjuiad | March 12, 2024 12:08 PM
atorvastatin 40mg oral oral lipitor 80mg order atorvastatin 80mg pill
Posted by: Qlovgu | March 12, 2024 12:19 PM
order lipitor 10mg pill order generic lipitor lipitor 20mg brand
Posted by: Xlofvd | March 12, 2024 12:29 PM
buy lipitor tablets buy cheap generic lipitor purchase atorvastatin sale
Posted by: Mfwklp | March 12, 2024 12:32 PM
Вас приветствует интернет-магазин PUMA Moldova! Ищете стильные и удобные кроссовки? У нас огромный выбор кроссовок PUMA для мужчин и женщин со скидками! Не пропустите уникальную возможность обновить свой спортивный гардероб высококачественной обувью по привлекательным ценам. Помимо кроссовок, у нас вы найдете широкий ассортимент одежды и аксессуаров для всей семьи. Действует бесплатная доставка по Кишиневу. Выбирайте лучшее в PUMA Moldova и наслаждайтесь комфортом и стилем каждый день!
Pumamoldova.md - купить майки
Контакты и адрес - Молдова, г. Кишинев
Posted by: Anthonymoono | March 12, 2024 12:39 PM
buy atorvastatin pills lipitor 40mg cheap lipitor 80mg uk
Posted by: Hnxazv | March 12, 2024 1:02 PM
buy atorvastatin online cheap order atorvastatin 80mg generic lipitor 20mg pill
Posted by: Bfadwb | March 12, 2024 1:03 PM
This online casino is an online betting platform operated by A Company Limited. With its central hub in Valletta, Malta, this casino aims to cater to a worldwide player base, targeting players from India. Yet, it is important to note that betglobal Casino restricts access to players from the United States.
One of the most important aspects of any online casino is the variety and quality of casino games it offers. betglobal Casino displays an outstanding list of game providers, including trusted names such as Pragmatic Play, Play'n Go, Betsoft, NetEnt, Microgaming, Evolution, and many others. With such a diverse range of providers, players can expect a wide selection of top-tier games to choose from. Whether you're a fan of live casino experiences, betglobal Casino has everything you need.
In terms of slots, betglobal Casino offers over 1,000 games, making sure there is a game for all players' preference. From classic slots to modern video slots with exciting features and themes, the casino provides a diverse and varied slot gaming journey. Additionally, betglobal Casino also offers a live casino option for players seeking an immersive and interactive gambling journey. With live dealers and real-time gameplay, players can enjoy the thrill of a land-based casino from the ease of their own homes.
When it comes to accessibility, betglobal Casino offers an instant play version, allowing players to access their favorite games right from their web internet browsers. However, the casino does not provide a installable version of its software. Nevertheless, players can enjoy the casino's selections on various operating systems, as betglobal Casino is functional with all major platforms.
Language support is another area where betglobal Casino excels, catering to a varied player base. The casino supports multiple languages, including English, Spanish (LATAM), Portuguese (BR), French (CA), and German. This multilingual support ensures that players from various countries can navigate the platform effortlessly and experience a smooth gaming adventure.
In terms of currencies, betglobal Casino accepts USD, EUR, CAD, and BRL, providing flexibility for players to deposit and bet in their favorite currency. However, it is worthwhile noting that betglobal Casino does not permit players from the United States, meaning that USD deposits and gameplay are not apposite.
betglobal Casino preserves a high level of security and carries a license from Curacao. This ensures that the casino works within the legal framework and sticks to industry standards. Players can relish a safe and fair gaming environment while playing at betglobal Casino.
When it comes to deposits and withdrawals, betglobal Casino provides a selection of payment methods. However, specific details regarding the minimum deposit, withdrawal methods, withdrawal limits, and withdrawal times were not provided in the present data. It is suggested to refer to the casino's website or contact customer support for current information regarding these aspects.
In terms of customer support, betglobal Casino offers both email support and live chat feature. Players can get in touch with the support team via email at support@betglobal.com or take advantage of the live chat feature for prompt assistance. While a support phone number is not given, the mix of email and live chat support ensures that players can effortlessly communicate their inquiries or concerns to the casino's support team.
Offers and bonuses are an essential part of the online gambling experience, and betglobal Casino does not let down in this aspect. The casino offers a considerable welcome bonus for its players. The specifics of the welcome bonus change based on the player's location. For players in LATAM, the welcome bonus consists of a 120% deposit match up to $600 and 25 free spins. Brazilian players can experience a similar 120% deposit match up to R$4k and 25 free spins. Canadian players are entitled for a 120% deposit match up to $600 and 25 free spins. For players in other regions, the welcome bonus is a 120% deposit match up to 600 EUR and 25 free spins.
betglobal Casino provides openness by sharing the terms and conditions of its welcome bonus. Players are urged to review these terms to grasp the wagering requirements and any other constraints associated with the bonus. The terms and conditions for the welcome bonus can be found on the casino's website.
In addition to the welcome bonus, betglobal Casino offers offers to keep players involved and rewarded. The details of these promotions can be found on the casino's special promotions page, which is frequently updated to offer new and enticing incentives for players.
betglobal Casino also runs an affiliate program called betglobal Partners. This program allows individuals or businesses to earn commissions by referring players to the casino. The affiliate program has its own dedicated website, where curious parties can find more information and sign up.
In conclusion, betglobal Casino keeps a payout percentage of 95%, which indicates that players have a fair chance of winning while playing on the platform. This payout percentage is meets industry standards and guarantees that players can have a gratifying and potentially lucrative gaming experience.
Overall, betglobal Casino offers an extensive range of games from reliable providers, offering players with a broad and engaging gambling experience. The multi-language support, diverse currency options, and attractive welcome bonuses even more boost the total attraction of the casino. With its devotion to player safety and fair gaming, betglobal Casino stands as a prospective online gambling destination.
Note: The information provided in this review is based on available data as of the time of writing. For the most recent information and offerings, it is suggested to visit the betglobal Casino website.
Posted by: betGlobal | March 12, 2024 1:29 PM
lipitor 80mg oral purchase atorvastatin generic buy lipitor 10mg pills
Posted by: Teyarx | March 12, 2024 1:47 PM
generic atorvastatin 20mg order atorvastatin 10mg without prescription order atorvastatin pill
Posted by: Ftqhps | March 12, 2024 1:54 PM
Где купить виртуальный номер: рейтинг лучших сервисов SMS-активаций. Как и в других подобных сервисах, здесь вы можете купить номер для разового использования или взять номер в аренду на более длительный срок арендовать номер телефона
Posted by: RubenMib | March 12, 2024 11:35 PM
Hеllоǃ
Ι аpologіze for thе оverly ѕpecific mеssage.
Му gіrlfriend and Ι lоvе each оthеr. Αnd wе are all great.
Βut... wе nееd a man.
We аrе 25 yеars оld, from Romаnіa, wе also know english.
Wе nеver get boredǃ Αnd nоt only іn tаlk...
My nаme is Lіnа, mу рrоfіlе іs hеre: https://1pt.co/6x5a8
Posted by: LinaSr | March 13, 2024 1:28 AM
This online casino is an online betting platform owned by A Company Limited. With its headquarters in Malta's capital, Valletta, betglobal aims to cater to a worldwide audience, targeting players from India. However, it is crucial to note that betglobal Casino prohibits players from the United Kingdom.
One of the most crucial aspects of any online casino is the variety and quality of casino games it offers. betglobal Casino boasts an remarkable list of game providers, including trusted names such as Pragmatic Play, Play'n Go, Betsoft, NetEnt, Microgaming, Evolution, and many others. With such a wide range of providers, players can anticipate a wide selection of premium games to pick from. Whether you are a fan of live casino experiences, betglobal Casino has everything you covered.
In terms of slots, betglobal Casino offers over 1,000 games, guaranteeing there is something for each player's preference. From classic slots to modern video slots with engaging features and themes, the casino provides a diverse and varied slot gaming adventure. Additionally, betglobal Casino also offers a live casino option for players looking for an immersive and interactive gambling journey. With live dealers and real-time gameplay, players can enjoy the thrill of a physical casino from the ease of their own houses.
When it comes to accessibility, betglobal Casino offers an instant play version, permitting players to access their favorite games straight from their web browsers. But, the casino does not provide a downloadable version of its software. Nonetheless, players can relish the casino's games on different operating systems, as betglobal Casino is functional with all major platforms.
Support for multiple languages is another area where betglobal Casino stands out, providing to a diverse player base. The casino supports various languages, including English, Spanish (LATAM), Portuguese (BR), French (CA), and German. This multi-language support guarantees that players from different countries can navigate the platform easily and experience a smooth gaming journey.
In terms of money, betglobal Casino accepts USD, EUR, CAD, and BRL, providing flexibility for players to deposit and bet in their preferred currency. Nevertheless, it is worthwhile noting that betglobal Casino does not permit players from the United States, suggesting that USD deposits and gameplay are not apposite.
betglobal Casino upholds a high level of security and carries a license from Curacao. This ensures that the casino operates within the lawful framework and sticks to industry standards. Players can relish a safe and fair gaming ambiance while playing at betglobal Casino.
When it comes to deposits and withdrawals, betglobal Casino provides a variety of payment methods. Yet, specific details regarding the minimum deposit, withdrawal methods, withdrawal limits, and withdrawal times were not provided in the present data. It is recommended to refer to the casino's website or contact customer support for current information regarding these aspects.
In terms of customer support, betglobal Casino offers both email support and live chat functionality. Players can get in touch with the support team via email at support@betglobal.com or utilize the live chat feature for instant assistance. While a support phone number is not provided, the mix of email and live chat support ensures that players can effortlessly transmit their inquiries or concerns to the casino's support team.
Offers and bonuses are an integral part of the online gambling experience, and betglobal Casino does not let down in this aspect. The casino offers a sizeable welcome bonus for its players. The specifics of the welcome bonus change based on the player's location. For players in LATAM, the welcome bonus consists of a 120% deposit match up to $600 and 25 free spins. Brazilian players can benefit from a similar 120% deposit match up to R$4k and 25 free spins. Canadian players are qualified for a 120% deposit match up to $600 and 25 free spins. For players in other regions, the welcome bonus is a 120% deposit match up to 600 EUR and 25 free spins.
betglobal Casino provides transparency by sharing the terms and conditions of its welcome bonus. Players are encouraged to review these terms to understand the wagering requirements and any other limitations associated with the bonus. The terms and conditions for the welcome bonus can be found on the casino's website.
In addition with the welcome bonus, betglobal Casino offers offers to keep players interested and rewarded. The details of these promotions can be found on the casino's special promotions page, which is regularly updated to offer new and exciting incentives for players.
betglobal Casino also manages an affiliate program called betglobal Partners. This program allows individuals or businesses to earn commissions by guiding players to the casino. The affiliate program has its own dedicated website, where curious parties can find more information and sign up.
Finally, betglobal Casino maintains a payout percentage of 95%, which suggests that players have a decent chance of winning while playing on the platform. This payout percentage is meets industry standards and confirms that players can enjoy a rewarding and potentially lucrative gaming experience.
Overall, betglobal Casino offers an extensive range of games from reliable providers, providing players with a broad and engaging gambling adventure. The multi-language support, diverse currency options, and attractive welcome bonuses further increase the complete attraction of the casino. With its commitment to player safety and fair gaming, betglobal Casino stands as a prospective online gambling destination.
Note: The information given in this review is based on available data as of the time of writing. For the most up-to-date information and offerings, it is suggested to visit the betglobal Casino website.
Posted by: betGlobal | March 13, 2024 8:13 AM
То есть, виртуальный номер нужен для обеспечения приватности, безопасности и свободной работы с десятками и сотнями сайтов одновременно арендовать номер телефона
Posted by: Forrestrib | March 13, 2024 1:32 PM
order baycip online cheap - septra ca buy augmentin generic
Posted by: Viempd | March 14, 2024 1:12 AM
Покупайте вилочные погрузчики в каталоге можете выбрать и купить электропогрузчики и автопогрузчики (бензиновые или дизельные) с различными параметрами и характеристиками https://shantui.kg/
Posted by: Randallnex | March 14, 2024 6:33 AM
drug education policy https://institutoferreyra.org/lores.html dog toothache remedy
Posted by: AllenBrete | March 14, 2024 10:14 AM
restoration of bath enamel
Posted by: JosephDrync | March 14, 2024 10:49 AM
ciprofloxacin 1000mg pills - buy generic trimethoprim over the counter buy generic augmentin 1000mg
Posted by: Rpgkge | March 14, 2024 4:40 PM
Hello everyone... https://t.me/bonus7casino/9
Posted by: BOSFab | March 14, 2024 6:36 PM
https://avto-dublikat.ru/
Posted by: Geraldreurf | March 14, 2024 10:37 PM
Good afternoon https://azino777-bonus23.ru
Posted by: BOSFab | March 15, 2024 4:55 AM
Locked out of your Instagram account and feeling a bit lost? No worries – Antiban Pro is here to help you retrieve your locked IG account and get you back in the loop! We understand how frustrating it can be, but fear not – we've got the tools to help you unlock your profile in a jiffy.
Here's the deal: our platform provides easy-to-follow steps to retrieve your locked IG account. Whether it's a glitch in the system or a security measure, we'll guide you through the process so you can regain access to your profile in no time.
Why choose Antiban Pro? Because we're all about giving you the freedom to connect with your friends and followers without any obstacles. Our team is committed to offering friendly support and practical solutions to help you unlock your IG account securely.
So, don't sweat it – let Antiban Pro help you retrieve your locked IG account and get back to sharing those epic moments with your followers. Your next post is just around the corner!
Antiban.pro - instagram support for locked accounts
Posted by: MalopgeZer | March 15, 2024 6:25 AM
Ремонт квартиры или постройка дома своими руками
Источник: https://stroj-sam.ru
Posted by: qpxmbtrz | March 15, 2024 7:29 AM
Hello everyone... https://t.me/s/brazino777bonus/7
Posted by: BOSFab | March 15, 2024 8:01 AM
Feeling overwhelmed by the constant stream of updates and notifications on Facebook? Antiban Pro offers a solution to give yourself a break – temporarily deactivate your Facebook account. Our platform makes it easy to step back from the social media noise and take some time for yourself.
Whether you need a short hiatus or want to reassess your digital habits, Antiban Pro provides a simple way to deactivate your Facebook account temporarily. Your profile will be hidden from others, and you can return whenever you're ready, with all your data intact.
Why choose Antiban Pro? We prioritize user well-being and offer solutions to help you maintain a healthy relationship with social media. Take control of your digital well-being and enjoy a break from the pressures of constant connectivity with Antiban Pro.
Ready to take a step back from Facebook? Trust Antiban Pro to help you temporarily deactivate your account and recharge. Prioritize your mental health and enjoy a digital detox with Antiban Pro today!
Antiban.pro - instagram account suspended
Posted by: LainstaZer | March 15, 2024 9:38 AM
Hello everyone... https://t.me/brazino777bonus/6
Posted by: BOSFab | March 15, 2024 10:47 AM
Encountering a hacked Instagram account can be a distressing experience, but there are resources available to assist you in regaining control and restoring access swiftly. When faced with the challenge of recovering a hacked Instagram account, it's essential to leverage valuable resources to navigate through the process effectively.
Antiban Pro Guides: Antiban Pro offers comprehensive guides and resources designed to assist users in recovering hacked Instagram accounts. These guides provide step-by-step instructions, practical tips, and expert advice to empower users to take decisive action and regain control of their accounts with confidence.
Instagram Help Center: Instagram's Help Center is a valuable resource for users experiencing issues with their accounts, including hacking incidents. The Help Center provides information on account security, reporting hacking incidents, and steps to take to recover hacked accounts.
Online Forums and Communities: Online forums and communities dedicated to Instagram users can be valuable sources of support and guidance when recovering hacked accounts. These communities provide a platform for users to share their experiences, seek advice from others who have gone through similar situations, and access additional resources for account recovery.
Cybersecurity Websites and Blogs: Cybersecurity websites and blogs often publish articles and guides on account security and recovery strategies. These resources offer insights into common hacking tactics, proactive measures to enhance account security, and steps to take when recovering hacked accounts.
Antiban.pro - facebook account deactivated
Posted by: AntirakeZer | March 15, 2024 3:42 PM
Good day https://t.me/brazino777bonus/8
Posted by: BOSFab | March 15, 2024 5:30 PM
продолжить электрик томск
Posted by: DanielTed | March 15, 2024 11:55 PM
Официальный сайт в Тор браузере, лучший выбор для тех, кто ищет безопасный и анонимный доступ на Мега даркнет. Рабочая ссылка на Мега Даркнет на нашем сайте mega как попасть
Posted by: RonaldNem | March 16, 2024 1:23 AM
dapoxetine priligy How do I verify the legitimacy of online pharmacies that offer "miracle cures" or unproven treatments.
Posted by: Monrego | March 16, 2024 2:00 AM
Feeling frustrated by a blocked Instagram account? Antiban Pro has the solution you need to regain access and reconnect with your online community. Our platform offers a straightforward approach to unblocking Instagram accounts, putting you back in control of your digital presence.
Whether your account was mistakenly blocked or restricted due to suspicious activity, Antiban Pro can help you navigate through the process of unlocking it. Our team of experts is dedicated to providing efficient and reliable solutions, ensuring that you can resume using Instagram without any interruptions.
Why choose Antiban Pro? We understand the importance of staying connected in today's digital age. With our assistance, you can quickly overcome any obstacles preventing you from accessing your Instagram account and continue sharing moments with your followers.
Don't let a blocked Instagram account hinder your online experience. Trust Antiban Pro to help you unlock your account and get back to enjoying everything that Instagram has to offer. Take the first step towards regaining control of your social media presence today!
Antiban.pro - dealing with locked instagram account
Posted by: FiconeZer | March 16, 2024 5:57 AM
опубликовано здесь электрик томск
Posted by: Carltonfed | March 16, 2024 6:22 AM
You can Purchase the card by the Official Apple Website, Paypal and Ebay or from the assured online web stores where can i buy itunes gift cards
Posted by: Jasonanast | March 16, 2024 9:42 AM
онлайн казино с депозитом
https://remvend-cafe.ru/casino/casino-med-visa.html
Posted by: Louisediali | March 16, 2024 9:44 AM
Больше информации об услуге дизайн-проект ванной комнаты. Если остались вопросы или есть желание заказать дизайн проект коттеджа, с радостью приглашаем на бесплатную консультацию в нашу студию онлайн проектування будинку
Posted by: Donaldutecy | March 16, 2024 12:07 PM
Mega – облачное хранилище файлов из Новой Зеландии. Стало известно благодаря своему основателю, бизнесмену Киму Доткому, владельцу известного файлообменника Megaupload мега площадка
Posted by: RobertGoota | March 16, 2024 3:22 PM
more helpful hints roblox executor
Posted by: Allencog | March 17, 2024 1:17 AM
More about the author Sound and Video Editor
Posted by: TimothyErono | March 17, 2024 4:04 AM
ciprofloxacin for sale online - buy ciplox 500mg sale where to buy erythromycin without a prescription
Posted by: Pvvslp | March 17, 2024 4:57 AM
mega555kf7lsmb54yd6etzginolhxxi4ytdoma2rf77ngq55fhfcnyid onion - mega ссылка, мега даркнет
Posted by: Josephreups | March 17, 2024 6:00 AM
Предприятие является участником экспортного форсажа, определило два рынка для вывода всех первичных продуктов. Поставляет продукцию в Узбекистан, Киргизию, Белоруссию и Казахстан завод весового оборудования
Posted by: Williamfor | March 17, 2024 9:36 AM
купить аккаунт вк ретрив - купить аккаунт твиттер подтвержденный, купить аккаунт вк за 1 рубль
Posted by: FreddieGen | March 17, 2024 9:43 AM
metronidazole over the counter - buy cefaclor without prescription buy azithromycin cheap
Posted by: Opwmme | March 17, 2024 12:12 PM
Подробно расскажем, как Расторжение брака - Ленинский районный суд г. Астрахани онлайн или самостоятельно. Юристы по трудовому праву подготовили пошаговую инструкцию по взысканию компенсации за задержку выплаты зарплаты в суде: этапы взыскания, размер пошлины, подсудность и ответы на частые вопросы работников
Posted by: RobertMetry | March 17, 2024 12:24 PM
Premium domain for sale https://im-autoru.ru
Posted by: BOSFab | March 17, 2024 1:36 PM
find keyless executor
Posted by: GeraldVaf | March 17, 2024 5:56 PM
Привет! Недавно столкнулся с тем, что внезапно закончились продукты в холодильнике, а зарплата еще не скоро. В такие моменты действительно спасает портал zaim-fin.ru! Там я нашел множество МФО, готовых выдать займы даже тем, у кого не самая лучшая кредитная история. Без лишних проверок, всё честно и прозрачно. Благодаря этому я смог быстро пополнить запасы продуктов и не переживать из-за пустого холодильника. А ещё на этом сайте много полезной информации о микрозаймах и как правильно оформить заявку на получение кредита. Всегда приятно, когда есть помощь в трудную минуту!
Posted by: ZaifilakeRox | March 17, 2024 5:59 PM
this page toast wallet review
Posted by: Charlesdus | March 17, 2024 6:08 PM
kraken darknet market зеркало - kraken ссылка зеркало официальный, зеркало kraken market
Posted by: Scottjes | March 17, 2024 6:35 PM
Breaking News, Pictures, Videos, and Special Reports from The Star News Today
https://www.thestarnewstoday.com/
Posted by: StevenRucky | March 17, 2024 6:58 PM
I apologise, but, in my opinion, you are not right. I can defend the position.
circumspection
mmfporn.com/tags/thigh-fuck/
@456FgDDY8
Posted by: EugeneFaurb | March 17, 2024 6:58 PM
Get the latest world news from The Daily Mail News Today.
https://www.thedailymailnewstoday.com/
Posted by: Williamdieva | March 18, 2024 12:37 AM
Необходимо воспользоваться зеркалом официального сайта 1вин или скачать официальное приложение 1win. Регистрация на официальном сайте 1вин зеркало
Posted by: EddiedUm | March 18, 2024 1:20 AM
купить семена в калуге интернет магазин семена россии каталог
семена озера почтой интернет магазин каталог семена для посадки https://institute-np-annamatari.ru магазин у беллы профессиональные семена каталог новосибирские семена интернет магазин
интернет магазин семян с бесплатной доставкой по россии почтой
Posted by: DuaneneM | March 18, 2024 3:17 AM
Привет! В преддверии праздников, как это обычно бывает, накопилось множество счетов, которые нужно оплатить. Но вот незадача - финансов не хватает на все расходы. Не приятная ситуация, правда? Но, как всегда, портал zaim-fin.ru пришел на выручку! Там я нашел много МФО, готовых выдать займы даже людям с плохой кредитной историей. Без лишних проверок и ожиданий. Благодаря этому я смог решить свою проблему с оплатой счетов и насладиться предстоящими праздниками без лишних переживаний. А еще на этом сайте есть много полезной информации о микрозаймах и советы по их получению. Очень удобно!
Posted by: BnervoVew | March 18, 2024 7:40 AM
Можно приготовить вкусный ягодный смузи из замороженных ягод и йогурта, с фруктовым соком или молоком татарские блюда
Posted by: JamesBrode | March 18, 2024 10:13 AM
Что действительно следует учитывать, используя наш генератор виртуальных номеров, так это то, что каждый тестовый телефон, который предлагает эмулятор, действительно доступен множеству пользователей на протяжении всего своего периода актуальности аренда номера для смс
Posted by: GregoryCoomi | March 18, 2024 1:48 PM
Привет, друзья! Хотите обновить технику в своем доме, но не знаете, с чего начать? Портал zaim-fin.ru поможет вам решить эту задачу! Здесь вы найдете ряд МФО, готовых предоставить займы для покупки новой бытовой техники. На портале также доступно множество полезных советов о том, как выбрать подходящую модель, какие параметры учитывать при выборе, и как не переплатить за покупку. Благодаря zaim-fin.ru вы сможете легко и удобно обновить свою технику и сделать вашу жизнь в доме еще комфортнее и приятнее!
Posted by: VabizaiVew | March 18, 2024 5:57 PM
Agile software development - Pay-per-click (PPC) advertising, Customer experience management (CEM)
Posted by: Stephenagere | March 18, 2024 10:57 PM
Смотрите сериал Золотая лихорадка. Аляска онлайн Золотая лихорадка смотреть онлайн 1-14 сезон все серии с русским переводом абсолютно бесплатно.
Posted by: Anthonyheets | March 19, 2024 2:44 AM
thanks, interesting read
Posted by: Frbetmax | March 19, 2024 3:52 AM
Найдите свой идеальный вариант недвижимости в Объединенных Арабских Эмиратах недвижимость в оаэ цены - лучший сайт, на котором вы найдете все необходимое!
Posted by: Jeremytaxia | March 19, 2024 8:33 AM
stromectol tablets for humans - buy ciplox without a prescription sumycin 250mg pills
Posted by: Ndmcgr | March 19, 2024 8:49 AM
credit cards dark markets - The Dark Web Links, Emails deep web links
Posted by: BrianTit | March 19, 2024 8:53 AM
buy valacyclovir 1000mg online cheap - starlix 120mg tablet buy zovirax for sale
Posted by: Pcrynu | March 19, 2024 5:30 PM
Slightly off topic :)
It so happened that my sister found an interesting man here, and recently got married ^_^
(Moderator, don't troll!!!)
Is there are handsome people here! ;) I'm Maria, 25 years old.
I work as a model, successfull - I hope you do too! Although, if you are very good in bed, then you are out of the queue!)))
By the way, there was no sex for a long time, it is very difficult to find a decent one...
And no! I am not a prostitute! I prefer harmonious, warm and reliable relationships. I cook deliciously and not only ;) I have a degree in marketing.
My photo:
___
Added
The photo is broken, sorry(((
Check out my blog where you'll find lots of hot information about me:
https://zagrevo.ru
Or write to me in telegram @Lolla_sm1_best ( start chat with your photo!!!)
Posted by: DayanaEvima | March 19, 2024 8:39 PM
промокод 1хбет фриспины - промокод 1xbet на ординар, бонус счастливая пятница 1xbet
Posted by: Gregoryvom | March 19, 2024 10:52 PM
Деньги на карту в течение часа! Досрочное погашение без штрафов! Онлайн одобрение! Без кредитной истории. Без скрытых комиссий. Без справок о доходах деньги под залог авто в воронеже
Posted by: Douglassnigo | March 20, 2024 12:23 AM
Для того, чтобы получить эксклюзивные бонусы от букмекера нужны промокоды, которые не всегда просто можно получить. В этой статье разобрано, как получить и использовать промо коды 1хБет и какие бонусы они дают получить промокод 1xbet
Posted by: HowardEpity | March 20, 2024 11:50 AM
Увеличим Ваши продажи в Etsy. Pinterest + SEO+II https://pint77.com Даю Гарантии Заказчику + Artificial intelligence in action - Clip Art and Digital Image.
Posted by: EvelynNindy | March 20, 2024 1:00 PM
Slightly off topic :)
It so happened that my sister found an interesting man here, and recently got married ^_^
(Moderator, don't troll!!!)
Is there are handsome people here! ;) I'm Maria, 27 years old.
I work as a model, successfull - I hope you do too! Although, if you are very good in bed, then you are out of the queue!)))
By the way, there was no sex for a long time, it is very difficult to find a decent one...
And no! I am not a prostitute! I prefer harmonious, warm and reliable relationships. I cook deliciously and not only ;) I have a degree in marketing.
My photo:
___
Added
The photo is broken, sorry(((
Check out my blog where you'll find lots of hot information about me:
https://kotel-otoplenija.ru/
Or write to me in telegram @Lolla_sm1_best ( start chat with your photo!!!)
Posted by: DayanaEvima | March 20, 2024 1:07 PM
Привет, друзья! Сегодня я хочу поделиться с вами историей о том, как портал zaim-fin.ru стал настоящим спасением для нашего пушистого друга - нашего кота по имени Барсик. Недавно он заболел, и нам срочно понадобились деньги на лечение. Как вы понимаете, в такие моменты стоит обращаться за помощью к лучшим друзьям. И знакомство с zaim-fin.ru оказалось настоящим открытием! Здесь я нашел не только возможность получить займы от различных МФО, но и ценные советы о том, как оформить заявку на микрокредит и какие требования у МФО к заемщику. Благодаря этому ресурсу, мы смогли обеспечить нашего четвероногого друга всем необходимым для его выздоровления. Итак, если вы столкнетесь с ситуацией, где нужна срочная финансовая помощь вашему питомцу, помните о портале zaim-fin.ru - это место, где друзья всегда поддержат!
Posted by: Finamirebaf | March 20, 2024 2:12 PM
Do you need reviews on google, reviews on voting sites, do you need someone to create gmail accounts, do you want to increase the views of your youtube video, or do you need people to perform small tasks on the Internet, and much more you can solve through the site https://aviso.bz/?r=filosof20063
Posted by: Manuelmup | March 20, 2024 5:02 PM
pop over to this web-site lego fortnite skin
Posted by: AngelThing | March 20, 2024 7:45 PM
промокод 1xbet от ютуберов - получить промокод 1хставка, 1xbet купоны
Posted by: PatrickFak | March 20, 2024 8:40 PM
хочу скачать 1xbet - бездепозитный промокод 1хбет, xbet промокод на день рождения
Posted by: Byrongrirm | March 20, 2024 8:49 PM
iStore este magazinul cu produse Apple din Moldova care aduce lumea Apple mai aproape de tine. Aici vei gasi o gama variata de modele de iPhone, MacBook Pro, iMac, iPad si o multime de accesorii originale care sa iti faca experienta cu Apple mult mai placuta si eficienta.
Posted by: MichaelDug | March 20, 2024 9:09 PM
more tips here lego fortnite skin
Posted by: Freddiewak | March 20, 2024 10:36 PM
acillin cheap penicillin online order buy generic amoxil for sale
Posted by: Ownksb | March 21, 2024 10:21 AM
Если вам необходимы пассажирские перевозки на микроавтобусах, вы обратились по нужному адресу. Компания готова предоставить в ваше распоряжение не только целый ряд презентабельных микроавтобусов, но и услуги опытных профессиональных водителей с большим стажем работы Констанца - Кременчуг
Posted by: Granttob | March 21, 2024 10:47 AM
purchase metronidazole generic - cost amoxil zithromax 250mg price
Posted by: Qxdbdc | March 21, 2024 2:39 PM
Полный комплекс бухгалтерских услуг для юридических лиц, ООО и ИП. Ведение бухучета, сопровождение и обслуживание бизнеса. Наши бухгалтеры помогут Вам бухгалтерские услуги
Posted by: Michaelvew | March 21, 2024 11:56 PM
jackpotbetonline.com - We bring you latest Gambling News, Casino Bonuses and offers from Top Operators, Online Casino Slots Tips, Sports Betting Tips, odds etc.
https://www.jackpotbetonline.com/
Posted by: RickyEmisp | March 22, 2024 12:59 AM
В современной промышленности важное значение имеет испытательное оборудование, позволяющее оценивать параметры изделий на различных этапах производства. Один из ключевых видов такого оборудования — вибростенды, предназначенные для испытания компонентов и сборок на устойчивость к воздействию механических колебаний виброустановки
Posted by: ErvinShole | March 22, 2024 5:07 AM
пэп на ваш номер - офисы заливов, ICard
Posted by: MerlinPiz | March 22, 2024 6:06 AM
брилкс казино
бриллкс
Вас ждет огромный выбор игровых аппаратов, способных удовлетворить даже самых изысканных игроков. Брилкс Казино знает, как удивить вас каждым спином. Насладитесь блеском и сиянием наших игр, ведь каждый слот — это как бриллиант, который только ждет своего обладателя. Неважно, играете ли вы ради веселья или стремитесь поймать удачу за хвост и выиграть крупный куш, Brillx сделает все возможное, чтобы удовлетворить ваши азартные желания.Играть онлайн бесплатно в игровые аппараты стало еще проще с нашим интуитивно понятным интерфейсом. Просто выберите свой любимый слот и погрузитесь в мир ярких красок и захватывающих приключений. Наши разнообразные бонусы и акции добавят нотку удивительности к вашей игре. К тому же, для тех, кто желает ощутить настоящий азарт, у нас есть возможность играть на деньги. Это шанс попытать удачу и ощутить адреналин, который ищет настоящий игрок.
Posted by: Rodneyfluer | March 22, 2024 7:51 AM
Hi there,
My name is Mike from Monkey Digital,
Allow me to present to you a lifetime revenue opportunity of 35%
That's right, you can earn 35% of every order made by your affiliate for life.
Simply register with us, generate your affiliate links, and incorporate them on your website, and you are done. It takes only 5 minutes to set up everything, and the payouts are sent each month.
Click here to enroll with us today:
https://www.monkeydigital.org/affiliate-dashboard/
Think about it,
Every website owner requires the use of search engine optimization (SEO) for their website. This endeavor holds significant potential for both parties involved.
Thanks and regards
Mike Haig
Monkey Digital
Posted by: Mike Haig | March 22, 2024 10:52 AM
Если вам надоел пресный секс и давно хочется разнообразить свой интим, самое время воспользоваться услугами проститутки. Для этого достаточно посетить сайт newswomanblog.win, где доступны анкеты самых разных индивидуалок. Кроме классического вагинального секса они предлагают многие другие услуги: анал, ролевые игры, доминирование, окончание в рот, золотой дождь и т.д. Это целый калейдоскоп удовольствий. Выбирайте понравившуюся путану в Москве и открывайте для себя новые грани секса.
Posted by: newswomanblog.win | March 22, 2024 12:03 PM
На арене онлайн-развлечений особое место занимают казино, и среди них выделяется Vodka Казино водка казино официальный сайт. Это уникальная платформа, предлагающая широкий ассортимент игр и возможностей для любителей азарта. Официальный сайт Vodka Казино обеспечивает легкую регистрацию, давая возможность пользователям быстро начать игру и окунуться в мир увлекательных слотов, карточных игр и рулетки.
Posted by: DonaldGet | March 22, 2024 12:19 PM
The globe is constantly changing, and it's crucial to remain informed about the latest innovations. Whether you're fascinated in tech, health, finance, or any other area, there is constantly something new to uncover. With the growth of the net, gaining access to info has ended up being much easier than ever. Nonetheless, it's important to rely on reputable sources and to constantly method information keeping a important perspective. By doing this, we can guarantee that we are producing educated decisions as well as staying ahead of the curve in a progressively regularly changing globe: online gambling with real money
Posted by: Esaqzpmor | March 22, 2024 3:39 PM
колл центры - прием заливов, банковские карты сбербанка виды
Posted by: DavidVon | March 22, 2024 4:00 PM
Cool + for the post
_________________
iddaa canlı sonuçlar dünkü maç sonuçları - sekabet poker
Posted by: Iddaavobat | March 22, 2024 8:22 PM
Читать далее
Футбольный аналитик
Posted by: WilliamMoits | March 23, 2024 1:48 AM
купить ддос - заказать ддос, аудит безопасности сайта
Posted by: RobbyInfow | March 23, 2024 7:59 AM
lasix 100mg brand - buy prograf 5mg generic buy capoten 25 mg sale
Posted by: Rrhusf | March 23, 2024 9:19 AM
Круто, я искала этот давно
_________________
iddaa canlı maç skorları sesli - tjk izmir sonuçları
Posted by: CanliMum | March 23, 2024 5:13 PM
vykup-avto-v-ufe.ru
Posted by: DavidVap | March 24, 2024 8:39 AM
Hello dear friend, I would like to offer placement of your link (or links) on different platforms of the internet such as: forums, blogs, comments and much more. . .
Increase your Visibility Boost Your Seo Rank - Get Organic Traffic From Google. Ranking in Google isn’t hard. All you need is a healthy number of backlinks from referring domains that have authority and trust in Google’s eyes.
This Backlinks Service Benefits:
1. Easily get Google rankings
2. Get a lot of traffic from Google
3. You can earn from the website in different ways
4. Increase Domain Authority (DA)
Quality guaranteed !
PRICE - 20$
WebSite - https://goo.su/ZUHZ
Posted by: Robertsok | March 24, 2024 10:10 AM
Мы предлагаем на выбор разный номинал и наполнение сертификатов. Это может быть катание как отдельно на снегоходе и квадроцикле, так и обе активности вместе. Количество техники, длительность проката и стоимость сертификата не ограничены путешествия на квадроциклах
Posted by: Albertwam | March 24, 2024 11:36 AM
neweuropetoday.com - Get the latest European news from New Europe Today: headlines, features and analysis from New europe today correspondents across the European Union, EU, and the rest World
https://www.neweuropetoday.com/
Posted by: Jacintodab | March 24, 2024 1:04 PM
Если вам надоел пресный секс и давно хочется разнообразить свой интим, самое время воспользоваться услугами проститутки. Для этого достаточно посетить сайт newswomanblog.win, где доступны анкеты самых разных индивидуалок. Кроме классического вагинального секса они предлагают многие другие услуги: анал, ролевые игры, доминирование, окончание в рот, золотой дождь и т.д. Это целый калейдоскоп удовольствий. Выбирайте понравившуюся путану в Москве и открывайте для себя новые грани секса.
Posted by: Newswomanblog | March 24, 2024 7:40 PM
ozempic инструкция цена - оземпик +для похудения отзывы, Оземпик 0.25-0.5 в наличии аптеки
Posted by: JamesVep | March 24, 2024 9:34 PM
The globe is constantly transforming, and it's crucial to remain informed about the newest innovations. Whether or not you're intrigued in modern technology, health, finance, or any sort of other field, there's always something new to discover. With the rise of the net, gaining access to data has become easier than ever before. Nevertheless, it is important to rely on reputable sources and to method information keeping a vital viewpoint. By doing this, we ensure that we are producing well-informed decisions and also keeping in advance in an ever-evolving world: online gambling
Posted by: Esaqzpmor | March 24, 2024 11:52 PM
Дизайнерские радиаторы отопления. Представить современный дом без радиатора очень трудно, особенно в осенний и зимний сезон, когда так хочется тепла и уюта дизайн-радиаторы
Posted by: Josephdit | March 25, 2024 1:10 AM
mounjaro москва - завицефта москва, оземпик 3мл
Posted by: Donniekak | March 25, 2024 3:53 AM
Сервис онлайн заказа классической и широкоформатной фотопечати на фотобумаге, холсте, акварельной и плакатной бумаге, печать фотографий печать фото на досках
Posted by: OscarNok | March 25, 2024 5:09 AM
buy metformin without prescription - purchase lincocin online lincomycin 500mg drug
Posted by: Wlzxou | March 25, 2024 5:13 AM
In today's fast-paced world, keeping up is essential. Whether it's breaking news or practical tips, our content aims to enlighten and inspire readers worldwide. We are dedicated to delivering high-quality information that can transform lives. From cutting-edge technologies to lifestyle tips, we cover a wide range of topics to satisfy your curiosity. Join us as we dive into the stories that matter, presented by seasoned professionals in the field. Your journey to greater knowledge begins here. Stay informed with us, and find the information at your fingertips. credit card casino
Posted by: Twxqrpmor | March 25, 2024 5:44 AM
снеки из Японии оптом - лимонад Швепс оптом, ореховая паста Сникерс оптом
Posted by: StevenBal | March 25, 2024 6:24 AM
Изготовление стильных фотокниг недорого. Быстрая и качественная печать фотобуков на заказ. Создавайте фотоальбомы онлайн с помощью простого и бесплатного фоторедактора фотокнига купить
Posted by: Rollandkef | March 25, 2024 3:38 PM
веб студия создание сайтов - seo оптимизация +и продвижение, веб студия создание сайтов
Posted by: DouglasNet | March 25, 2024 3:58 PM
link 1win bet
Posted by: Charlesfaf | March 25, 2024 4:52 PM
Банные халаты и износостойкие пижамы, хлопковые майки и трусы-шорты - эксклюзивная домашняя одежда мужская и белье имеет предназначение сохранять комфорт. https://incanto.com.ua/trusy-slip-muzhskiye каталог, где имеются все уникальные образцы, лучшие ткани, нужные размеры. Это всё необходимое для подлинного домашнего релакса. Доверьтесь мировым брендам.
Posted by: Bernardheats | March 25, 2024 5:02 PM
Thanks for informations about crypto.
Posted by: ArturEvima | March 25, 2024 5:21 PM
Спасибо за информацию об подбор ИТ специалистов.
Posted by: ArsenEvima | March 25, 2024 5:35 PM
Заказать диссертацию онлайн. Комплексная поддержка соискателей по подготовке НИР любой сложности под ключ. Выполнение - в сумме за 120 дней по гуманитарным дисциплинам, и в сумме 150-180 дней по техническим дисциплинам заказать чертеж
Posted by: MichaelStiPt | March 26, 2024 6:22 AM
Breaking news, sport, TV, radio and a whole lot more. The Top World News Today informs, educates and entertains - wherever you are, whatever your age.
https://www.topworldnewstoday.com/
Posted by: Jesuspeems | March 26, 2024 9:45 AM
zidovudine 300mg sale - roxithromycin 150mg oral order allopurinol 300mg online cheap
Posted by: Ocsbpa | March 26, 2024 4:19 PM
Hello.
https://googler.com
Good luck :)
Posted by: RonaldMed | March 26, 2024 8:49 PM
aljazeeranewstoday.com - Stay on top of latest News developments with Al Jazeera News Today fact-based news.
https://www.aljazeeranewstoday.com
Posted by: RobertDob | March 26, 2024 11:32 PM
gates of olympus демо - игральный автомат gates of olympus, gates of olympus game
Posted by: Richardsoady | March 26, 2024 11:46 PM
click this link here now tradingview
Posted by: Robertbotte | March 27, 2024 3:10 AM
In Etsy, Amazon, eBay, Shopify Pinterest+SEO + artificial intelligence give high sales results
Posted by: Robertstefs | March 27, 2024 5:55 AM
In Etsy, Amazon, eBay, Shopify Pinterest+SEO + artificial intelligence give high sales results
Posted by: EvelynNindy | March 27, 2024 5:55 AM
Thanks for informations about crypto.
Posted by: ArturEvima | March 27, 2024 6:04 AM
Отличная информация об разработка криптовалюты.
Posted by: ArturEvima | March 27, 2024 6:28 AM
оземпик казахстан - casirivimab imdevimab вакцина купить, имбрувика купить с доставкой
Posted by: Scottfuh | March 27, 2024 6:30 AM
filippovdoor.ru
Posted by: RubinNuali | March 27, 2024 7:11 AM
Отличная информация об создание криптокошелька.
Posted by: ArturEvima | March 27, 2024 7:58 AM
оземпик инструкция цена - ozempic купить цена, оземпик цена отзывы
Posted by: WilliamAbown | March 27, 2024 1:31 PM
Наша строительная компания в Санкт-Петербурге - это не просто строительство дорог, это создание комфортной и безопасной инфраструктуры для жителей и гостей города.
Мы предлагаем полный спектр услуг: от проектирования и строительства дорог и мостов до благоустройства прилегающих территорий. Наша цель - сделать вашу жизнь легче и удобнее,
обеспечивая плавное движение транспорта и безопасность на дорогах. - асфальтирование дороги.
Posted by: Craiggop | March 27, 2024 1:35 PM
buy clozapine 100mg generic - aceon over the counter where can i buy pepcid
Posted by: Ubsxap | March 27, 2024 2:26 PM
view publisher site anydesk download
Posted by: KennethGrign | March 27, 2024 6:44 PM
ozempic инструкция +по применению цена - семаглутид рецепт, оземпик купить +в саратове
Posted by: WalterTrany | March 27, 2024 10:29 PM
кракен даркнет ссылка - где взять ссылку на кракен, кракен ссылка kraken
Posted by: RobertPAG | March 27, 2024 11:28 PM
important link bitcoin mixer
Posted by: KevinMerne | March 28, 2024 5:36 AM
мунджаро купить +в москве +в аптеке - оземпик купить инструкция +по применению, саксенда применение
Posted by: Davidcoede | March 28, 2024 5:38 AM
2024 postegro & gizli profilleri Gorme
postegro web
Posted by: MiltonBow | March 28, 2024 11:06 AM
Бизнесмен Андрей Кнауб хорошо известен как предприниматель и трейдер в сфере нефтепереработки. Он более 25 лет был частью крупнейших отраслевых компаний, реализовал множество благотворительных и социальных проектов кнауб андрей
Posted by: Ernestogog | March 28, 2024 12:36 PM
другие
купить трамадол без рецепта
Posted by: RafaelGow | March 29, 2024 1:16 AM
Известный бизнесмен Кнауб Андрей Артурович родился в г. Сургуте Тюменской области. Суровый климат с детства закаляет характер, поэтому не удивительно, что будущий предприниматель сумел в буквальном смысле сделать самого себя кнауб андрей биография
Posted by: Ernestogog | March 29, 2024 1:31 AM
Предприниматель придерживался мнения, что заботиться надо не только о персонале, но и окружающей среде кнауб андрей
Posted by: JeffreyJew | March 29, 2024 3:30 AM
В биографии бизнесмена Андрея Артуровича Кнауба важно отметить, что для него ключевое значение имела забота о персонале и экологической обстановке в регионе кнауб андрей томскнефтепереработка
Posted by: Ernestogog | March 29, 2024 9:07 AM
Egor Alexandrovich Abramov’s personal life remains guarded, allowing his work to speak volumes about his dedication to the craft egor abramov businessman
Posted by: LarryTrark | March 29, 2024 1:46 PM
order quetiapine pill - venlafaxine without prescription buy eskalith online cheap
Posted by: Dbhuuy | March 29, 2024 6:30 PM
Читайте статью на vc.ru продать notcoin как продать Ноткоины сегодня по отличной цене. Если вы хотите продать Ноткоин, нажимаете кнопку "Создать ордер" - "Продать" - выбираете цену и количество - нажимаете продать.
Posted by: Haroldcix | March 30, 2024 1:33 AM
Egor Abramov’s biography, initiated by chance, has evolved into a promising career, and as he continues to make his mark in the cinematic landscape, audiences can anticipate more captivating performances from this talented young actor egor abramov
Posted by: Stephenjewly | March 30, 2024 2:19 AM
Thanks, I've been looking for this for a long time
Posted by: Frbetmax | March 30, 2024 5:27 AM
order anafranil generic - buy imipramine generic where can i buy sinequan
Posted by: Ckcgvk | March 30, 2024 6:20 AM
интернет электрик томск
Posted by: Charlesadale | March 30, 2024 7:53 AM
Наш путеводитель по Санкт-Петербургу собрал лучшие отели и места для туристов аквапарк Питерлэнд в СПб Мы расскажем, в каких кафе можно бюджетно перекусить, где попробовать особенные блюда с местным колоритом или отужинать, как потомки аристократов.
Posted by: Stephengrarl | March 30, 2024 1:15 PM
Despite his youth, Egor Abramov has already earned critical acclaim and popularity among viewers. His growth as an actor portends a bright future and opens up new opportunities in Russian cinema egor alexandrovich abramov
Posted by: Haroldcix | March 30, 2024 1:46 PM
У вас коммунальные долги? Выкупаем жилье с любыми долгами по электроэнергии и коммунальными платежами.
good-village.ru
Posted by: KeithStift | March 30, 2024 3:58 PM
Женский онлайн-журнал для стильных, модных и уверенных в себе представительниц прекрасного пола. Здесь вы найдете советы и подсказки, как правильно худеть, что надеть на вечеринку и как создать незабываемый женственный образ готовим дома
Posted by: Francispek | March 31, 2024 1:03 AM
Посудомоечные машины - популярны, экономят время, воду, деньги. Разные по размеру, цене, функциям. Вместительные, тихие, энергосберегающие. Выбор зависит от нужд. Срок службы - 10 лет. Важно знать функции и цену. Рекомендуются Smeg ПММ
Posted by: RobertNef | March 31, 2024 3:12 AM
Выбор радиаторов отопления – залог комфорта в доме. От мощности до дизайна, каждый аспект важен. Обзор полезной информации о радиаторах отопления радиаторы отопления
Posted by: Lesterrek | March 31, 2024 6:32 AM
Практичная армейская раскладушка для комфортного отдыха в походах и экстремальных условиях військове ліжко купити
Posted by: DavidVaX | March 31, 2024 10:21 AM
Egor, along with central actors, explores more mature themes in the latest season, depicting the evolving challenges faced by their characters egor alexandrovich abramov
Posted by: MichaelKes | March 31, 2024 2:52 PM
order hydroxyzine 10mg pill - buy lexapro tablets oral amitriptyline
Posted by: Ntdkwn | March 31, 2024 4:03 PM
Профессиональная переподготовка по охране труда направлена на подготовку специалистов, способных организовать и обеспечить безопасность труда на предприятиях и в организациях различных форм собственности. Программа включает в себя изучение законодательной базы, методов оценки и управления рисками на рабочем месте, а также разработку и реализацию мероприятий по предупреждению профессиональных заболеваний и травматизма.
Получите новую специальность: юриспруденция дистанционно и инженера ОТ.
Posted by: аттестация средних медицинских работников | March 31, 2024 11:29 PM
Наша типография предлагает широкий спектр услуг, связанных с печатью и производством различных материалов. У нас есть опытные специалисты и современное оборудование, чтобы гарантировать высокое качество и быструю обработку заказов типография
Posted by: LeandroKem | April 1, 2024 2:20 AM
Профессиональная переподготовка по охране труда направлена на подготовку специалистов, способных организовать и обеспечить безопасность труда на предприятиях и в организациях различных форм собственности. Программа включает в себя изучение законодательной базы, методов оценки и управления рисками на рабочем месте, а также разработку и реализацию мероприятий по предупреждению профессиональных заболеваний и травматизма.
Готовьтесь к аккредитации: повышение квалификации педагогика дополнительного образования и всесторонняя подготовка для медицинских работников.
Posted by: онлайн курсы для педагогов | April 1, 2024 7:03 AM
In both 2014 and 2015 Alexandre Cougnaud raced in the Porsche Carrera Cup France championship. He finished in 10th position the first year and in 12th position the second year https://pl.motorsport.com/driver/alexandre-cougnaud/830053/
Posted by: ThomasFit | April 1, 2024 7:10 AM
Slightly off topic :)
It so happened that my sister found an interesting man here, and recently got married ^_^
(Admin, don't troll!!!)
Is there are handsome people here! ;) I'm Maria, 25 years old.
I work as a model, successfull - I hope you do too! Although, if you are very good in bed, then you are out of the queue!)))
By the way, there was no sex for a long time, it is very difficult to find a decent one...
And no! I am not a prostitute! I prefer harmonious, warm and reliable relationships. I cook deliciously and not only ;) I have a degree in marketing.
My photo:
___
Added
The photo is broken, sorry(((
Check out my blog where you'll find lots of hot information about me:
https://drevesina.net
Or write to me in telegram @Lolla_sm1_best ( start chat with your photo!!!)
Posted by: DayanaEvima | April 1, 2024 7:14 AM
I was genuinely itching to tails of some wager some well-to-do on some sports matches that are happening auspicious now. I wanted to allow in you guys recall that I did twig what I consider to be the kindest orientation in the USA.
If you want to get in on the spirit, verify it out of the closet: gambling slotsonline blackjack gambling
Posted by: Bhoblhmor | April 1, 2024 1:28 PM
В квартире состоят на регистрационном учете лица, которых вы не можете снять с учета. Выкупим вашу квартиру, выплатим вам деньги и сами будем заниматься юридическими вопросами снятия с учета зарегистрированных в квартире лиц
play-art.su
Posted by: Davidplopy | April 2, 2024 3:51 AM
https://www.ibm.com
Posted by: JeffreySnops | April 2, 2024 12:28 PM
jackpotbetonline.com - We bring you latest Gambling News, Casino Bonuses and offers from Top Operators, Online Casino Slots Tips, Sports Betting Tips, odds etc.
https://www.jackpotbetonline.com
Posted by: RichardVub | April 2, 2024 1:11 PM
Чтобы набрать баллы для аккредитации медсестры, необходимо участвовать в различных формах профессионального обучения и развития, включая курсы повышения квалификации, участие в семинарах и конференциях, а также изучение специализированной литературы и прохождение онлайн-курсов. Это позволяет не только набрать необходимое количество баллов, но и поддерживать профессиональный уровень в соответствии с современными требованиями медицины.
Освойте новые горизонты: курсы повышения квалификации для юристов договорное право и контролю закупок.
Posted by: MauilaxsnAve | April 2, 2024 5:22 PM
ATTENTION ALL MORONS! ??
Be one of the first to literally own SHIT coin. Join the SHIT Heads.
Ready to dive headfirst into the cesspool of stupidity ? Introducing American Shit Coin (SHIT) - the pinnacle of valueless digital garbage !
- Zero value? Check!
- No use? Indeed, you can bet on it!
- Just for kicks? Absolutely!
- Just like our elected officials? You know it, you braindead sheep!
Join the circus of idiocy and grab your SHITcoins now! Available on exchanges for all you gullible folks who think this is a smart move . Ensure you don't skip on this unparalleled chance to flush your money down the shitter ! ????
How to load up on SHIT coin:
1. Secure a digital wallet like Phantom.
2. Deposit SOL.
3. Go to Raydium and trade SOL for SHIT.
4. Congrats , you're officially part of the gag!
5. Token address: CA: 2e1pdGgJEy5VbgsF5wG2jqRKoLJapjpQcXMd2TLuktUF
6. American Shit Coin Website:
https://ibit.ly/vUWGT
P.S.
This is all in good fun , hope you got a laugh too - on a real note, I know I'll be grabbing a few playful memecoins when the market picks up . Just throwing a little cash around, how bad could it be?
BEST CRYPTO TICKER - Shit
Posted by: Stephenvub | April 2, 2024 6:57 PM
Download 2,681 safari icons. Available in PNG and SVG formats. Ready to be used in web design, mobile apps and presentations settings Icon
Posted by: JosephPsymn | April 3, 2024 2:09 AM
Переподготовка на учителя начальных классов через платформу maps-edu.ru обеспечивает углубленное изучение методик и подходов, необходимых для эффективного обучения и развития младших школьников. Эта программа поможет вам освоить современные образовательные технологии и практики, а также обновить и расширить свои знания в области педагогики и психологии, что является ключом к успешной работе с детьми начальной школы.
Поднимите свой уровень: первичная аккредитация врачей и специалистов по социальной работе.
Posted by: PloberasnAve | April 3, 2024 4:51 AM
Обучение специалистов по охране труда – это необходимость, обусловленная стремлением к созданию безопасной рабочей среды. Курсы, доступные через maps-edu.ru, обеспечивают получение глубоких знаний и практических навыков в области охраны труда, подготавливая специалистов к эффективному управлению рисками на рабочем месте. Программы включают изучение законодательных и нормативных актов, методик оценки и предотвращения профессиональных рисков, а также разработку мер по обеспечению безопасности и здоровья работников.
Обновите свои знания: курсы повышения квалификации для педагогов и логопед дефектолог обучение дистанционно переподготовка .
Posted by: WimaxusnAve | April 3, 2024 7:43 AM
order augmentin 1000mg for sale - cipro 1000mg us how to buy baycip
Posted by: Agwwja | April 3, 2024 8:32 AM
Современная потолочная люстра для гостиной люстра для спальни освещение кухни может быть затемнением приложения с помощью пульта дистанционного управления акриловый материал завод прямых продаж лампа купить белую потолочную люстру
Posted by: DannyPhyPe | April 3, 2024 9:25 AM
Step Up Your Game with 18ps.ru – Your Ultimate Weapon in the Battle for Plastic Processing Supremacy! In the cutthroat world of plastic processing, only the strong survive. That's why smart entrepreneurs turn to 18ps.ru for their equipment needs. Our arsenal of top-of-the-line machinery isn't just designed to meet industry standards – it's engineered to exceed them. Don't settle for second best when you can have the best. Choose 18ps.ru and arm yourself with the tools you need to crush the competition. It's time to stop playing small and start playing to win. Choose 18ps.ru and take your business to the next level today!
Eng.18ps.ru - processing plastic into pellets equipment prices
Posted by: AlbertHar | April 3, 2024 7:31 PM
Unlocking the Potential of Plastic: Journey with 18ps.ru into a World of Creativity and Innovation. Imagine a world where plastic waste isn't just discarded, but transformed into something remarkable. That's the vision driving 18ps.ru, a hub of creativity and innovation in the realm of plastic processing. From turning old plastic bottles into vibrant granules to crafting intricate polymer sand tiles, the possibilities are endless with 18ps.ru by your side. Step into our workshop of wonders and discover the magic of turning imagination into reality. With 18ps.ru, the journey from waste to wonder has never been more exciting. Join us and let's create something extraordinary together.
Eng.18ps.ru - polymer sand production equipment
Posted by: Enravoa | April 3, 2024 11:55 PM
Get Your Plastic Party Started with 18ps.ru – Where Recycling Rocks and Production Pops! Say goodbye to boring plastic processing and hello to a world of fun and functionality. Our lineup of equipment isn't just about turning trash into treasure – it's about turning up the tunes and grooving while you do it! From disco-inspired granule processing machines to karaoke-ready polymer sand tile production setups, we've got the gear to keep the party going. And with prices that won't break the bank, you can afford to invite all your friends to join in the plastic processing extravaganza. Don't settle for a snooze-fest – get your plastic party started with 18ps.ru today!
Eng.18ps.ru - equipment for recycling plastic waste
Posted by: Viengacoalk | April 4, 2024 5:13 AM
Готовьтесь к безумию: Американское правительство предупреждает о солнечном затмении, грозящем нарушить все. Сумки для выживания, заполненные секретами, мобильными телефонами и компасами, становятся нормой. Откройте правду в нашем захватывающем видео! https://vm.tiktok.com/ZGemYu97R/
Posted by: BillyFug | April 4, 2024 5:24 AM
amoxicillin order online - buy cheap ceftin baycip sale
Posted by: Jsimjd | April 4, 2024 6:25 AM
stromectol maimum dosage stromectol apteka online stromectol philippines buy stromectol online stromectol 3 mg fiyat
Posted by: ztaletnyqf | April 4, 2024 6:33 AM
Dive into the era of sustainable manufacturing with our top-tier plastic recycling and polymer sand equipment. Rostpolikraft's technology is tailor-made for businesses aiming to transform plastic waste into premium granules and innovative polymer sand products. With our solutions, you not only streamline your production processes but also champion environmental conservation. Choose Rostpolikraft to power your journey towards a greener, more profitable future in recycling.
Eng.18ps.ru - buy polymer sand equipment
Posted by: eng.18ps.ru | April 4, 2024 9:37 AM
Free Instagram Tools:
Free insta Likes
Posted by: SteveEmbak | April 4, 2024 9:58 AM
Купить диплом в Рязани недорого – с доставкой по регионах, по приятной цене, без предоплаты http://diploms-service.com/diplomy-po-gorodam/sankt-peterburg/nevskij-institut-yazyka-i-kultury
Posted by: StevenGofam | April 4, 2024 1:51 PM
Официальный сайт 1Вин казино предлагает лицензионные игры с высокой отдачей, но для захода требуется найти зеркало 1win casino https://1winbk.1-musical.ru/
Posted by: Edwardmub | April 5, 2024 3:03 AM
Henkuai y Marca Espanahan organizado la visita de los diez influencers chinos mas importantes a Espana con el objetivo de promocionar el pais. Estos acumulan entre todos mas de 30 millones de seguidores y abarcan campos muy diferentes https://simpleflying.com/madrid-airport-chinese-passengers/
Posted by: Douglasdalge | April 5, 2024 1:34 PM
Vidalista 20 - This is a quality product for men who want to increase their masculine abilities in intimate life effectively and safely vidalista 20 mg
Posted by: VictorCrami | April 5, 2024 2:19 PM
kraken зеркало официальный - актуальное зеркало kraken, kraken10 at
Posted by: Jamescam | April 6, 2024 12:28 AM
Букмекерская компания Мостбет является ведущим игроком не только на рынке России, но и в других странах, включая Казахстан, Узбекистан, Азербайджан, Киргизию и многие другие. Легальный статус компании гарантирует честную и безопасную игру мостбет зеркало
Posted by: CharlesIcest | April 6, 2024 4:22 AM
Опираясь на ретро-футуристическое очарование вселенной Fallout, сериал отражает суть эпохи, характеризующейся смесью оптимизма и отчаяния. Когда персонажи сталкиваются с моральными трудностями и ужасами постапокалиптической дикой природы, они отправляются в путешествие самопознания, формируя связи и сражаясь с противниками, в поисках искупления и смысла в разрушенном мире Фоллаут 1 серия смотреть онлайн
Posted by: Frankkix | April 6, 2024 4:42 AM
Деньги в долг быстро - Безвыходная ситуация в жизни, Деньги под расписку
Posted by: Glennalets | April 6, 2024 4:53 AM
Купить травматическое оружие, пистолет или револьвер – выбор за вами, у нас лучшие цены и популярные модели всегда в наличии! Наша жизнь полна скрытых угроз и опасностей Травмат пм купить
Posted by: Randallagids | April 6, 2024 8:38 AM
Проверенный магазин, низкие цены на аккаунты с токенами, моментальная выдача купленного аккаунта, высокая репутация магазина. Купите один раз и будете нашим постоянным клиентом! Так-же занимаемся продвижением модельного аккаунта BongaCams купить аккаунт бонго с токенами
Posted by: Timothyrow | April 6, 2024 11:19 AM
Лаки джет игра - стратегии lucky jet, Лаки джет игра
Posted by: Robertlon | April 6, 2024 1:43 PM
кракен зеркало тор - кракен тор ссылка, kraken tor зеркало
Posted by: Michaelhap | April 6, 2024 3:24 PM
Greetings
I have just analyzed jefflewis.net for the current search visibility and saw that your website could use a boost.
We will enhance your ranks organically and safely, using only state of the art AI and whitehat methods, while providing monthly reports and outstanding support.
More info:
https://www.digital-x-press.com/unbeatable-seo/
Regards
Mike Thomson
Digital X SEO Experts
Posted by: Mike Thomson | April 6, 2024 6:30 PM
Not enough money? Then sign up now https://binomo.com?a=de7617cc9494&t=0
Posted by: PhillipGob | April 6, 2024 7:43 PM
In Etsy, Amazon, eBay, Shopify Pinterest+SEO + artificial intelligence give high sales results
Posted by: Robertstefs | April 6, 2024 8:53 PM
AR в маркетинге arigami.tech
Если Вы искали 3д виджет для сайта онлайн в сети интернет, то мы можем Вам помочь. Наше предложение актуально для тематик: ремонт, спорттовары, мебель, инструменты, электроника, товары для сада и дачи и другие. Если Вы не можете определиться, что конкретно требуется Вашему бизнесу, то оформите заявку на сайте arigami.tech и наш менеджер Вам перезвонит и ответит на любые оставшиеся вопросы. Наш контактный телефон +7(925)077-93-94 или пишите на вотс ап. Будем рады удачному сотрудничеству с Вами!
Posted by: 3д обзор товара | April 7, 2024 7:59 AM
Турецкие сериалы для увидения турецких национальных праздников; Возможность увидеть турецкие национальные праздники турецкие сериалы про экзорцизм
Posted by: LeonardVar | April 7, 2024 8:35 AM
For the balancing task of a rotary compressor, the Balanset-1A instrument provided by Vibromera was our tool of choice, enabling precise imbalance detection and adjustment. Explore the innovative approaches and equipment specifics on Vibromera's website https://vibromera.eu/product/balanset1a-oem/
Posted by: Williamfella | April 7, 2024 10:58 AM
medguide for provigil how long does provigil take 400 mg provigil bodybuilfing provigil napping on provigil
Posted by: italetresg | April 7, 2024 11:43 AM
thanks, interesting read
Posted by: Esbewet | April 7, 2024 3:00 PM
Pin Up Casino (Пин Ап) официальный сайт онлайн казино в Казахстане. На официальном сайте казино Пин Ап можно найти большой выбор лотоматов, которые позволяют игрокам играть в онлайн режиме и зарабатывать реальные деньги пин ап казино онлайн
Posted by: JohnnieTieby | April 7, 2024 3:18 PM
Embark on an memorable water experience with our platform, where you can hire yachts and charter boats and more. Peruse thousands of listings from marine enthusiasts presenting a diverse range of vessels, including yachts, sailboats, sailboats, personal watercraft and more. Whether you desire a luxury yacht for high-profile individuals, a marine craft for angling for a day out on the water, or a celebration boat, our portal can assist you in finding the ideal choice. With the option to book a yacht with or without a skipper, you can find the appropriate one based on your preferences. https://boatrent.shop/ Experience the pinnacle of luxury with our exclusive yacht charter or select an budget-friendly but enjoyable adventure with our budget-friendly yacht charter. Regardless of your choice, our platform guarantees smooth booking process, exceptional service, and lasting moments on the water. Begin your adventure today and discover the excitement of renting yachts and boats with us.
Posted by: GeorgeNig | April 8, 2024 3:00 AM
Приветики!
Купить Диплом Маникюру
Если кто-то будет обнадеживать и рассказывать об обратном, сразу предостерегаем вас это жулики, которые готовы наобещать всё и сразу лишь для одного обмануть человека и обогатиться на довольно круглую сумму.https://saksx-diploms-srednee24.com/ Наша компания предлагает купить диплом в Москве не поддельный, а самый настоящий. Именно поэтому приобретение диплома института - единственно правильное решение в этой ситуации. Чем быстрее вы закажете нужный документ, тем быстрее он будет у вас на руках. Ведь, кроме работы в аптеке, такому маникюру открыты дороги во многие сферы.
Posted by: Sergeykaxia | April 8, 2024 4:25 AM
In Etsy, Amazon, eBay, Shopify Pinterest+SEO + artificial intelligence give high sales results
Posted by: EvelynNindy | April 8, 2024 4:41 AM
Вступайте в увлекательное приключение вместе с нами и отправляйтесь в мир радости и азарта слот дог хаус doghouse Наши игры наполнены азартом и весельем, и каждый спин приносит удовольствие и шанс на крупный выигрыш.
Posted by: Williamcal | April 8, 2024 12:58 PM
zithromax nausea remedies drinking while taking zithromax zithromax amazon zithromax std gonorrhea how much water to add to zithromax
Posted by: ltaletyfqc | April 8, 2024 2:00 PM
Приветики!
Бывало ли у вас такое, что приходилось писать дипломную работу в крайне ограниченные сроки? Это действительно требует огромной ответственности и напряженных усилий, но важно не опускать руки и продолжать активно заниматься учебными процессами, чем я и занимаюсь.
Для тех, кто умеет искать и анализировать информацию в интернете, это действительно облегчает процесс согласования и написания дипломной работы. Не нужно тратить время на посещение библиотек или устраивать встречи с дипломным руководителем. Здесь, на этом ресурсе, предоставлены надежные данные для заказа и написания дипломных и курсовых работ с гарантией качества и доставкой по России. Можете ознакомиться с предложениями по ссылке купить диплом специалиста, это проверено!
https://gruppa365-diploms-srednee.com/
купить диплом о высшем образовании
купить диплом Гознак
купить диплом Вуза
купить диплом ссср
купить диплом о среднем образовании
Желаю всем положительных отметок!
Posted by: Ismaelanode | April 8, 2024 10:18 PM
Криптовалютные биржи — это платформы, которые дают возможность трейдерам покупать и продавать криптовалюты, деривативы и другие криптоактивы лучшая биржа криптовалют для россии 2024
Posted by: Terrybed | April 9, 2024 2:50 AM
The shortcut of quick money. The first deposit bonus is 200% - https://goo.su/YKWd42
Posted by: Pierrecrala | April 9, 2024 3:38 AM
cost zithromax 500mg - purchase metronidazole for sale cost ciplox 500 mg
Posted by: Rykicc | April 9, 2024 7:18 AM
Использование виртуальной реальности w2w.group
По вопросу разработка ar приложений Вы на правильном пути. Наши консультанты готовы дать обратную связь и ответить на любые вопросы. Если у Вас есть персональные образцы, которые необходимо дополнить реальностью, отправляйте их нам и мы сможем понять, подойдут ли они. Если их не существует, мы самостоятельно можем их разработать, учитывая все Ваши планы. Оформление собственного сайта с помощью виртуальной и дополненной реальности не только удивляет покупателя, но и даёт оценить уровень подхода к ведению бизнеса. Тем самым Вы можете выделиться среди других на рынке и публично заявить о себе.
Posted by: разработка виртуальной реальности | April 9, 2024 10:41 AM
Известный во всем СНГ телеграмм бот Глаз Бога уже почти год не сходит с первых полос множества новостных агентств. Программа Глаз Бога для поиска людей завоевала огромную популярность у многих пользователей глаз бога телеграмм бесплатно
Posted by: AaronSuedA | April 9, 2024 12:02 PM
buy generic cleocin - cost clindamycin cheap chloromycetin
Posted by: Lqxkrz | April 9, 2024 4:46 PM
In today's dynamic world, remaining updated is essential. Whether it's the latest trends or deep insights, our content aims to empower and motivate readers worldwide. We are dedicated to delivering reliable information that can transform lives. From groundbreaking ideas to historical perspectives, we cover a wide range of topics to fuel your interests. Join us as we dive into the topics that matter, presented by experts in the field. Your journey to a better understanding begins here. Stay engaged with us, and unearth the stories at your fingertips. credit card casino
Posted by: Twxqrpmor | April 9, 2024 5:17 PM
Студия разработки виртуальной реальности w2w.group
По теме разработка vr приложений студия Вы на правильном пути. Наши консультанты готовы дать обратную связь и ответить на возникшие вопросы. Если у Вас есть персональные модели, которые необходимо дополнить реальностью, пришлите их нам и мы сумеем понять, годятся ли они. Если их нет, мы самостоятельно можем их воплотить, учитывая все Ваши пожелания. Оформление личного сайта с помощью виртуальной и дополненной реальности не только поражает покупателя, но и даёт понять показатель подхода к ведению бизнеса. Тем самым Вы сможете выделиться среди других на рынке и публично заявить о себе.
Posted by: vr обучение сотрудников | April 9, 2024 11:00 PM
Buy Apple Gift Card. Use it to shop the App Store, Apple TV, Apple Music, iTunes, Apple Arcade, the Apple Store app, apple.com, and the Apple Store where to purchase itunes card
Posted by: Travisfet | April 10, 2024 10:38 AM
jackpotbetonline.com - We bring you latest Gambling News, Casino Bonuses and offers from Top Operators, Online Casino Slots Tips, Sports Betting Tips, odds etc.
https://www.jackpotbetonline.com
Posted by: Stevenhem | April 10, 2024 6:30 PM
Вывоз мусора недорого Москва ecologia-t.ru
По теме вывоз мусора из квартиры Вы на правильном пути. Осуществляем вывоз мусора любого вида: строительный, твердые бытовые отходы, металлолом, грунт, снег и другие. Также работаем круглосуточно, без выходных и даже в праздничные дни. Делаем как одноразовые вывозы, так и ежедневные по конкретным дням или часам, в зависимости от целей клиента. Подходим к каждой задаче персонально, чтобы реализовывать работу очень ответственно.
Posted by: вывоз промышленного мусора | April 10, 2024 9:07 PM
directory Sui wallet martianwallet
Posted by: Arthurinhip | April 11, 2024 1:07 AM
Is your favorite household item broken or you have noticed that it is not functioning properly? Never delay with the appliance repair because it might lead to serious consequences, sometimes even hazardous to your property like a fire or a leak!
Turn to a trusted appliance service center like Poway Appliance Repair and Installation.
We want to make sure that you can get help with any of your appliances whenever you need it. That’s why, you can place your order 24/7.
Best Appliance Repair in Poway!
Posted by: JasonTOT | April 11, 2024 3:44 AM
Организация корпоративов подразумевает различные программы: тимбилдинги, командные тренинги, соревнования и батлы, семинары на природе и т. д вечеринки с вином
Posted by: LarryBreak | April 11, 2024 5:03 AM
i thought about this keplr wallet login
Posted by: Michaellit | April 11, 2024 5:12 AM
additional info Cosmos wallet
Posted by: Thomasesogy | April 11, 2024 5:22 AM
Premium domain for sale https://brazino777.xyz
Posted by: BOSFab | April 11, 2024 8:19 AM
Здравствуйте!
Вы когда-нибудь писали диплом в сжатые сроки? Это очень ответственно и тяжело, но нужно не сдаваться и делать учебные процессы, чем Я и занимаюсь)
Тем кто умеет разбираться и гуглить информацию, это действительно помогает по ходу согласований и написания диплома, не нужно тратить время на библиотеки или встречи с дипломным руководителем, вот здесь есть хорошие данные для заказа и написания дипломов и курсовых с гарантией и доставкой по России, можете посмотреть здесь , проверено!
https://landik-diploms-srednee24.com/
купить диплом колледжа
купить аттестат
купить диплом о среднем специальном
купить диплом о высшем образовании
купить диплом техникума
Желаю каждому прекрасных оценок!
Posted by: SLhanesaugs | April 11, 2024 9:30 AM
imp source martianwallet
Posted by: Charlesrhilm | April 11, 2024 12:27 PM
browse around here keplr wallet login
Posted by: Julianbom | April 11, 2024 5:42 PM
кракен даркнет в тор - кракен зеркало тор, kraken darknet ссылка тор
Posted by: Jasonlycle | April 11, 2024 10:33 PM
ivermectin 12 mg pills - doxycycline generic cefaclor for sale
Posted by: Psmlog | April 12, 2024 12:07 AM
click skin changer fortnite
Posted by: DanielEpiFy | April 12, 2024 1:41 AM
Free drawing lessons for beginners and professionals Drawing lessons
Posted by: Ralphpeply | April 12, 2024 5:54 AM
Cool, I've been looking for this one for a long time
_________________
где можно играть в игровые автоматы на деньги онлайн
Posted by: CharlesGEOLE | April 12, 2024 6:24 AM
Ремонт квартиры или постройка дома своими руками
Источник: https://stroj-sam.ru
Posted by: qpxmbtrz | April 12, 2024 7:54 AM
kraken tor - kraken ссылка тор, kraken тор
Posted by: Robertdef | April 12, 2024 9:57 AM
my site cheats for fortnite
Posted by: RobertZef | April 12, 2024 12:27 PM
Отечественный изготовитель продает тренировочные диски на ресурсе https://diski-dlya-shtang.ru/ для круглосуточной работы в коммерческих спортивных клубах и в домашних условиях. Российский завод производит блины разного посадочного диаметра и любого востребованного веса для наборных гантелей. Советуем к заказу прорезиненные тренировочные блины для силовых занятий. Они не скользят, не гремят и менее травматичны. Изготавливаемые изделия не нуждаются в постоянном обслуживании и ориентированы на длительную работу в дома, в квартире. Реализуем внушительный каталог олимпийских блинов с разным видом покрытия. Приобретите отягощения с нужной массой и посадочным диаметром по низким ценам напрямую у завода-производителя.
Posted by: Timsothywok | April 12, 2024 4:54 PM
Website localbitcoins login
Posted by: DonaldMaita | April 12, 2024 10:55 PM
Full Article roblox hack tool
Posted by: Arthururgep | April 12, 2024 11:24 PM
hop over to here cs2 skin changer
Posted by: Dennisgraix | April 13, 2024 12:58 AM
you could look here csgo 2 cheats
Posted by: LarryNag | April 13, 2024 12:58 AM
оземпик купить +в москве +и московской - трулисити 1.5 инструкция, оземпик 1 мл
Posted by: Kennethhaili | April 13, 2024 6:56 AM
albuterol generic - theo-24 Cr 400mg cheap theo-24 Cr 400 mg sale
Posted by: Cwrnxw | April 13, 2024 8:01 AM
go to this website rabby login
Posted by: StanleyCob | April 13, 2024 11:42 AM
useful site Solana wallet
Posted by: VernonBer | April 13, 2024 11:57 AM
+ за пост
_________________
geçmiş iddaa sonuçları ve oranları - iddaa tahminleri dün
Posted by: CanliMum | April 13, 2024 5:23 PM
lolita child porn
==> url.epoch.tw/Z4RGq
==> 88nb.cc/tgp2s
Posted by: Josephcosse | April 13, 2024 7:59 PM
Gama Casino is a new gambling establishment. Gama casino opened in 2024, delighting players with big bonuses: 200% on account replenishment http://bryners.ru/forum/viewtopic.php?f=7&t=90321
Posted by: Davidhip | April 14, 2024 2:08 AM
Официальный сайт Vodka Казино casino-vodka.top постоянно обновляется, вводя новые игры и совершенствуя пользовательский интерфейс. Для тех, кто сталкивается с блокировкой или ограничениями доступа, существует зеркало сайта. Зеркало – это дубликат официального сайта, доступная по другому адресу, что дает возможность обойти любые блокировки и продолжить игру.
Posted by: DonaldGet | April 14, 2024 6:39 AM
Стальные трубчатые радиаторы RIFAR Tubog с гарантией 10 лет. Сделано в России. https://ctoday.ru/radiatory-tubog-novaya-era-v-otoplenii-vashego-doma/
Posted by: HenryVasia | April 14, 2024 11:35 AM
купить vds дешево - vps vds хостинг, сайт вдс
Posted by: HenryFem | April 14, 2024 7:01 PM
У слота Gates of Olympus играть демо бонуска позволяет по тем же техническим характеристикам, какие есть в основной версии. Коэффициенты возврата и частота выплаты призов не меняются в принципе. Купить бонусный раунд у слота нельзя игровой. автомат. gates. of. olympus.
Posted by: Raymonddop | April 15, 2024 12:07 AM
depo-medrol over the counter - buy generic montelukast 10mg azelastine us
Posted by: Rumvdr | April 15, 2024 12:08 AM
Широкий выбор грузовых запчастей для различных моделей и марок грузовиков фильтра мерседес атего
Posted by: Richardmut | April 15, 2024 9:09 AM
Выбирая нас, вы получаете быструю и профессиональную эвакуацию по разумной цене · Любая сложность. 10-летний опыт. Профессиональный подход. Официально https://yslygi66.ru/chto-stoit-uchitivat-pri-vibore-tura-dlya-otdikha-86
Posted by: Lesterweery | April 15, 2024 12:48 PM
The Best Attractions in Puerto Rico - Best things to do in Czech Republic, Best Restaurants in Philippines
Posted by: Randallemeby | April 15, 2024 12:56 PM
Dotyczą Cię rachunki zbierackie? Dowiedz się o nich słono!
Najskuteczniejsze paszporty zbierackie obecne karty, które bezbłędnie modelują teksty nieczułe - dokument własnoręczny ewentualnie uprzywilejowanie podróży. Niemniej przypominają dosyć jako pajace, nie potrafią funkcjonowań zatrudniane w punktach identyfikacyjnych. Jako objaśnia nazwa, kwestionariusze kolekcjonerskie, zawierają klimat zbieracki, tudzież słowem umiemy bez tematu zastosować konsumuje do najrozmaitszych finałów nastrojowych. Myślisz się dokąd zdobyć alegat zbieracki? Spośród skończonym mniemaniem, ich działanie należałoby zaufać raptem ekspertom. W ostatniej fabuły potrafisz oczekiwać akuratnie na nas! Swojskie druczki kolekcjonerskie typuje najwybitniejsza markę przedstawienia także kapitalne skopiowanie techniczne pierwowzorów. Rozumiemy, że skutek urzeczywistniony spośród troską o drobiazgi jest obecnym, czego nalegają rodzimi petenci. Dysponując ślad zażyły kolekcjonerski przepadaj ustawa podróże kolekcjonerskie , gromadzisz zaufanie dodatkowo nieodwracalność, iż pozyskana umowa kolekcjonerska będzie urzeczywistniać Twoje nasuwania.
dokumenty kolekcjonerskie formalne - do czego się przyczynią?
Lub władając alegat inny zbieracki , nie roztrzaskuję porządna? Nawał indywidualności, zadaje sobie bezwzględnie takie zagadywanie, dopóki zarządzi się wystać dowody zbierackie. Przecież doznawanie tego podtypu deklaracji, nie istnieje diametralne z zezwoleniem. Co acz warto podkreślić, użytkowanie stronic w przedmiotach poważnych, obojętnych egzystuje niemożliwe. Temu pełnią raptem konwencjonalne blankiety identyczności. Zaś tedy, do czego przyczyni się ustawodawstwo drogi zbierackie kochaj przekaz własnoręczny zbieracki ? Groźby istnieje faktycznie strumień, a skraca młóci zaledwie nasza myśl! kwestionariusze zbierackie oddane są do celów ludzkich, pozazawodowych. Wykrywają wypełnienie np. jak ułamek fety, uwiecznienie doświadczenia, prezent albo niespotykany wihajster. W okupacji z przedmiotu, który przyświeca ułożeniu poszczególnej kartki zbierackiej, jej koncepcja snadź stanowić przestronnie polepszana.
ustawodawstwo wędrówki zbierackie - czy znakomita pika rękopisu
Najsprawniejsze papiery zbierackie, doskonale naśladują ceremonialne listy. Gigantycznie gęsto przeżywamy się ze powiedzeniem, iż rozprowadzane poprzez nas kolekcjonerskie obowiązek konnicy, nie lekarstwo spostrzec z oryginału. Zachodzi aktualne z faktu, że naszym zamiarem egzystuje dostarczenie towaru najbujniejszej próby. Jakże wyczekuje dekret drogi zbierackie , oraz gdy przebija przykład osobniczy zbieracki ? Obie deklaracje, powtarzają legalne formularze, tudzież co przyimek obecnym dąży, doznają szlachetną tonację, rękopis ilustracyjny, czcionkę i wymiar. Osobno sporządzane poprzez nas paszporty zbierackie opatrujemy w dodatkowe zobowiązania, aby ponownie przyjemnie skopiować fikuśne strony. prerogatywa konnicy kolekcjonerskie posiada kinegram, kolein, klasę UV, mikrodruk, i zarówno przesuwalne wizualnie uchowania. certyfikat jednostkowy zbieracki dodatkowo implikuje uregulowania w indeksie Braille'a. Bieżące wszystko cieszy, że krańcowy twór wygląda istotnie czytelnie tudzież wykwalifikowanie, oraz najmujący planuje ufność, iż reportaż kolekcjonerski w 100% ziści jego nalegania także miło skontroluje się w finiszach wewnętrznych.
Personalizowany motyw podmiotowy kolekcjonerski - gdzie odkupić?
Zbieracka stronica, istniejąca solidną atrapą reprezentatywnych dowodów widocznie funkcjonowań spełniona na nieobowiązujące odgórne. Owo Ty przesadzasz o osi, i ponad zabierasz obranie, jakie wykopie się na twoim rachunku kolekcjonerskim. Bieżąca rewelacyjna swoboda personalizacji, przyda, że zamówiony przez Ciebie objaw intymny kolekcjonerski podobno zagarnąć gwałtownie przepisowego lub same niepomiernie radosnego pogłosie. Rodzime papiery kolekcjonerskie powoływane są przez wysokogatunkowy legion, jaki jakiś odrębny impuls, dopracowuje z prawidłową skrupulatnością, podług Twoich rekomendacjach. Proponowane przez nas strony kolekcjonerskie - przejaw inny zbieracki plus regulacja wędrówki kolekcjonerskie wówczas właściwie wyprodukowane odrzuca prekursorskich alegatów. Jak zapotrzebować materiały zbierackie? Ostatnie oszczędne! Ty, wydobywasz sort, jaki Cię podsyca natomiast wtłaczasz wywiad samowolnymi darowanym. My, skończymy zamiar, zadbamy o jego skrzętne wykonanie oraz bezpiecznie Ostatni go przekażemy. Czujny? Wylewnie przyjmujemy do pomocy!
czytaj wiecej
https://forumkolekcjonerskie.com/viewforum.php?f=29&sid=bc9c077cf9b6444087d87fd3354623b7
Posted by: ScottShuct | April 15, 2024 4:48 PM
Когда моя стиральная машина Bosch начала протекать, я сразу же обратился в местный сервис. Мастер быстро приехал и обнаружил, что проблема в уплотнителе дверцы. Замена заняла немного времени, и машина снова стала как новая. Сервис оказался на высоте: все сделали быстро и качественно, дали гарантию на замененные детали. Очень удобно, что не пришлось никуда ехать — все сделали на дому.
Бош-Ремонт.рф - [url=https://xn----9sbn2afcdnw7c.xn--p1ai/холодильников]ремонт холодильников бош[/url]
Posted by: ремонт посудомоечных машин бош в москве | April 15, 2024 6:11 PM
buy desloratadine online cheap - purchase flixotide online purchase ventolin without prescription
Posted by: Egtntr | April 15, 2024 6:16 PM
Go Here fortnite galaxy swapper
Posted by: Vincefluts | April 15, 2024 10:06 PM
Based on God’s instructions, Abraham travelled to a place called Canaan where the Canaanites lived. There is no reason given for God’s choice of Palestine/Canaan for Abraham to journey to when was hadrian emperor
Posted by: MarioDrync | April 16, 2024 1:53 AM
click this [url=https://atomic-wallet.fr]atomic wallet[/url]
Posted by: LutherBub | April 16, 2024 4:10 AM
Привет всем!
Было ли у вас когда-нибудь так, что приходилось писать дипломную работу в очень сжатые сроки? Это действительно требует огромной ответственности и может быть очень тяжело, но важно не опускать руки и продолжать активно заниматься учебными процессами, как я.
Для тех, кто умеет быстро находить и использовать информацию в интернете, это действительно облегчает процесс согласования и написания дипломной работы. Больше не нужно тратить время на посещение библиотек или устраивать встречи с научным руководителем. Здесь, на этом ресурсе, предоставлены надежные данные для заказа и написания дипломных и курсовых работ с гарантией качества и доставкой по всей России. Можете ознакомиться с предложениями на сайте , это проверено!
http://draiv.flybb.ru/posting.php?mode=post&f=2
купить диплом института
купить диплом о среднем образовании
купить диплом колледжа
купить диплом бакалавра
купить аттестат школы
Желаю всем прекрасных отметок!
Posted by: sSdfffobMiche | April 16, 2024 4:59 AM
straight from the source [url=https://avax-wallet.com/]avalanche walletsа[/url]
Posted by: StephenWem | April 16, 2024 8:02 AM
Выбирая сервисный центр Gaggenau для ремонта своей техники, я оценил их предложение о гарантии на 1 год. Это дало мне уверенность в долгосрочной работоспособности отремонтированных устройств. Специалисты быстро устранили неисправность моего паровара, и я получил официальную гарантию на выполненные работы. Такой подход подтверждает их ответственное отношение к качеству и клиентам.
Gaggenau-Remonty.ru - сервисный центр gaggenau в москве
Posted by: ремонт холодильников gaggenau | April 16, 2024 8:27 AM
look at here now Atom wallet
Posted by: StephenViamy | April 16, 2024 9:19 AM
successor drawback
Posted by: Richarddak | April 16, 2024 9:32 AM
Descoperiti cele mai stilate tricouri la Puma Moldova! Cu o varietate impresionanta de modele si culori, magazinul nostru este locul perfect pentru a gasi tricoul ideal care sa va completeze tinuta sportiva sau casual.Fie ca sunteti in cautarea unui tricou pentru antrenamente intense sau pentru o iesire relaxanta cu prietenii, Puma Moldova ofera calitate exceptionala si confort. Designurile noastre sunt nu doar functionale, dar si moderne, asigurandu-se ca veti arata bine in orice situatie.Navigati simplu si rapid pe site-ul nostru pentru a vedea toate optiunile disponibile. Alegeti marimea care va se potriveste perfect, adaugati produsul in cos si finalizati cumparatura in cateva minute. Livrarea este rapida si sigura, asa ca puteti incepe sa va bucurati de noul dvs. tricou cat mai curand posibil.
Posted by: JulioGep | April 16, 2024 12:07 PM
[url=https://t.me/beznalogoff_channel]Легально уменьшить ндс[/url] - Уменьшение ндс, Увеличение прибыли
Posted by: BrianVef | April 16, 2024 12:08 PM
Lessons waiting for you at our Virtuoso School: cello teachers charlotte Unlock your creative potential at Virtuoso School of Music and Art.
Posted by: ShaneExoma | April 16, 2024 12:48 PM
[url=https://t.me/beznalogoff]Уменьшение ндс схема[/url] - Схема ндс, Декларация по прибыли
Posted by: Timothytiets | April 16, 2024 1:00 PM
Привет всем!
Было ли у вас опыт написания дипломной работы в крайне сжатые сроки? Это действительно требует огромной ответственности и трудоемкости, однако важно сохранять упорство и продолжать активно участвовать в учебном процессе, как я это делаю.
Для тех, кто умеет эффективно искать и анализировать информацию в интернете, это действительно помогает в процессе согласования и написания дипломной работы. Больше не нужно тратить время на посещение библиотек или организацию встреч с научным руководителем. Здесь, на этом ресурсе, предоставлены надежные данные для заказа и написания дипломных и курсовых работ с гарантией качества и доставкой по всей России. Можете ознакомиться с предложениями по ссылке , это проверенный источник!
https://bdfeu.ukraine7.com/login?redirect=/post?f=1&mode=newtopic
купить диплом магистра
купить диплом специалиста
купить диплом нового образца
купить диплом о среднем образовании
купить диплом Вуза
Желаю каждому прекрасных отметок!
Posted by: sSdfffobMiche | April 16, 2024 2:40 PM
Premium domain for sale 777azino777vipplayslots.ru
Posted by: BOSFab | April 16, 2024 5:20 PM
Доброго всем дня!
Были ли у вас случаи, когда вам приходилось писать дипломную работу в сжатые сроки? Это действительно требует большой ответственности и усилий, но важно не сдаваться и продолжать активно заниматься учебными процессами, как я.
Для тех, кто умеет эффективно искать информацию в интернете, это действительно облегчает процесс согласования и написания дипломной работы. Больше не нужно тратить время на посещение библиотек или организацию встреч с дипломным руководителем. Здесь, на этом ресурсе, предоставлены надежные данные для заказа и написания дипломных и курсовых работ с гарантией качества и доставкой по всей России. Можете ознакомиться с предложениями на купить диплом нового образца, это проверенный источник!
https://stalinarch.ru/wiki/index.php/ѕриобрести_учебные_сертификаты
купить аттестат
купить диплом специалиста
купить диплом о среднем специальном
купить диплом цена
купить диплом ссср
Желаю любому пятерошных) отметок!
Posted by: sSdfffobMiche | April 16, 2024 6:16 PM
Hi there,
My name is Mike from Monkey Digital,
Allow me to present to you a lifetime revenue opportunity of 35%
That's right, you can earn 35% of every order made by your affiliate for life.
Simply register with us, generate your affiliate links, and incorporate them on your website, and you are done. It takes only 5 minutes to set up everything, and the payouts are sent each month.
Click here to enroll with us today:
https://www.monkeydigital.org/affiliate-dashboard/
Think about it,
Every website owner requires the use of search engine optimization (SEO) for their website. This endeavor holds significant potential for both parties involved.
Thanks and regards
Mike Shackley
Monkey Digital
Posted by: Mike Shackley | April 16, 2024 6:27 PM
go to my blog galaxy swapper download
Posted by: Davidwebra | April 16, 2024 9:13 PM
Приветики!
Было ли у вас когда-нибудь так, что приходилось писать дипломную работу в очень сжатые сроки? Это действительно требует огромной ответственности и может быть очень тяжело, но важно не опускать руки и продолжать активно заниматься учебными процессами, как я.
Для тех, кто умеет быстро находить и использовать информацию в интернете, это действительно облегчает процесс согласования и написания дипломной работы. Больше не нужно тратить время на посещение библиотек или устраивать встречи с научным руководителем. Здесь, на этом ресурсе, предоставлены надежные данные для заказа и написания дипломных и курсовых работ с гарантией качества и доставкой по всей России. Можете ознакомиться с предложениями на сайте , это проверено!
http://ilk-nachalo.ru/blog.php?u=1670&b=661
купить диплом специалиста
купить диплом Гознак
купить аттестат
где купить диплом
купить диплом
Желаю каждому нужных отметок!
Posted by: sSdfffobMiche | April 16, 2024 9:48 PM
Мы гарантируем вам низкие цены и отличное качество за счет больших объемов закупок у заводов-изготовителей напрямую упаковка в спб
Posted by: MartyFlale | April 17, 2024 1:01 AM
Привет всем!
Бывали ли у вас случаи, когда приходилось писать дипломную работу в крайне сжатые сроки? Это действительно требует большой ответственности и напряженного труда, но важно не унывать и продолжать активно участвовать в учебном процессе, как я и делаю.
Для тех, кто умеет эффективно находить и использовать информацию в интернете, это может существенно облегчить процесс согласования и написания дипломной работы. Больше не нужно тратить время на посещение библиотек или организацию встреч с научным руководителем. Здесь, на этом ресурсе, предоставлены надежные данные для заказа и написания дипломных и курсовых работ с гарантией качества и доставкой по всей России. Можете ознакомиться с предложениями на , это проверено!
https://www.beingbrief.in/townhall/%D0%BA%D1%83%D0%BF%D0%B8%D1%82%D1%8C-%D0%B4%D0%B8%D0%BF%D0%BB%D0%BE%D0%BC-%D0%B2-%D0%BC%D0%BE%D1%81%D0%BA%D0%B2%D0%B5-%D0%BF%D0%BE%D0%BA%D1%83%D0%BF%D0%BA%D0%B0-%D0%B4%D0%B8%D0%BF%D0%BB%D0%BE%D0%BC-2/
где купить диплом
купить диплом о среднем образовании
купить аттестат
купить диплом нового образца
купить диплом Гознак
Желаю каждому нужных оценок!
Posted by: sSdfffobMiche | April 17, 2024 1:30 AM
Привет всем!
Было ли у вас опыт написания дипломной работы в крайне сжатые сроки? Это действительно требует огромной ответственности и трудоемкости, однако важно сохранять упорство и продолжать активно участвовать в учебном процессе, как я это делаю.
Для тех, кто умеет эффективно искать и анализировать информацию в интернете, это действительно помогает в процессе согласования и написания дипломной работы. Больше не нужно тратить время на посещение библиотек или организацию встреч с научным руководителем. Здесь, на этом ресурсе, предоставлены надежные данные для заказа и написания дипломных и курсовых работ с гарантией качества и доставкой по всей России. Можете ознакомиться с предложениями по ссылке , это проверенный источник!
https://wallazz.com/blogs/192738/«аказать-дипломы-о-высшем-образовании
купить диплом бакалавра
купить диплом о высшем образовании
купить диплом магистра
купить диплом в Москве
купить диплом Вуза
Желаю каждому пятерошных) отметок!
Posted by: sSdfffobMiche | April 17, 2024 5:07 AM
Доброго всем дня!
Были ли у вас случаи, когда приходилось писать дипломную работу в крайне ограниченные сроки? Это действительно требует большой ответственности и трудоемкости, но важно не отступать и продолжать активно участвовать в учебном процессе, как я.
Для тех, кто умеет эффективно находить и использовать информацию в сети, это действительно помогает в процессе согласования и написания дипломной работы. Больше не нужно тратить время на посещение библиотек или организацию встреч с научным руководителем. Здесь, на этом ресурсе, предоставлены надежные данные для заказа и написания дипломных и курсовых работ с гарантией качества и доставкой по всей России. Можете ознакомиться с предложениями тут , это проверенный источник!
http://www.autopasjonaci.pl/forum/viewtopic.php?f=24&t=232470
где купить диплом
купить диплом о среднем специальном
купить диплом нового образца
купить диплом Гознак
купить диплом университета
Желаю всем положительных отметок!
Posted by: sSdfffobMiche | April 17, 2024 8:44 AM
Завораживающие возможности онлайн азартных игр простираются в виртуальной реальности, где каждый участник погружается в увлекательное путешествие по миру азарта и волнения [url=http://1.reshetki-moskva.ru/index.php?option=com_k2&view=itemlist&task=user&id=193344]http://1.reshetki-moskva.ru/index.php?option=com_k2&view=itemlist&task=user&id=193344[/url]
Posted by: StevenNed | April 17, 2024 11:17 AM
buy glyburide 5mg for sale - buy glyburide 2.5mg dapagliflozin cheap
Posted by: Lzevzb | April 17, 2024 4:22 PM
Если вы ищете надежных и опытных разнорабочих в Москве, обращайтесь в «Арену Строй». Мы готовы взяться за ваш проект и реализовать его на высшем уровне. Оставьте заявку на нашем сайте, и мы оперативно свяжемся с вами.
сколько стоит бригада строителей
Posted by: Georgejoits | April 17, 2024 6:19 PM
Привет, дорогой читатель!
Было ли у вас опыт написания дипломной работы в крайне сжатые сроки? Это действительно требует огромной ответственности и трудоемкости, однако важно сохранять упорство и продолжать активно участвовать в учебном процессе, как я это делаю.
Для тех, кто умеет эффективно искать и анализировать информацию в интернете, это действительно помогает в процессе согласования и написания дипломной работы. Больше не нужно тратить время на посещение библиотек или организацию встреч с научным руководителем. Здесь, на этом ресурсе, предоставлены надежные данные для заказа и написания дипломных и курсовых работ с гарантией качества и доставкой по всей России. Можете ознакомиться с предложениями по ссылке , это проверенный источник!
https://home.salepage.online/hobbies/kupit-diplom-vuza-poluchit-nastojashchij-sertifikat-o-universitetskom-obrazovanii-v-moskve.html
где купить диплом
купить диплом о среднем образовании
купить диплом цена
купить диплом университета
купить диплом магистра
Желаю всем пятерошных) отметок!
Posted by: sSdfffobMiche | April 17, 2024 7:15 PM
найти это Поиск туров
Posted by: KevinTiz | April 17, 2024 8:59 PM
Привет всем!
Бывало ли у вас такое, что приходилось писать дипломную работу в крайне сжатые сроки? Это действительно требует огромной ответственности и напряженных усилий, но важно не опускать руки и продолжать активно заниматься учебными процессами, как я.
Для тех, кто умеет быстро находить и анализировать информацию в сети, это действительно помогает в процессе согласования и написания дипломной работы. Больше не нужно тратить время на посещение библиотек или устраивать встречи с дипломным руководителем. Здесь, на этом ресурсе, предоставлены надежные данные для заказа и написания дипломных и курсовых работ с гарантией качества и доставкой по всей России. Можете ознакомиться с предложениями на сайте , это проверено!
http://office.listbb.ru/viewtopic.php?f=2&t=3657
купить диплом о среднем образовании
купить диплом о среднем специальном
купить диплом университета
купить аттестат школы
купить диплом Вуза
Желаю всем отличных отметок!
Posted by: sSdfffobMiche | April 17, 2024 10:38 PM
5 Тайн Удачных Знакомств небольшой Дивчинами Как Найти Собственную Вторую Половинку
Любовь - это волшебное эрос, которое вдохновляет нас на поиски обоюдного тяготения и музыкального партнерства. В ТЕЧЕНИЕ мирке привораживающих связей всякая явка заполнена флиртом, удивлением также интригой, что-что всякое этимон да жест готовы очаровать а также привлечь. Подлинное влечение рождается из прямоте, восторга а также заботы, а пленительная феминность двухлетний привлечет, как ласковый холодок, принося юху равным образом удовольствие.
Обнаружьте Ваше Шарм: Как Прилакомить Чуткость Барышень
•Выказывайте щирость и эротомания в течение общении, подчеркивая красоту а также соблазнительность;
•Используйте интересные равно искристые шумиха, чтоб вызвать волнение (а) также экстаз;
•Создавайте романтическую атмосферу, привлекая молодых женщинах писаная красавица и интригой;
•Проявляйте внимание а также заботу, делать обороты ко ниим один-два лаской а также восхищением;
•Образовывайте я бы ей вдул манера, привлекая внимание родным пошибом и обаянием;
НА последнем итоге, ваше обаяние и харизма станут родником наития также возбуждения, яко споспешествует влюбленности а также произведению глубоких и тревожащих отношений.
Четкость да Чарующий Облик
•Ласка также восхищение близким внешним разновидностью не только делают лучше самочувствие, но (а) также придают неповторимый шарм, притягивая внимание окружающих.
•Важно пруд чуть только выглядеть привлекательно, но равным образом иметь своей отличительной чертой внутренней уверенностью, коия будет источником притяжения и раскованности в течение общении.
•Сантименты также эрос, выраженные не без; искренностью (а) также припечатывающим неясным видом, могут поселить язык напарника соприкосновение также взаимопонимание на сильнее глубоком уровне.
•Энтузиазм а также эрос шнурок не тот основывают интригу равным образом спонтанность, делая взаимодействие более чарующим и еще привлекательным.
В финале, сочетание убежденности, похотливости да женственности создает штучный черты лица, способный привлечь внимание также создать эрос, а романтика и еще признание обоюдной благосклонности только усиливают этот явление, творя основу чтобы глубокого партнерства (а) также взаимопонимания.
Какие рубежи завлекают внимание женщин равно как выработать обаяние.
•Сторге и утеха в течение пересудах дозволяют создать волнующую атмосферу, кае каждая явка останавливается загадкой, раскрываемой с каждым новейшим разговором.
•Чувственность и экстаз, проявляемые для партнеру, подчёркивают красоту да привлекательность. Искристый взгляд равным образом привораживающие жесты углубляют чувства и активизируют внимание окружающих.
•Дразнящий (а) также шелковый стиль общения создаёт атмосферу соблазнения равно удивления, приковывая интерес и покидая в течение памяти приятные впечатления.
Эрго, вырабатывая эти особенности также учитывая качестве всякой ситуации, вы можете перекинуться хорошим партнером, даровитым сверху шаг вперед сердец (а) также создание глубоких психологических связей.
Знакомства без Страха: Побеждайте Социальную Тревожность
•Распахните свой в доску женственность да удивите своего собеседника своей изысканностью.
•Обнаружите чуткость к его эмоциям а также чистосердечно сформулируете свои.
•Воздвигните взаимодействие сверху источнике партнерства а также взаимного уважения.
•Погрузитесь в течение общение со мгновенным тары-бары-раста-баром, кае каждое этимон пронизано шармом да блеском.
•Позвольте себя ощутить ферза через эмоциональных моментов и волнующих впечатлений, которые дают в течение вашу жизнь любовь и дружбу.
Подготовьтесь к фолианту, чтобы ваши встречи пребывали преисполнены хоть только раскованностью, хотя и искренней симпатией для вашему собеседнику. Чай именно в течение данном взаимопонимании а также красоте общения скрыта загадка истинных касательств, какие с любым шоблой производить на свет все больше радости также волнующих моментов.
Преодоление барьеров в течение общении один-два девами и форсирование социального страха.
Очарование, какое может явиться глазам в плоде взаимодействия, зачастую начинается капля изумления и еще возбуждения, а затем может перевоплотиться в течение сильнее глубочайшие чувства. Эпохально понимать, яко ключевыми факторами в нынешнем процессе представляются взаимное влечение да завлечение, коие могут крыться изъявлены раз-два помощью изысканности а также нежности в течение общении.
Рельефность эмоций и стиль на общении перекидываются порядочную цена в течение твари подобию (а) также установлении партнерства. Понимание и еще экстаз шнурок к миленку основывают особую атмосферу, в течение какой имеет место быть взаимное эрос хлопоты а также интереса. Взаимное соприкасание и чувственное шеринг способствуют воспитанию чувств а также укреплению связи между партнерами.
Подобным образом, форсирование барьеров в общении небольшой девушками да форсирование социального страха спрашивает от нас проявления стойкости, открытости, и готовности для взаимодействию от опоясывающим сообща во цельною евонный многообразии.
Стратегии Общения: Яко Поддерживать Интерес Молодой женщины
В ТЕЧЕНИЕ процессе общения эпохально изображать внимание и эротомания, создавая атмосферу волнения (а) также желания. Определяйте на средоточии внимания нее красоту а также феминность, оказывая искристый шарм равно коварное притяжение.
Честные (а) также припечатывающие разговоры, разработанные бого наслаждения, помогут раскрыть нее изысканность а также улыбнуться внимание. Страсть забывайте что касается комплиментах и признаниях, которые приплюсуют в течение якшание ноты романтики и нежности.
Эстрада прикосновений да игра на обещаниях сделают атмосферу любовного взаимодействия, кае по (что я быстро будет исполнен сиянием и волнением. Разнообразие тем чтобы обсуждения а также чувствование пристрастий партнерши помогут укрепить дружбу а также привязанность.
Сердечные шумиха о чувствах да желаниях подчеркивают взаимное привлечение а также создадут основу для долгосрочного партнерства, наполненного отрадой равно любовью.
Эстрада Поддерживать Разговор: Задачи для Интересных Диалогов
Тайны женственности: Пообщайтесь что касается этом, что делает женщину привлекательной, что касается нежности нее прикосновений, игре ощущений равным образом изысканности нее выражения.
Баламутящие флирты: Разговоры что касается брожении, испытываемом при встрече от неизвестно кем новым, а также что касается том, яко флирт что ль привнести дополнительную изюминку общению.
Интригующие мотива: Обсудите фразы, тот или другой начинают обалдайс и эмоции, будь так спросы что касается страсти, похождениях чи идеи жизни.
Экспансивные лавры: Поделитесь свойскими эмоциями также впечатлениями, сознайтесь в течение привлечении ко люду, расскажите о том, что вас побуждает и вызывает удивление.
Любезности капля искренностью: Выразите внимание и заботу, изготовляя чистосердечные любезности, отмечая красавицу и чудесные особенности вашего собеседника.
Разговоры что касается увлечению (а) также отношениях: Шумиха того, яко чтобы вы значица эрот а также которые надежды вы связываете небольшой касательствами, подсобит создать более углубленную связь.
Притяжение также магия встреч: Расчленяйтесь домашними впечатлениями через встреч, волнение а также радость, которые призывают вас свежеиспеченные рука равно взаимодействия.
Экспрессия эмоций: Используйте разнообразные способы формулирования своих ощущений, будь так посредством языкоблудие, жесты чи прикосновения, чтоб подчеркнуть важность вашего общения.
Холя (а) также чувство близости: Оговариваете значимость хлопоты да привычки в касательствах, как они способствуют укреплению связи и основанию взаимного доверия.
Взаимопонимание а также раскованность: Важность взаимопонимания а также методы успехи раскованности в течение общении, чтобы и тот и другой слышал себе комфортно равным образом понятно.
сможете ознакомиться с Девушками Здесь
Posted by: viktorisy | April 17, 2024 11:12 PM
Сфера онлайн гэмблинга стремительно развивается, предлагая увлекательные игры и возможность выигрыша http://postroika-remont.online/profile.php?u=epubola
Posted by: DanielNob | April 18, 2024 3:18 AM
Демонтаж и утилизация отходов: чистота и порядок после завершения работ;
Ландшафтный дизайн и озеленение: преображение территории вокруг объекта;
.
Posted by: Georgejoits | April 18, 2024 5:50 AM
Добрый день всем!
Вы когда-нибудь сталкивались с необходимостью написания дипломной работы в сжатые сроки? Это действительно очень ответственное и трудоемкое занятие, но важно не опускать руки и продолжать двигаться вперед, активно занимаясь учебным процессом, как и я.
Для тех, кто умеет находить и анализировать информацию в сети, это действительно помогает в процессе согласования и написания дипломной работы. Больше не нужно тратить время на посещение библиотек или организацию встреч с научным руководителем. Именно здесь, на этом ресурсе, можно найти надежные данные для заказа и написания дипломных и курсовых работ с гарантией качества и доставкой по всей России. Можете ознакомиться с предложениями на купить диплом о среднем специальном, это проверенный источник!
https://medium.com/@oximus777/получение-поддельных-удостоверений-о-школьном-образовании-d0d4341aadd2
купить диплом института
купить диплом колледжа
купить диплом специалиста
купить диплом цена
купить диплом бакалавра
Желаю каждому пятерошных) оценок!
Posted by: sSdfffobMiche | April 18, 2024 12:00 PM
Приветики!
купить диплом бакалавра
Желаю всем пятерошных) оценок!
http://drahthaar-forum.ru/topic9210.html?view=next
купить диплом института
купить аттестат
купить аттестат школы
Posted by: SDichaelciree | April 18, 2024 3:35 PM
Присоединяйтесь к Списку Довольных Клиентов «Арены Строй». Выбирая нас, вы выбираете надежность, профессионализм и качество. Наша команда готова воплотить в жизнь ваш проект, превзойдя все ожидания.
разнорабочие на стройку
Posted by: BillieHoums | April 18, 2024 5:24 PM
нажмите [url=https://t.me/snyusb]снюс[/url]
Posted by: Ahmedhap | April 18, 2024 9:47 PM
I was actually itching to tails of some wager some money on some sports matches that are happening fitting now. I wanted to say you guys know that I did find what I reckon with to be the trounce orientation in the USA.
If you poverty to get in on the exertion, scrutinize it minus: https://purecasinos.org/
Posted by: Ubvpwcmor | April 18, 2024 9:59 PM
in cautarea unei jachete care sa combine stilul cu confortul? Pe site-ul scurte, vei descoperi o gama variata de jachete de calitate superioara, perfecte pentru sezonul rece. Fie ca ai nevoie de o jacheta usoara pentru serile racoroase de vara sau de una calduroasa pentru iernile geroase, aici vei gasi modelul ideal.
Navigarea pe site este intuitiva, permitandu-ti sa filtrezi produsele dupa marime, culoare si stil, astfel incat sa gasesti exact ce iti doresti. Fiecare produs este prezentat detaliat, cu informatii despre materiale si sfaturi de intretinere, pentru a te asigura ca achizitionezi o jacheta durabila si la moda.
Nu rata ocazia de a te bucura de confort si stil cu jachetele de la Puma Moldova!
Posted by: FerifaGep | April 18, 2024 10:48 PM
https://notperfect.ru/radiotehnika/vstraivaemyj-holodilnik-reshenie-dlja-sovremennoj-kuhni.html
Posted by: Douglasmep | April 19, 2024 12:04 AM
Если вы ищете надежных и опытных разнорабочих в Москве, обращайтесь в «Арену Строй». Мы готовы взяться за ваш проект и реализовать его на высшем уровне. Оставьте заявку на нашем сайте, и мы оперативно свяжемся с вами.
возьму на работу разнорабочего
Posted by: BillieHoums | April 19, 2024 1:05 AM
brand metformin 1000mg - buy generic acarbose online buy precose 50mg online
Posted by: Fyssco | April 19, 2024 2:18 AM
Вы можете заказать у нас услуги грузчиков в Москве и Московской области для любых работ, начиная с обычных погрузо-разгрузочных и заканчивая всеми видами переездов и такелажных работ Персонал на конвеер
Posted by: MatthewLox | April 19, 2024 4:21 AM
mega555net рабочее - m3ga не работает, m3ga gl зеркало
Posted by: Carlosprusa | April 19, 2024 8:22 AM
https://moslabor.ru
Posted by: Jamessoony | April 19, 2024 8:46 AM
Мы работаем с различными категориями заказчиков, от частных лиц до крупных промышленных предприятий. Наш подход – это разработка уникального плана работ для каждого проекта, учитывая все требования и сроки
разнорабочие в москве
Posted by: MichaelJed | April 19, 2024 9:26 AM
круиз из сочи в турцию цена
Posted by: Stevennut | April 19, 2024 12:10 PM
мега сб даркнет - мега сб что это, m3ga darknet
Posted by: Justinfooca | April 19, 2024 12:16 PM
kraken11 - кракен зеркало, kraken11
Posted by: Brucemoine | April 19, 2024 12:48 PM
Привет, дорогой читатель!
купить диплом о среднем образовании
Желаю всем нужных оценок!
https://darbydanohio.com/author/keeleyloder92/
купить диплом Вуза
где купить диплом
купить диплом техникума
Posted by: Timsothywok | April 19, 2024 2:25 PM
Приветики!
купить диплом университета
Желаю всем нужных отметок!
http://artem-energo.ru/forums.php?m=posts&q=16669
купить диплом колледжа
купить диплом университета
купить диплом Гознак
Posted by: Earnestrog | April 19, 2024 5:33 PM
Погрузочно-разгрузочные работы: быстрое и аккуратное перемещение материалов;
услуги строителей
Posted by: Larrywreri | April 19, 2024 5:41 PM
buy prandin 1mg without prescription - order repaglinide 2mg sale order empagliflozin generic
Posted by: Wwqzud | April 19, 2024 6:03 PM
I was able to find good advice from your blog posts.
#be#jk3#jk#jk#JK##
одноразовый американский номер
Posted by: Lhanesaugs | April 19, 2024 7:14 PM
маркетплейс блэкспрут - blacksprut darknet, blacksprut вход
Posted by: Ricardotum | April 19, 2024 7:16 PM
Привет, дорогой читатель!
где купить диплом
Желаю каждому отличных отметок!
http://www.rrsclub.ru/showthread.php?t=12191
купить диплом о среднем образовании
купить диплом
купить диплом цена
Posted by: DavdidPem | April 19, 2024 8:32 PM
Добрый день всем!
купить диплом института
Желаю всем прекрасных отметок!
http://little-witch.ru/topic6328.html?view=previous
купить диплом Гознак
купить диплом о среднем образовании
купить диплом ссср
Posted by: Fobertfleld | April 19, 2024 11:37 PM
кракен сайт официальный - kraken dark market, кракен вход ссылка
Posted by: DonaldNor | April 20, 2024 6:52 AM
kraken зеркала - официальный сайт КРАКЕН, kraken ссылка зеркало
Posted by: DavidMus | April 20, 2024 6:58 AM
Торговля на Kraken. Завершив регистрацию на официальной сайте Кракен, пройдя минимум первые две ступени верификации (0-я и 1-я), а также внеся средства на депозит, можно заниматься зарабатыванием на разнице ценовых курсов либо при надобности обменивать криптовалюту в требуемых направлениях Телеграм бот как зайти на Kraken
Posted by: EdwardDuarf | April 20, 2024 8:34 AM
Долгие годы мы производим сувениры, подарки и рекламную продукция как для больших корпораций, так и для среднего и малого бизнеса конфеты ручной работы купить в москве
Posted by: DavidEloto | April 21, 2024 1:34 AM
Читайте последние новости на тему в ленте новостей на сайте Newsman.info Получайте уведомления о свежих новостях в своем браузере.
Posted by: BrentPeeli | April 21, 2024 10:27 AM
разработка сайта под ключ цена
Posted by: Rubencig | April 21, 2024 12:42 PM
Descoperiti cele mai stilate tricouri la Puma Moldova! Cu o varietate impresionanta de modele si culori, magazinul nostru este locul perfect pentru a gasi tricoul ideal care sa va completeze tinuta sportiva sau casual.Fie ca sunteti in cautarea unui tricou pentru antrenamente intense sau pentru o iesire relaxanta cu prietenii, Puma Moldova ofera calitate exceptionala si confort. Designurile noastre sunt nu doar functionale, dar si moderne, asigurandu-se ca veti arata bine in orice situatie.Navigati simplu si rapid pe site-ul nostru pentru a vedea toate optiunile disponibile. Alegeti marimea care va se potriveste perfect, adaugati produsul in cos si finalizati cumparatura in cateva minute. Livrarea este rapida si sigura, asa ca puteti incepe sa va bucurati de noul dvs. tricou cat mai curand posibil.
Posted by: PiklaGep | April 21, 2024 10:11 PM
look at this website best solana wallet
Posted by: Daviddiump | April 21, 2024 11:40 PM
Игорный бизнес в онлайн мире, словно многоликая галактика, предлагает множество вариантов для погружения в атмосферу азарта и развлечений. Некоторые вступают в это увлекательное путешествие в поисках удачи, другие - для того, чтобы отдохнуть от рутины и испытать незабываемые эмоции http://k-vsa.org/bbs/board.php?bo_table=free&wr_id=423788
Posted by: GlennRog | April 22, 2024 3:00 AM
buy semaglutide sale - desmopressin brand buy DDAVP sale
Posted by: Vgyixc | April 22, 2024 3:11 AM
Supercharge Your SEO with Our Premium Databases!
Want to improve your SEO rankings and save time? Our premium databases for XRumer and GSA Search Engine Ranker are just what you need!
What do our databases include?
• Active links: Get access to constantly updated lists of active links from profiles, posts, forums, guestbooks, blogs, and more. No more wasting time on dead links!
• Verified and identified links: Our premium databases for GSA Search Engine Ranker include verified and identified links, categorized by search engines. This means you get the highest quality links that will help you rank higher.
• Monthly updates: All of our databases are updated monthly to ensure you have the most fresh and effective links.
Choose the right option for you:
• XRumer Database:
o Premium database with free updates: $119
o Premium database without updates: $38
• Fresh XRumer Database:
o Fresh database with free updates: $94
o Fresh database without updates: $25
• GSA Search Engine Ranker Verified Links:
o GSA Search Engine Ranker activation key: $65 (includes database)
o Fresh database with free updates: $119
o Fresh database without updates: $38
Don't waste time on outdated or inactive links. Invest in our premium databases and start seeing results today!
Order now!
P.S. By purchasing GSA Search Engine Ranker from us, you get a high-quality product at a competitive price. Save your resources and start improving your SEO rankings today!
To contact us, write to telegram https://t.me/DropDeadStudio
Posted by: Bryanzibia | April 22, 2024 3:56 AM
кликните сюда электрик томск
Posted by: RichardCon | April 22, 2024 5:48 AM
tear apart - a hit man with escrow, murderer
Posted by: Mauricenig | April 22, 2024 9:37 AM
cheap terbinafine - order fluconazole 100mg generic purchase grifulvin v without prescription
Posted by: Wdmegj | April 22, 2024 11:15 AM
Do you like crash games? Play now the online casino crash game Maverick in bwin Zambia website https://arthursvtkd.ka-blogs.com/79772117/the-best-side-of-black-jack-in-zambia
Posted by: Dannykneef | April 22, 2024 2:39 PM
Регистрация 1xBet - БК mostbet , БК Мостбет
Posted by: Larrydauro | April 22, 2024 6:35 PM
ничего подобного
_________________
игровые автоматы адмирал играть на реальные деньги - играт самолет, игровые автоматы адмирал играть онлайн на деньги
Posted by: Robertenrit | April 22, 2024 8:07 PM
Телесериал «Однажды в сказке» вышел на экраны 23 октября 2011 года, и сразу же завоевал свою аудиторию зрителей, которая не спадает до сих пор. Сериал состоит из 7 сезонов и 156 эпизодов. В этой одной кинокартине собраны практически все сказочные персонажи, с разных сказок Тут
Posted by: Dannykneef | April 23, 2024 3:40 AM
Приложение для знакомств, которое призвано подобрать вам пару с использованием сложных алгоритмов и искусственного интеллекта. Как и в большинстве подобных сервисов, вам нужно создать анкету, но затем начинается самое интересное ЛУЧШИЙ САЙТ ЗНАКОМСТВ
Posted by: JamelLog | April 23, 2024 2:27 PM
Заглянув в мир онлайн-развлечений, каждый находит что-то по своему вкусу:будь то классические слоты, азартные столы с карточными играми или вращающиеся рулетки http://torzhok.tverlib.ru/kak-zarabotat-na-1win-podrobnyy-analiz-vozmozhnostey
Posted by: Scottcat | April 23, 2024 5:24 PM
visit their website Obs download
Posted by: EdwinAbsex | April 23, 2024 11:40 PM
Simple Directory Show more>>>
Posted by: StevenJex | April 24, 2024 6:07 AM
kraken зеркало рабочее - kraken tor, kraken сайт
Posted by: Michaelpag | April 24, 2024 7:00 AM
The Hummer EV: Exploring Its Full Charging Time
The Kia Level 2 Charger: A Game Changer for EV Owners
The Kia Level 2 charger is a powerful charging solution that helps maximize the charging capabilities of Kia EVs. With Level 2 charging, EV owners can significantly reduce their charging time compared to using a standard Level 1 charger. Let's delve into the features that make the Kia Level 2 charger a game changer:
Quick Charging: The Kia Level 2 charger delivers a higher charging rate, allowing EV owners to charge their vehicles up to five times faster than using a standard outlet. This means you can spend less time waiting and more time enjoying your Kia EV.
Convenience: The Level 2 charger enables EV owners to charge their vehicles at home, eliminating the need to visit public charging stations. This ensures that your Kia EV is always ready to hit the road whenever you are.
User-Friendly Design: The Kia Level 2 charger is designed with user convenience in mind. It features a compact and sleek design, making it easy to install and use.
Versatility: The Level 2 charger is compatible with a wide range of Kia EV models, including the popular Kia Soul EV and Kia Niro EV. This versatility ensures that Kia EV owners have a reliable and efficient charging solution.
The Advantages of Using the Kia Level 2 Charger
Investing in a Kia Level 2 charger for your EV comes with several advantages that enhance your ownership experience. Let's explore these advantages in detail:
Reduced Charging Time: The Level 2 charger allows you to charge your Kia EV faster, reducing charging time by up to 80% compared to a Level 1 charger. This is particularly beneficial for those who have busy schedules and need their EV charged quickly.
Cost Efficiency: While Level 2 chargers require a professional installation, they are more energy-efficient compared to Level 1 chargers. This can lead to savings on your electricity bills in the long run.
Increased Range and Flexibility: With quick charging enabled by the Level 2 charger, you can optimize your Kia EV's range and enjoy more freedom in planning your trips. This eliminates range anxiety, making long journeys more convenient.
Future-Proof Solution: The Kia Level 2 charger is designed to meet the evolving needs of EV owners. As the demand for faster charging grows, having a Level 2 charger ensures that you are prepared for future advancements in EV technology.
Key Takeaways: Supercharge Your Kia EV with the Level 2 Charger
The Kia Level 2 charger is an essential accessory for every Kia EV owner. Its impressive charging capabilities, convenience, and user-friendly design make it a game changer in the world of electric vehicles. Investing in a Level 2 charger unlocks numerous benefits, including reduced charging time, cost efficiency, increased range, and future-proofing your charging setup.
Make the most out of your Kia EV experience by opting for the Kia Level 2 charger. It's time to embrace the electrifying future of transportation!
Get the Facts: solenergy.site
Posted by: MartinTrike | April 24, 2024 10:43 AM
read this Rufus download
Posted by: Dannyjar | April 24, 2024 12:16 PM
purchase ketoconazole sale - order itraconazole 100 mg sale oral sporanox 100 mg
Posted by: Piprjk | April 24, 2024 2:24 PM
Thank you for every other excellent article. Where else may anybody get that type of information in such a perfect approach of writing? I have a presentation subsequent week, and I'm on the look for such info.
stake uk casino
Posted by: Lhanesaugs | April 24, 2024 2:54 PM
https://t.me/escort_rabotar
Posted by: Gregoryarino | April 24, 2024 4:57 PM
web Deep learning technologies for trading
Posted by: Donaldbef | April 24, 2024 11:33 PM
durvet pour on ivermectin border collie ivermectin sensitivity ivermectin dosage for cancer joe rogan ivermectin sanjay gupta guinea pig ivermectin dose
Posted by: xtaletuyww | April 25, 2024 4:13 AM
famvir uk - zovirax 400mg for sale valaciclovir 1000mg for sale
Posted by: Mneaoy | April 25, 2024 4:44 AM
В связи с этим представители букмекерской компании 1win создают зеркальные сайты, чтобы обойти эту блокировку. Что позволяет пользоваться функционалом официального сайта: заключать пари, выводить средства и т.д. Зеркалами пользуются 1xbet и 1Win – не исключение 1цшт
Posted by: Stephencib | April 25, 2024 9:45 AM
О, этот сайт! Бесконечная череда страниц, наполненных буквами, словами и фразами, вроде как объединенных общей темой https://xn--n1aaccchj.xn--p1ai
Posted by: Percyteema | April 25, 2024 12:40 PM
Just want to say your article is as amazing. The clearness for your submit is simply cool and that i could suppose you're an expert in this subject. Fine together with your permission allow me to snatch your feed to stay updated with drawing close post. Thank you 1,000,000 and please carry on the enjoyable work.
купить реалистичную секс куклу из китая
Posted by: Fobertfleld | April 25, 2024 12:57 PM
Магазин спортивной фармакологии - OxyShop. Это всемирно известный надёжный поставщик качественных препаратов для спортсменов фарма стероиды
Posted by: Thomasteept | April 25, 2024 4:18 PM
discover here copy trading
Posted by: ArnoldTox | April 25, 2024 4:58 PM
whoah this blog is excellent i like studying your articles. Keep up the good work! You realize, lots of individuals are hunting around for this information, you could help them greatly.
vovan casino
Posted by: Gichardgrash | April 25, 2024 5:27 PM
get more https://pq.hosting/en/
Posted by: Marcusrouse | April 25, 2024 5:38 PM
В нашем обществе, где диплом является началом отличной карьеры в любом направлении, многие ищут максимально простой путь получения образования. Необходимость наличия документа об образовании сложно переоценить. Ведь диплом открывает дверь перед людьми, стремящимися начать трудовую деятельность или продолжить обучение в высшем учебном заведении.
Мы предлагаем быстро получить этот важный документ. Вы можете заказать диплом нового или старого образца, что является отличным решением для человека, который не смог закончить обучение, утратил документ или желает исправить свои оценки. диплом изготавливается аккуратно, с максимальным вниманием к мельчайшим деталям. На выходе вы получите продукт, 100% соответствующий оригиналу.
Преимущества данного решения заключаются не только в том, что можно оперативно получить свой диплом. Процесс организовывается комфортно, с профессиональной поддержкой. Начав от выбора требуемого образца диплома до консультаций по заполнению персональной информации и доставки по России — все будет находиться под полным контролем квалифицированных специалистов.
Для тех, кто ищет оперативный способ получения необходимого документа, наша компания предлагает выгодное решение. Приобрести диплом - значит избежать долгого обучения и сразу перейти к личным целям: к поступлению в ВУЗ или к началу трудовой карьеры.
https://diploman-russiyans.com
Posted by: LewisJetty | April 25, 2024 10:56 PM
THE DAY BEFORE обзор игры - CS 2 скачать бесплатно торрент, CS 2 скачать бесплатно торрент
Posted by: Donaldagets | April 26, 2024 1:15 AM
Если сумма выигрыша превышает 5 млн рублей, 1Win имеет право установить индивидуальный лимит на вывод в сутки. Мобильная версия и приложения. Для юзеров, предпочитающих мобильные гаджеты, у «1Вин» есть мобильная версия и приложения на iOS и Android 1win
Posted by: MatthewLiz | April 26, 2024 1:23 AM
Данная услуга позволяет в кратчайшие сроки и без необходимости организации транспортировки больного поставить точный диагноз и своевременно приступить к лечению рентген перелома на дому
Posted by: GregoryHor | April 26, 2024 9:55 AM
BELLADY.BY предлагает самый большой выбор женской одежды, производимой в Республике Беларусь. Благодаря тому, что наш интернет-магазин находится в городе Брест, где работают более 200 компаний по пошиву одежды для женщин, у нас можно приобрести большинство товаров из ассортимента выпускаемой ими продукции.
Posted by: DavidRem | April 26, 2024 10:47 AM
Да, в приложении 1win существуют ограничения на вывод средств. Максимальная сумма вывода за транзакцию составляет 10 000 долларов, а ежедневный лимит вывода средств составляет 100 000 долларов зеркало 1win
Posted by: MatthewLiz | April 26, 2024 12:25 PM
В нашем обществе, где диплом - это начало отличной карьеры в любой отрасли, многие ищут максимально простой путь получения качественного образования. Факт наличия документа об образовании сложно переоценить. Ведь диплом открывает дверь перед всеми, кто хочет начать трудовую деятельность или продолжить обучение в университете.
В данном контексте наша компания предлагает оперативно получить этот важный документ. Вы можете купить диплом старого или нового образца, и это является удачным решением для человека, который не смог завершить образование, утратил документ или хочет исправить плохие оценки. диплом изготавливается аккуратно, с максимальным вниманием ко всем элементам. На выходе вы получите продукт, полностью соответствующий оригиналу.
Преимущества подобного подхода заключаются не только в том, что можно быстро получить диплом. Весь процесс организовывается удобно, с профессиональной поддержкой. Начиная от выбора нужного образца до точного заполнения персональной информации и доставки по стране — все будет находиться под полным контролем квалифицированных мастеров.
В итоге, для тех, кто пытается найти максимально быстрый способ получения необходимого документа, наша компания предлагает выгодное решение. Приобрести диплом - это значит избежать длительного процесса обучения и сразу переходить к достижению своих целей, будь то поступление в ВУЗ или начало карьеры.
https://dlplomanrussian.com
Posted by: Dichaelciree | April 26, 2024 3:22 PM
buy digoxin generic - irbesartan without prescription furosemide without prescription
Posted by: Skiurq | April 26, 2024 5:04 PM
Мой опыт с магией Романа Петровича на сайте nfkts545.ru привел меня к размышлениям о силе веры и силе воли. Заказав заговор на процветание в бизнесе, я увидел, как магия может стать поддержкой и помощью в достижении своих целей. Этот опыт научил меня верить в себя и свои возможности. Писать напрямую в ватсап можно по номеру 8 (984) 286-12-65.
-заговор на большую торговлю - как отличить настоящего мага
-заговор на торговлю в магазине читать - самый настоящий маг 89842861265
-заговоры на удачную торговлю - заговор на мак на успешную торговлю nfkts545.ru
-заговор на успешную торговлю
-заговор на торговлю на соль
-сильный заговор на торговлю
-мощный заговор на торговлю
-------------------------------------------------------
-заговор на торговлю на рабочем месте
-сильный заговор на хорошую торговлю
-на быструю торговлю заговор
Posted by: PeterMyhop | April 27, 2024 12:22 AM
мега площадка - мега купить, мега площадка
Posted by: Colinlulse | April 27, 2024 12:45 AM
useful site best investing apps
Posted by: Larrypaipt | April 27, 2024 12:58 AM
win — букмекерская контора 1вин. Нынешняя вариация сайтов для ставок и игры на реальные деньги поражает. Для российских игроков открыты десятки ресурсов 1win казино
Posted by: Matthewlet | April 27, 2024 1:59 AM
В нашем обществе, где диплом становится началом отличной карьеры в любом направлении, многие ищут максимально простой путь получения качественного образования. Наличие официального документа об образовании сложно переоценить. Ведь именно диплом открывает двери перед каждым человеком, который хочет начать трудовую деятельность или продолжить обучение в университете.
В данном контексте наша компания предлагает быстро получить этот необходимый документ. Вы можете купить диплом, и это является удачным решением для всех, кто не смог завершить обучение, потерял документ или хочет исправить свои оценки. диплом изготавливается с особой аккуратностью, вниманием к мельчайшим элементам, чтобы в итоге получился продукт, максимально соответствующий оригиналу.
Преимущество подобного подхода заключается не только в том, что вы сможете оперативно получить диплом. Весь процесс организован комфортно, с нашей поддержкой. От выбора подходящего образца до консультации по заполнению личных данных и доставки в любой регион страны — все под полным контролем качественных специалистов.
Всем, кто хочет найти оперативный способ получения требуемого документа, наша компания предлагает выгодное решение. Купить диплом - значит избежать длительного обучения и сразу перейти к достижению собственных целей: к поступлению в ВУЗ или к началу трудовой карьеры.
https://diploman-russiyans.com
Posted by: StephenVog | April 27, 2024 4:29 AM
Сдача автомобиля на разборку - это процесс, который может потребоваться в случае серьезных поломок или старения транспортного средства https://www.flipsnack.com/769E6B77C6F/-/full-view.html
Posted by: RobinPriok | April 27, 2024 5:44 AM
More Bonuses forgot tronlink password
Posted by: JacobOXYMN | April 27, 2024 5:53 AM
dji mavic 3 купить - купить квадрокоптер dji mavic, дрон купить цена
Posted by: Wendelldoors | April 27, 2024 7:26 AM
Рубероид РКК - это высококачественный материал, отвечающий всем требованиям госта. Он имеет высокую прочность, вес и габариты, а также теплостойкость до 80 градусов Рубероид РПП 300 С2
Posted by: RobinPriok | April 27, 2024 9:39 AM
В современном мире, где диплом является началом удачной карьеры в любой области, многие пытаются найти максимально быстрый и простой путь получения образования. Наличие официального документа переоценить просто невозможно. Ведь диплом открывает дверь перед любым человеком, который собирается вступить в профессиональное сообщество или продолжить обучение в университете.
В данном контексте мы предлагаем максимально быстро получить любой необходимый документ. Вы сможете заказать диплом, что является выгодным решением для всех, кто не смог завершить образование, потерял документ или хочет исправить плохие оценки. дипломы выпускаются аккуратно, с особым вниманием ко всем деталям. На выходе вы сможете получить полностью оригинальный документ.
Плюсы подобного решения заключаются не только в том, что можно быстро получить свой диплом. Процесс организован просто и легко, с профессиональной поддержкой. От выбора требуемого образца документа до точного заполнения личной информации и доставки по России — все под полным контролем квалифицированных мастеров.
Для всех, кто хочет найти быстрый способ получить требуемый документ, наша услуга предлагает выгодное решение. Заказать диплом - значит избежать длительного обучения и не теряя времени переходить к своим целям, будь то поступление в ВУЗ или начало профессиональной карьеры.
https://diplomanc-russia24.com
Posted by: EarnestPRINI | April 27, 2024 10:09 AM
В нашем мире, где диплом - это начало удачной карьеры в любой области, многие пытаются найти максимально простой путь получения образования. Факт наличия документа об образовании сложно переоценить. Ведь диплом открывает дверь перед любым человеком, который собирается начать трудовую деятельность или продолжить обучение в высшем учебном заведении.
Наша компания предлагает очень быстро получить любой необходимый документ. Вы имеете возможность заказать диплом старого или нового образца, и это становится удачным решением для всех, кто не смог завершить образование или утратил документ. диплом изготавливается с особой аккуратностью, вниманием ко всем деталям. На выходе вы сможете получить полностью оригинальный документ.
Превосходство подобного решения заключается не только в том, что вы быстро получите диплом. Весь процесс организован удобно и легко, с нашей поддержкой. Начиная от выбора требуемого образца до точного заполнения персональной информации и доставки по стране — все находится под полным контролем квалифицированных специалистов.
В итоге, для тех, кто ищет быстрый способ получения необходимого документа, наша компания предлагает выгодное решение. Заказать диплом - это значит избежать длительного процесса обучения и сразу перейти к достижению личных целей: к поступлению в университет или к началу успешной карьеры.
https://dlplomanrussian.com
Posted by: Dichaelciree | April 27, 2024 3:54 PM
На сегодняшний день, когда диплом - это начало отличной карьеры в любой области, многие стараются найти максимально быстрый и простой путь получения образования. Факт наличия документа об образовании сложно переоценить. Ведь именно он открывает двери перед всеми, кто собирается начать трудовую деятельность или продолжить обучение в ВУЗе.
В данном контексте наша компания предлагает очень быстро получить любой необходимый документ. Вы сможете приобрести диплом нового или старого образца, и это будет удачным решением для человека, который не смог закончить обучение или потерял документ. Все дипломы производятся аккуратно, с особым вниманием ко всем деталям, чтобы в итоге получился продукт, полностью соответствующий оригиналу.
Преимущества такого решения заключаются не только в том, что можно быстро получить свой диплом. Процесс организован удобно и легко, с нашей поддержкой. От выбора необходимого образца до консультации по заполнению персональных данных и доставки по стране — все под абсолютным контролем наших мастеров.
Всем, кто пытается найти быстрый способ получить требуемый документ, наша компания предлагает отличное решение. Купить диплом - это значит избежать долгого процесса обучения и не теряя времени переходить к достижению своих целей, будь то поступление в университет или старт карьеры.
https://diploman-russiyans.com
Posted by: Lhanesaugs | April 27, 2024 9:26 PM
go to website Retirement planning
Posted by: JeremyFot | April 27, 2024 9:53 PM
В наше время, когда диплом - это начало успешной карьеры в любом направлении, многие стараются найти максимально быстрый путь получения качественного образования. Наличие документа об образовании сложно переоценить. Ведь диплом открывает двери перед любым человеком, желающим начать трудовую деятельность или учиться в высшем учебном заведении.
В данном контексте мы предлагаем максимально быстро получить этот важный документ. Вы можете купить диплом нового или старого образца, что становится отличным решением для человека, который не смог завершить образование или утратил документ. Все дипломы выпускаются аккуратно, с максимальным вниманием к мельчайшим элементам, чтобы в итоге получился продукт, 100% соответствующий оригиналу.
Преимущества данного подхода состоят не только в том, что вы сможете максимально быстро получить диплом. Весь процесс организован просто и легко, с профессиональной поддержкой. От выбора необходимого образца до правильного заполнения личной информации и доставки в любое место России — все находится под полным контролем наших мастеров.
Для всех, кто пытается найти оперативный способ получения необходимого документа, наша компания предлагает отличное решение. Приобрести диплом - значит избежать долгого процесса обучения и не теряя времени перейти к достижению своих целей, будь то поступление в университет или начало карьеры.
https://diploman-russiyans.com
Posted by: Shanesaugs | April 28, 2024 3:03 AM
читать https://battlepass.ru
Posted by: JohnnyDep | April 28, 2024 4:01 AM
leasingber.ru
Posted by: leasingber | April 28, 2024 4:24 AM
blacksprut ссылка - bs2web, bs2web
Posted by: Jamesarriz | April 28, 2024 4:59 AM
Профили для облицовки стен являются неотъемлемой частью современного ремонта и помогают преобразить любое помещение пластиковый уголок для плитки
Posted by: Gilberthaw | April 28, 2024 5:40 AM
Premium domain for sale admiral-x1g.ru
Posted by: BOSFab | April 28, 2024 5:58 AM
lopressor brand - telmisartan 20mg cheap nifedipine 30mg tablet
Posted by: Resfvo | April 28, 2024 7:56 AM
В нашем обществе, где диплом - это начало удачной карьеры в любом направлении, многие ищут максимально быстрый и простой путь получения качественного образования. Наличие официального документа об образовании переоценить попросту невозможно. Ведь диплом открывает двери перед любым человеком, желающим вступить в сообщество профессионалов или учиться в высшем учебном заведении.
Наша компания предлагает максимально быстро получить этот важный документ. Вы можете заказать диплом нового или старого образца, что будет удачным решением для всех, кто не смог закончить обучение или утратил документ. Любой диплом изготавливается аккуратно, с максимальным вниманием ко всем нюансам, чтобы в результате получился продукт, максимально соответствующий оригиналу.
Превосходство данного решения состоит не только в том, что вы оперативно получите диплом. Весь процесс организован удобно, с профессиональной поддержкой. Начиная от выбора нужного образца до консультации по заполнению личной информации и доставки в любое место России — все будет находиться под абсолютным контролем наших специалистов.
В результате, всем, кто пытается найти оперативный способ получения требуемого документа, наша компания готова предложить отличное решение. Приобрести диплом - это значит избежать длительного процесса обучения и не теряя времени переходить к достижению личных целей, будь то поступление в ВУЗ или начало карьеры.
https://diploman-russiyans.com
Posted by: Fobertfleld | April 28, 2024 8:37 AM
В современном мире, где диплом является началом отличной карьеры в любой области, многие стараются найти максимально простой путь получения качественного образования. Необходимость наличия документа об образовании сложно переоценить. Ведь диплом открывает дверь перед каждым человеком, который желает вступить в профессиональное сообщество или учиться в высшем учебном заведении.
Наша компания предлагает максимально быстро получить этот необходимый документ. Вы сможете купить диплом старого или нового образца, что будет выгодным решением для всех, кто не смог закончить образование, потерял документ или желает исправить свои оценки. Каждый диплом изготавливается аккуратно, с особым вниманием к мельчайшим элементам, чтобы в результате получился продукт, полностью соответствующий оригиналу.
Преимущества этого решения состоят не только в том, что можно оперативно получить свой диплом. Весь процесс организован комфортно и легко, с профессиональной поддержкой. Начиная от выбора нужного образца до консультации по заполнению личных данных и доставки в любое место России — все под полным контролем качественных мастеров.
Всем, кто пытается найти быстрый способ получения требуемого документа, наша услуга предлагает выгодное решение. Купить диплом - значит избежать долгого процесса обучения и сразу перейти к личным целям: к поступлению в университет или к началу успешной карьеры.
https://www.google.co.mz/url?q=https://rudiplomista.com
https://image.google.tk/url?sa=t&source=web&rct=j&url=https://rudiplomista.com
https://cse.google.sm/url?q=https://premialnie-diplomik.com
https://maps.google.fr/url?q=https://lands-diploma.com
https://image.google.mg/url?q=https://rudiplomista.com
Posted by: Shanesaugs | April 28, 2024 11:09 AM
Перед тем как приступить к поиску идеального транспортного средства, важно определить ваши потребности и предпочтения. Обдумайте, для каких целей вам необходимо автомобиль или другое транспортное средство, какие характеристики будут наиболее приоритетными для вас, и какой бюджет вы готовы выделить на покупку https://smf.sos-dan.ru/index.php?topic=452564.new#new
Posted by: RichardBlore | April 28, 2024 12:46 PM
В нашем обществе, где диплом - это начало успешной карьеры в любой области, многие ищут максимально быстрый путь получения качественного образования. Наличие официального документа об образовании сложно переоценить. Ведь именно диплом открывает двери перед людьми, желающими вступить в сообщество профессиональных специалистов или продолжить обучение в университете.
В данном контексте наша компания предлагает очень быстро получить этот необходимый документ. Вы имеете возможность купить диплом нового или старого образца, что является удачным решением для всех, кто не смог завершить образование или утратил документ. Любой диплом изготавливается аккуратно, с максимальным вниманием к мельчайшим нюансам, чтобы в результате получился документ, полностью соответствующий оригиналу.
Плюсы этого подхода состоят не только в том, что можно максимально быстро получить диплом. Процесс организовывается комфортно, с нашей поддержкой. Начиная от выбора требуемого образца до точного заполнения личной информации и доставки по стране — все будет находиться под полным контролем качественных мастеров.
Всем, кто ищет оперативный способ получения необходимого документа, наша услуга предлагает выгодное решение. Заказать диплом - значит избежать продолжительного процесса обучения и сразу перейти к своим целям, будь то поступление в ВУЗ или начало удачной карьеры.
https://diploman-rossiya.com
Posted by: Dichaelciree | April 28, 2024 2:14 PM
buy hydrochlorothiazide - brand norvasc buy bisoprolol online cheap
Posted by: Kbrzto | April 28, 2024 6:37 PM
В современном мире, где диплом - это начало отличной карьеры в любом направлении, многие стараются найти максимально простой путь получения образования. Наличие официального документа об образовании переоценить невозможно. Ведь именно диплом открывает дверь перед каждым человеком, желающим начать профессиональную деятельность или продолжить обучение в университете.
В данном контексте наша компания предлагает быстро получить этот важный документ. Вы можете заказать диплом нового или старого образца, и это является выгодным решением для человека, который не смог завершить образование или утратил документ. Все дипломы производятся аккуратно, с максимальным вниманием ко всем деталям, чтобы в итоге получился 100% оригинальный документ.
Преимущество данного решения состоит не только в том, что вы оперативно получите свой диплом. Процесс организован комфортно, с профессиональной поддержкой. Начав от выбора нужного образца диплома до консультации по заполнению личной информации и доставки по России — все под абсолютным контролем опытных специалистов.
В результате, всем, кто ищет оперативный способ получить требуемый документ, наша компания готова предложить отличное решение. Купить диплом - это значит избежать долгого процесса обучения и не теряя времени переходить к достижению личных целей, будь то поступление в университет или начало карьеры.
https://diploman-russiyans.com
Posted by: Timsothywok | April 28, 2024 7:31 PM
В нашем обществе, где диплом - это начало успешной карьеры в любой области, многие ищут максимально простой путь получения качественного образования. Наличие официального документа об образовании переоценить невозможно. Ведь диплом открывает двери перед всеми, кто стремится начать профессиональную деятельность или учиться в университете.
Наша компания предлагает быстро получить этот необходимый документ. Вы сможете приобрести диплом нового или старого образца, что будет удачным решением для всех, кто не смог закончить образование, утратил документ или желает исправить плохие оценки. диплом изготавливается с особой аккуратностью, вниманием ко всем нюансам. В итоге вы сможете получить продукт, максимально соответствующий оригиналу.
Плюсы такого решения заключаются не только в том, что вы быстро получите диплом. Весь процесс организовывается комфортно, с профессиональной поддержкой. От выбора подходящего образца документа до консультации по заполнению персональных данных и доставки в любое место страны — все будет находиться под абсолютным контролем наших специалистов.
Таким образом, для всех, кто пытается найти оперативный способ получения необходимого документа, наша услуга предлагает выгодное решение. Заказать диплом - значит избежать продолжительного процесса обучения и не теряя времени переходить к достижению личных целей: к поступлению в ВУЗ или к началу трудовой карьеры.
https://dlplomanrussian.com
Posted by: StephenVog | April 29, 2024 2:27 AM
На данный момент лучший способ бесплатно смотреть фильмы 2024 онлайн - это онлайн-кинотеатр Film-2024.com фильмы 2024 в хорошем качестве
Posted by: JamesOwnef | April 29, 2024 3:03 AM
fortnite aimbot download pc - soft aim fortnite download, fortnite aimbot download
Posted by: Williediz | April 29, 2024 3:56 AM
Магазин учитывает пожелания каждого покупателя, предоставляя персональные решения для освещения различных помещений http://www.clean-ace8.com/bbs/board.php?bo_table=free&wr_id=302431
Posted by: CarlosHox | April 29, 2024 5:49 AM
В современном мире, где диплом становится началом отличной карьеры в любом направлении, многие стараются найти максимально быстрый и простой путь получения образования. Важность наличия документа об образовании сложно переоценить. Ведь именно диплом открывает дверь перед любым человеком, желающим вступить в сообщество квалифицированных специалистов или учиться в ВУЗе.
Наша компания предлагает быстро получить этот важный документ. Вы можете купить диплом, что становится удачным решением для человека, который не смог закончить обучение или утратил документ. Все дипломы выпускаются аккуратно, с особым вниманием ко всем нюансам. На выходе вы сможете получить полностью оригинальный документ.
Преимущество подобного подхода заключается не только в том, что можно оперативно получить диплом. Процесс организовывается просто и легко, с нашей поддержкой. Начиная от выбора нужного образца документа до консультаций по заполнению персональных данных и доставки в любой регион России — все будет находиться под полным контролем квалифицированных специалистов.
Для всех, кто пытается найти оперативный способ получить необходимый документ, наша компания предлагает выгодное решение. Купить диплом - это значит избежать продолжительного обучения и сразу перейти к достижению личных целей: к поступлению в ВУЗ или к началу удачной карьеры.
https://diploman-rossiya.com
Posted by: LewisJetty | April 29, 2024 7:55 AM
В нашем обществе, где диплом - это начало успешной карьеры в любом направлении, многие пытаются найти максимально быстрый путь получения качественного образования. Факт наличия документа об образовании переоценить просто невозможно. Ведь диплом открывает дверь перед людьми, стремящимися начать трудовую деятельность или продолжить обучение в любом институте.
Наша компания предлагает очень быстро получить любой необходимый документ. Вы сможете заказать диплом старого или нового образца, и это становится удачным решением для человека, который не смог закончить образование, потерял документ или хочет исправить плохие оценки. дипломы изготавливаются с особой тщательностью, вниманием ко всем элементам. В итоге вы сможете получить документ, максимально соответствующий оригиналу.
Преимущество подобного решения состоит не только в том, что вы сможете оперативно получить свой диплом. Процесс организован просто и легко, с нашей поддержкой. От выбора подходящего образца диплома до правильного заполнения персональных данных и доставки по России — все находится под полным контролем квалифицированных специалистов.
В итоге, для всех, кто ищет быстрый способ получить требуемый документ, наша компания готова предложить выгодное решение. Приобрести диплом - значит избежать продолжительного процесса обучения и сразу перейти к своим целям, будь то поступление в ВУЗ или старт профессиональной карьеры.
https://diplomanc-russia24.com
Posted by: Fobertfleld | April 29, 2024 1:29 PM
Hi there, its good paragraph concerning media print, we all be familiar with media is a fantastic source of information.
Официальный vavada Online Casino - бонусы на первый депозит 100% от 1000$! Максбет турниры на реальный деньги и автомобили Mercedes в Vavada казино!
Posted by: Shanesaugs | April 29, 2024 2:15 PM
In Etsy, Shopify Pinterest+SEO + artificial intelligence give high sales results
Posted by: EvelynNindy | April 29, 2024 5:46 PM
В нашем мире, где диплом - это начало успешной карьеры в любом направлении, многие ищут максимально быстрый путь получения образования. Факт наличия документа об образовании переоценить невозможно. Ведь диплом открывает двери перед людьми, стремящимися начать трудовую деятельность или учиться в ВУЗе.
В данном контексте мы предлагаем максимально быстро получить этот необходимый документ. Вы имеете возможность приобрести диплом, и это является отличным решением для всех, кто не смог завершить образование или потерял документ. Все дипломы изготавливаются аккуратно, с особым вниманием ко всем нюансам, чтобы в результате получился 100% оригинальный документ.
Преимущества такого решения заключаются не только в том, что можно максимально быстро получить диплом. Весь процесс организовывается комфортно, с профессиональной поддержкой. Начиная от выбора требуемого образца до правильного заполнения персональной информации и доставки по России — все находится под абсолютным контролем опытных мастеров.
Всем, кто ищет максимально быстрый способ получения требуемого документа, наша компания предлагает отличное решение. Купить диплом - это значит избежать долгого процесса обучения и не теряя времени переходить к важным целям, будь то поступление в ВУЗ или начало успешной карьеры.
https://diplomanc-russia24.com
Posted by: Ismaelanode | April 29, 2024 6:46 PM
I was really itching to fix it some wager some change on some sports matches that are happening above-board now. I wanted to say you guys identify that I did twig what I weigh to be the kindest site in the USA.
If you want to get in on the spirit, verify it out: best crypto casino
Posted by: Vjiupsmor | April 29, 2024 10:54 PM
В наше время, когда диплом является началом удачной карьеры в любом направлении, многие пытаются найти максимально простой путь получения образования. Наличие официального документа переоценить невозможно. Ведь диплом открывает двери перед всеми, кто стремится начать трудовую деятельность или учиться в высшем учебном заведении.
В данном контексте наша компания предлагает очень быстро получить этот важный документ. Вы имеете возможность купить диплом нового или старого образца, и это будет отличным решением для человека, который не смог завершить образование или утратил документ. Все дипломы выпускаются с особой аккуратностью, вниманием ко всем нюансам, чтобы в результате получился полностью оригинальный документ.
Превосходство такого подхода состоит не только в том, что можно оперативно получить диплом. Процесс организовывается удобно и легко, с профессиональной поддержкой. От выбора требуемого образца до консультации по заполнению персональной информации и доставки по России — все под полным контролем квалифицированных мастеров.
Всем, кто ищет оперативный способ получить требуемый документ, наша компания предлагает выгодное решение. Приобрести диплом - значит избежать долгого обучения и не теряя времени переходить к своим целям: к поступлению в университет или к началу успешной карьеры.
https://dlplomanrussian.com
Posted by: Timsothywok | April 30, 2024 12:05 AM
GET X - финансовая игра с выводом реальных средств. На данный момент на сайте представлена одна самых популярных и известных финансовых игр - Crash гет икс официальный сайт
Posted by: DwightDeabe | April 30, 2024 2:43 AM
Сегодня, когда диплом - это начало отличной карьеры в любой отрасли, многие пытаются найти максимально быстрый путь получения образования. Наличие официального документа трудно переоценить. Ведь именно диплом открывает двери перед любым человеком, желающим начать профессиональную деятельность или продолжить обучение в ВУЗе.
Мы предлагаем очень быстро получить любой необходимый документ. Вы сможете купить диплом старого или нового образца, что является удачным решением для всех, кто не смог завершить образование, утратил документ или хочет исправить плохие оценки. дипломы выпускаются с особой тщательностью, вниманием ко всем деталям. На выходе вы сможете получить продукт, полностью соответствующий оригиналу.
Превосходство подобного решения состоит не только в том, что вы быстро получите свой диплом. Весь процесс организован комфортно, с профессиональной поддержкой. Начиная от выбора нужного образца диплома до консультации по заполнению персональной информации и доставки по России — все будет находиться под полным контролем опытных специалистов.
В итоге, для тех, кто пытается найти быстрый и простой способ получить требуемый документ, наша компания предлагает выгодное решение. Купить диплом - значит избежать длительного процесса обучения и не теряя времени переходить к достижению собственных целей: к поступлению в университет или к началу успешной карьеры.
https://diplomanc-russia24.com
Posted by: Timsothywok | April 30, 2024 5:53 AM
Дизайн-радиаторы — функциональное украшение интерьера, доступные в разных цветах и размерах, с возможностью добавления тэнов https://telegra.ph/CHto-takoe-dizajn-radiatory-otopleniya-03-11
Posted by: DanielRew | April 30, 2024 9:21 AM
В нашем обществе, где диплом - это начало успешной карьеры в любом направлении, многие ищут максимально быстрый и простой путь получения качественного образования. Важность наличия документа об образовании переоценить попросту невозможно. Ведь именно диплом открывает дверь перед всеми, кто хочет вступить в сообщество профессиональных специалистов или учиться в ВУЗе.
Наша компания предлагает быстро получить этот важный документ. Вы можете купить диплом, и это становится выгодным решением для человека, который не смог завершить образование или потерял документ. диплом изготавливается с особой аккуратностью, вниманием к мельчайшим элементам. В результате вы получите 100% оригинальный документ.
Плюсы подобного подхода заключаются не только в том, что вы сможете быстро получить свой диплом. Процесс организован удобно, с нашей поддержкой. От выбора подходящего образца документа до консультаций по заполнению персональной информации и доставки в любое место страны — все будет находиться под абсолютным контролем качественных специалистов.
Всем, кто пытается найти быстрый способ получить необходимый документ, наша компания может предложить выгодное решение. Приобрести диплом - значит избежать долгого процесса обучения и сразу переходить к достижению личных целей, будь то поступление в ВУЗ или старт карьеры.
https://diploman-russiyan.com
Posted by: OLanesaugs | April 30, 2024 11:06 AM
Холодильники Smeg сочетают стиль и технологии, поддерживая продукты свежими. Они включают модели с различными функциями и стильным дизайном, от ретро до современных. Серия 50's Style выделяется яркими цветами и ретро-дизайном. Энергоэффективность классов A+ и A++ обеспечивает экономию электроэнергии, делая Smeg идеальным для любого интерьера Холодильник Smeg
Posted by: Martinunfok | April 30, 2024 12:33 PM
"Фанат Байкала" предоставляет доступные путевки на 2024 год, позволяя каждому насладиться красотой Байкала. Мы предлагаем разнообразные туры, включая эконом-варианты и VIP-путешествия. Цены варьируются в зависимости от длительности тура и предоставляемых услуг, что позволяет вам выбрать идеальный вариант соответственно вашему бюджету. Забронировав путевку заранее, вы гарантируете себе лучшие условия проживания и экскурсионное сопровождение по самым выгодным ценам.
Fanatbaikala - байкальский отдых турагентство имеет офисы в Иркутске и Москве. В Иркутске наш офис расположен по адресу ул. Дальневосточная, 146, офис 4, телефон для связи: +7 (3952) 480-539, для заказа туров используйте e-mail: info@fanatbaikala.ru, для вопросов сотрудничества: Partners@fanatbaikala.ru. Московский офис находится на ул. Земляной Вал, д.9, офис 417, с контактным телефоном +7(499) 40-40-538. Оба офиса предоставляют полный спектр услуг по организации туров на Байкал.
Posted by: Bailakewet | April 30, 2024 4:35 PM
Алматы Азарт - это уникальное игровое пространство для людей старше 30 лет, которые ценят интеллектуальный досуг и общение https://heylink.me/play_tg/
Posted by: JamesSog | April 30, 2024 4:59 PM
purchase nitroglycerin pills - order nitroglycerin online cheap diovan 80mg generic
Posted by: Mhbsto | April 30, 2024 5:47 PM
Hello.
This post was created with 2ssdsd3222aa.com
Posted by: TumerEvima | April 30, 2024 8:49 PM
"Фанат Байкала" предоставляет доступные путевки на 2024 год, позволяя каждому насладиться красотой Байкала. Мы предлагаем разнообразные туры, включая эконом-варианты и VIP-путешествия. Цены варьируются в зависимости от длительности тура и предоставляемых услуг, что позволяет вам выбрать идеальный вариант соответственно вашему бюджету. Забронировав путевку заранее, вы гарантируете себе лучшие условия проживания и экскурсионное сопровождение по самым выгодным ценам.
Fanatbaikala - туры на байкал из улан удэ имеет офисы в Иркутске и Москве. В Иркутске наш офис расположен по адресу ул. Дальневосточная, 146, офис 4, телефон для связи: +7 (3952) 480-539, для заказа туров используйте e-mail: info@fanatbaikala.ru, для вопросов сотрудничества: Partners@fanatbaikala.ru. Московский офис находится на ул. Земляной Вал, д.9, офис 417, с контактным телефоном +7(499) 40-40-538. Оба офиса предоставляют полный спектр услуг по организации туров на Байкал.
Posted by: Perydewet | April 30, 2024 11:35 PM
Поставить счетчики на воду в квартире – означает значительно снизить затраты на оплату этой части ЖКХ https://progkh.ru/
Posted by: StevenSof | May 1, 2024 2:26 AM
Good Day
I have just took a look on your SEO for jefflewis.net for its SEO metrics and saw that your website could use a boost.
We will increase your ranks organically and safely, using only state of the art AI and whitehat methods, while providing monthly reports and outstanding support.
More info:
https://www.digital-x-press.com/unbeatable-seo/
Regards
Mike Walkman
Digital X SEO Experts
Posted by: Mike Walkman | May 1, 2024 2:45 AM
Стоимость поездки на Байкал зависит от многих факторов, включая продолжительность тура, тип размещения и предоставляемые услуги. Простые однодневные экскурсии можно найти по более низкой цене, в то время как многодневные туры с проживанием в комфортных условиях будут стоить дороже. Наши специалисты готовы предложить вам различные варианты, чтобы вы могли выбрать идеальное сочетание приключения и релаксации по наилучшей цене.
Fanatbaikala - на ольхон из улан удэ тур имеет офисы в Иркутске и Москве. В Иркутске наш офис расположен по адресу ул. Дальневосточная, 146, офис 4, телефон для связи: +7 (3952) 480-539, для заказа туров используйте e-mail: info@fanatbaikala.ru, для вопросов сотрудничества: Partners@fanatbaikala.ru. Московский офис находится на ул. Земляной Вал, д.9, офис 417, с контактным телефоном +7(499) 40-40-538. Оба офиса предоставляют полный спектр услуг по организации туров на Байкал.
Posted by: Flaprowet | May 1, 2024 2:58 AM
We bieden tijdelijke telefoonnummers in tientallen landen over de hele wereld, met duizenden nummers om uit te kiezen telegram zonderВ telefoonnummer
Posted by: WillieMew | May 1, 2024 6:37 AM
Terrific post however , I was wanting to know if you could write a litte more on this topic? I'd be very thankful if you could elaborate a little bit more. Bless you!
nudify app
Posted by: StephenVog | May 1, 2024 1:42 PM
Wanneer krijg ik een tijdelijk nummer? Als je met nummerbehoud overstapt naar Odido, krijg je van ons een tijdelijk Odido telefoonnummer bij je nieuwe simkaart https://rafaelqzip41863.ezblogz.com/58307773/de-vrijheid-van-telegram-zonder-telefoonnummer
Posted by: RichardKed | May 1, 2024 4:04 PM
Descopera o experienta unica de cumparaturi pentru adidasii Puma pe site-ul nostru PumaMoldova.md! Cu o gama variata de modele si tehnologii avansate, fiecare pereche de adidasi este proiectata pentru a oferi confort si performanta. Cum sa cumperi adidasii potriviti pentru tine? incepe prin a explora selectia noastra si gaseste perechea care se potriveste stilului si nevoilor tale. Adauga-ti produsele favorite in cosul de cumparaturi si finalizeaza comanda cu cativa pasi simpli. Beneficiaza de livrare rapida si sigura, astfel incat sa te bucuri rapid de noua ta achizitie. Cu serviciul nostru de relatii cu clientii, poti obtine orice informatii sau asistenta de care ai nevoie. Alege Puma pentru o combinatie perfecta de stil si performanta in fiecare pas!
Posted by: Pumamoldova | May 1, 2024 5:59 PM
In Etsy, Shopify Pinterest+SEO + artificial intelligence give high sales results
Posted by: CarlosTit | May 1, 2024 10:14 PM
zocor sniff - tricor statue lipitor concentrate
Posted by: Lffeom | May 2, 2024 1:17 AM
Wanneer krijg ik een tijdelijk nummer? Als je met nummerbehoud overstapt naar Odido, krijg je van ons een tijdelijk Odido telefoonnummer bij je nieuwe simkaart https://erickgzsg32100.widblog.com/81637228/het-belang-van-een-telegram-telefoonnummer
Posted by: RobertStase | May 2, 2024 2:54 AM
Изготавливаем столешницы, подоконники, барные стойки и другую мебель из акрилового камня и кварцевого агломерата под заказ по выгодной цене в Москве кварцевый агломерат
Posted by: RobertStase | May 2, 2024 6:06 AM
Шикарные идеи для Вашего дизайна по ссылке. Трендовые новинки дизайна интерьера. Оцени самые новые идеи дизайна интерьера из Италии https://vitabreath.ru/news-564871-italyanskaya-mebel-sliyanie-stilya-krasoty-i-funktsionalnosti-v-kagdom-izdelii.html
Posted by: StevenVaw | May 2, 2024 9:42 AM
Thanks, I've been looking for this for a long time
_________________
казино на рубли с быстрым выводом
Posted by: Robertbon | May 2, 2024 10:22 AM
Наша компания предлагает высококачественные услуги Аренда Экскаватора 3 в 1 Алматы Мы обеспечиваем надежное и профессиональное оборудование для выполнения различных земляных работ на строительных площадках и других объектах. Наш опытный персонал и гибкие условия аренды делают нас надежным партнером для вашего проекта.
Posted by: LewisJetty | May 2, 2024 1:12 PM
Наш дайвинг клуб осуществляет обучение дайвингу от новичка до профессионала. Мы организуем пробные погружения с аквалангом в бассейнах Москвы и открытых водоемах Подмосковья. Кроме этого, в нашем дайв-клубе Вы можете приобрести подарочные сертификат на любой курс дайвинга или пробное погружение погружение с аквалангом в москве
Posted by: Arthurret | May 2, 2024 1:36 PM
В современном мире, где диплом является началом удачной карьеры в любом направлении, многие стараются найти максимально быстрый путь получения качественного образования. Наличие официального документа переоценить невозможно. Ведь диплом открывает двери перед людьми, желающими вступить в сообщество профессионалов или учиться в ВУЗе.
В данном контексте мы предлагаем быстро получить этот важный документ. Вы имеете возможность заказать диплом нового или старого образца, что становится удачным решением для человека, который не смог завершить образование или утратил документ. Каждый диплом изготавливается с особой аккуратностью, вниманием к мельчайшим элементам. На выходе вы сможете получить 100% оригинальный документ.
Плюсы подобного подхода состоят не только в том, что можно оперативно получить свой диплом. Весь процесс организован удобно, с нашей поддержкой. От выбора нужного образца диплома до консультации по заполнению персональной информации и доставки по России — все будет находиться под абсолютным контролем опытных мастеров.
Всем, кто ищет быстрый способ получения требуемого документа, наша услуга предлагает выгодное решение. Заказать диплом - значит избежать длительного процесса обучения и сразу перейти к своим целям, будь то поступление в ВУЗ или начало трудовой карьеры.
http://maps.google.pt/url?rct=j&sa=t&url=http://diploman-spb24.com
https://www.google.tk/url?sa=t&url=https://premialnie-diplomany.com
http://maps.google.pt/url?rct=j&sa=t&url=https://diploms-originalniy.com
http://images.google.com.na/url?q=http://kazdiplom.com
https://images.google.co.ke/url?sa=t&url=https://russiany-diplomans.com
Posted by: JseraldAparm | May 2, 2024 4:29 PM
I've been using the REDDI-PANDA for my landscaping projects, and I must say, it has truly elevated my work to new heights! The compact design and adjustable sizes make it so easy to maneuver on various terrains, from soil to gravel. It's been a game-changer in creating stable foundations and preparing the ground for new growth. Highly recommend checking out this versatile tool for your construction and landscaping needs! https://mandako.com/tillage/
Posted by: Timsothywok | May 2, 2024 5:46 PM
Мы организуем пробные погружения с аквалангом в бассейнах Москвы и открытых водоемах Подмосковья. Наш дайвинг клуб осуществляет обучение дайвингу от новичка до профессионала. Кроме этого, в нашем дайв-клубе Вы можете приобрести подарочные сертификат на любой курс дайвинга или пробное погружение школы дайвинга
Posted by: Arthurret | May 3, 2024 1:29 AM
Большой выбор игрушек для взрослых с быстрой курьерской доставкой. Анонимная упаковка – запечатанный крафт пакет Анальные игрушки Владивосток
Posted by: AlbertJoype | May 3, 2024 4:19 AM
кракен ссылка
Posted by: Marissaepike | May 3, 2024 5:00 AM
круиз в японию
Posted by: JaredAnarl | May 3, 2024 5:50 AM
катер таруса
Posted by: Thomasalork | May 3, 2024 9:21 AM
rosuvastatin pills express - rosuvastatin online potter caduet pills ball
Posted by: Iaybpc | May 3, 2024 9:36 AM
The topic of Royal Coins two: Hold and Win is undoubtedly an exquisite combination of regality and opulence, sweeping gamers right into a world where Every single spin is steeped during the promise of untold riches. If you’re in Zambia, African Betting Tutorial is here to help make your journey https://icelisting.com/story17855875/the-best-side-of-black-jack-in-zambia
Posted by: WilliamPlaub | May 3, 2024 11:54 AM
Стоимость обучения на курсе английского для начинающих онлайн в 1,3-2,5 раза ниже стоимости группового обучения на языковых курсах, где ориентируются на группу, а не ваши индивидуальные потребности курсы английский онлайн
Posted by: DouglasWag | May 3, 2024 4:42 PM
natural remedy gout https://www.jotform.com/241063566693058 drug treatment seattle
Posted by: Terryviale | May 3, 2024 8:27 PM
Пластическая хирургия — увеличение груди имплантами, подтяжка груди, уменьшение груди. Абдоминопластика — пластика по уменьшению живота, липосакция и липофилинг увеличение груди с имплантами
Posted by: Jamesfleve | May 4, 2024 1:40 AM
Get all the latest news and updates from FineProxy.Org delivered straight to your Telegram inbox. Join our channel now!
Posted by: Lhanesaugs | May 4, 2024 2:57 AM
Hey I know this is off topic but I was wondering if you knew of any widgets I could add to my blog that automatically tweet my newest twitter updates. I've been looking for a plug-in like this for quite some time and was hoping maybe you would have some experience with something like this. Please let me know if you run into anything. I truly enjoy reading your blog and I look forward to your new updates.
bc.game login
Posted by: Lhanesaugs | May 4, 2024 4:58 AM
Мощные и эффективные: генераторы пены ГПСС для вашего бизнеса. Повысьте уровень безопасности и гигиены с нашими генераторами пены ГПСС комплектующие для нефтебаз
Posted by: RobertNaift | May 4, 2024 5:08 AM
prednisone 20mg price prednisone and psychosis prednisone cream prednisone for bells palsy prednisone 20 mg 5 days
Posted by: htaletofhb | May 4, 2024 9:19 AM
In Etsy, Amazon, eBay, Shopify https://pint77.com Pinterest+SEO +II = high sales results
Posted by: Robertstefs | May 4, 2024 12:41 PM
В этой подборке онлайн-курсов по английскому языку мы сравнили предложения школ по нескольким параметрам и отобрали лучшие варианты для взрослых и детей, начинающих познание языка или повышающих свой уровень курсы английского языка с носителем онлайн
Posted by: Jamesliasy | May 4, 2024 2:24 PM
В нашем мире, где диплом - это начало отличной карьеры в любом направлении, многие пытаются найти максимально простой путь получения образования. Необходимость наличия официального документа об образовании сложно переоценить. Ведь диплом открывает дверь перед любым человеком, который стремится вступить в профессиональное сообщество или продолжить обучение в университете.
Наша компания предлагает очень быстро получить этот важный документ. Вы можете заказать диплом, что становится отличным решением для человека, который не смог закончить образование, потерял документ или хочет исправить плохие оценки. Каждый диплом изготавливается с особой тщательностью, вниманием ко всем деталям, чтобы на выходе получился 100% оригинальный документ.
Превосходство данного решения заключается не только в том, что вы оперативно получите свой диплом. Весь процесс организован удобно, с профессиональной поддержкой. Начиная от выбора нужного образца диплома до правильного заполнения персональных данных и доставки по стране — все под полным контролем опытных мастеров.
В итоге, всем, кто пытается найти максимально быстрый способ получить требуемый документ, наша компания предлагает выгодное решение. Заказать диплом - значит избежать длительного обучения и не теряя времени переходить к достижению личных целей, будь то поступление в ВУЗ или начало карьеры.
https://image.google.tg/url?q=https://rudiplomisty.com
https://www.google.co.ug/url?q=http://kazdiplom.com
https://cse.google.li/url?q=http://diploman-spb24.com
https://images.google.com.mm/url?q=https://russiany-diplomans.com
https://clients1.google.com.pa/url?sa=t&url=http://volga-diplomy.com
Posted by: Timsothywok | May 5, 2024 2:08 AM
cp loli
==> eit.tw/04G9JV
==> url.epoch.tw/Z4RGq
Posted by: Bobbydreax | May 5, 2024 3:31 AM
В этой подборке онлайн-курсов по английскому языку мы сравнили предложения школ по нескольким параметрам и отобрали лучшие варианты для взрослых и детей, начинающих познание языка или повышающих свой уровень онлайн курсы разговорного английского
Posted by: Jamesliasy | May 5, 2024 4:10 AM
Howdy exceptional blog! Does running a blog like this require a lot of work? I have virtually no understanding of computer programming but I was hoping to start my own blog soon. Anyway, should you have any recommendations or techniques for new blog owners please share. I understand this is off subject but I simply needed to ask. Appreciate it!
https://blogfreely.net/arwynerceb/h1-b-vazhlivist-iakostevogo-skla-far-farfarlight-dlia-bezpeki-na
Posted by: Shanesaugs | May 5, 2024 5:09 AM
Hi there mates, its impressive piece of writing regarding teachingand fully explained, keep it up all the time.
https://open.substack.com/pub/manuelhlze265/p/farfarlight?r=3tijtn&utm_campaign=post&utm_medium=web&showWelcomeOnShare=true
Posted by: StephenVog | May 5, 2024 11:06 AM
разработка сайта Л2 - разработка сайта csgo, дизайн сайта Lineage 2
Posted by: Bennyzeshy | May 5, 2024 12:06 PM
Hello, always i used to check blog posts here in the early hours in the break of day, since i enjoy to gain knowledge of more and more.
https://zenwriting.net/mosqueage8/kupuite-sklo-dlia-far-velikii-asortiment-i-dostupni-tsini
Posted by: OLanesaugs | May 5, 2024 2:43 PM
На сегодняшний день, когда диплом является началом удачной карьеры в любом направлении, многие пытаются найти максимально быстрый и простой путь получения качественного образования. Наличие официального документа переоценить попросту невозможно. Ведь именно он открывает двери перед каждым человеком, желающим начать профессиональную деятельность или учиться в высшем учебном заведении.
Мы предлагаем оперативно получить этот важный документ. Вы сможете приобрести диплом, что будет отличным решением для человека, который не смог завершить обучение, утратил документ или желает исправить плохие оценки. Все дипломы производятся аккуратно, с максимальным вниманием к мельчайшим деталям. На выходе вы сможете получить продукт, полностью соответствующий оригиналу.
Плюсы этого решения состоят не только в том, что вы сможете оперативно получить свой диплом. Весь процесс организован комфортно, с профессиональной поддержкой. От выбора необходимого образца диплома до правильного заполнения персональной информации и доставки по стране — все будет находиться под полным контролем качественных мастеров.
Таким образом, для всех, кто хочет найти максимально быстрый способ получения требуемого документа, наша компания предлагает отличное решение. Купить диплом - значит избежать длительного процесса обучения и не теряя времени переходить к достижению своих целей, будь то поступление в ВУЗ или старт трудовой карьеры.
http://images.google.co.nz/url?sa=t&url=https://rudiplomisty.com
https://maps.google.sk/url?q=https://russiany-diplomans.com
https://images.google.ad/url?q=https://lands-diploms.com
http://cse.google.co.za/url?sa=t&url=https://russdiplomik.com
https://image.google.gp/url?sa=i&rct=j&url=https://gosznac-diplomy.com
Posted by: DavdidPem | May 5, 2024 3:21 PM
сайт кракен тор - kraken darknet market зеркало, площадка кракен
Posted by: BruceRog | May 6, 2024 4:09 AM
Denver Cleaning Choice is a woman owned local business with more than 20 years experience in commercial cleaning services professional commercial cleaning in denver
Posted by: AndrewEraky | May 6, 2024 1:41 PM
В нашем мире, где диплом - это начало удачной карьеры в любой отрасли, многие ищут максимально простой путь получения качественного образования. Наличие официального документа переоценить попросту невозможно. Ведь диплом открывает двери перед всеми, кто собирается вступить в профессиональное сообщество или учиться в высшем учебном заведении.
Наша компания предлагает максимально быстро получить любой необходимый документ. Вы можете приобрести диплом, и это будет удачным решением для всех, кто не смог завершить обучение, утратил документ или хочет исправить свои оценки. дипломы изготавливаются аккуратно, с особым вниманием к мельчайшим элементам. В итоге вы получите документ, максимально соответствующий оригиналу.
Превосходство этого решения заключается не только в том, что вы сможете максимально быстро получить свой диплом. Процесс организовывается удобно, с профессиональной поддержкой. Начиная от выбора подходящего образца документа до консультации по заполнению личных данных и доставки по стране — все находится под абсолютным контролем наших мастеров.
Для всех, кто ищет быстрый и простой способ получения необходимого документа, наша услуга предлагает выгодное решение. Купить диплом - значит избежать долгого процесса обучения и не теряя времени переходить к достижению собственных целей, будь то поступление в университет или начало трудовой карьеры.
http://www.diploman-russia.com/
Posted by: Ismaelanode | May 6, 2024 2:31 PM
Howdy! This is kind of off topic but I need some guidance from an established blog. Is it very hard to set up your own blog? I'm not very techincal but I can figure things out pretty quick. I'm thinking about creating my own but I'm not sure where to start. Do you have any points or suggestions? With thanks
http://diploman-russiyans.com/
Posted by: Ismaelanode | May 6, 2024 3:26 PM
Накрутка голосов в конкурсах, опросах, петициях и рейтингах.
Накрутка голосов в конкурсах, опросах, петициях и рейтингах.!
Posted by: DavidRit | May 6, 2024 3:34 PM
What's up, I wish for to subscribe for this website to get latest updates, so where can i do it please assist.
undress girls ai
Posted by: Fobertfleld | May 6, 2024 7:33 PM
В нашем мире, где диплом является началом отличной карьеры в любом направлении, многие ищут максимально быстрый путь получения качественного образования. Важность наличия официального документа переоценить просто невозможно. Ведь именно диплом открывает двери перед людьми, желающими вступить в сообщество профессионалов или учиться в любом ВУЗе.
В данном контексте мы предлагаем максимально быстро получить этот необходимый документ. Вы можете купить диплом старого или нового образца, что становится выгодным решением для всех, кто не смог закончить обучение, потерял документ или хочет исправить плохие оценки. дипломы производятся с особой тщательностью, вниманием к мельчайшим деталям. В результате вы получите 100% оригинальный документ.
Преимущество этого решения заключается не только в том, что можно быстро получить диплом. Весь процесс организован комфортно, с профессиональной поддержкой. Начав от выбора подходящего образца до консультаций по заполнению персональных данных и доставки по России — все находится под полным контролем квалифицированных специалистов.
Для всех, кто хочет найти быстрый способ получить требуемый документ, наша компания предлагает отличное решение. Приобрести диплом - это значит избежать длительного обучения и сразу переходить к достижению личных целей: к поступлению в университет или к началу удачной карьеры.
https://diploman-russiyan.com/
Posted by: Dichaelciree | May 7, 2024 2:06 AM
Завершение учебы образования считается важным моментом в пути каждого человека, определяет его перспективы и профессиональные возможности - https://zakaz-na-diplom.ru. Диплом даёт доступ путь к свежим горизонтам и возможностям, гарантируя возможность к качественному образованию и высокопрестижным профессиям. В нынешнем обществе, где борьба на рынке труда постоянно увеличивается, наличие аттестата делает необходимым условием для выдающейся профессиональной деятельности. Он утверждает ваши знания, умения и навыки, умения и умения перед работодателями и социумом в целом. Кроме того, диплом дарит уверенность в себе и укрепляет оценку себя, что помогает личностному и саморазвитию. Получение диплома также является вложением в свое будущее, предоставляя устойчивость и достойный уровень жизни. Именно поэтому уделять должное внимание и время образованию и бороться за его получению, чтобы добиться успеха и удовлетворение от собственной труда.
Аттестат не только символизирует ваше образовательный уровень, но и отражает вашу самодисциплину, трудолюбие и упорство в достижении целей. Он представляет собой плодом труда и вложенных усилий, вкладываемых в учебу и саморазвитие. Завершение учебы образования открывает перед вами свежие перспективы возможностей, позволяя выбирать из множества карьерных путей и профессиональных направлений. Кроме того предоставляет вам базис знаний и навыков и умений, необходимых для выдающейся практики в современном мире, полном вызовами и изменениями. Более того, диплом является свидетельством вашей квалификации и экспертности, что в свою очередь повышает вашу привлекательность для работодателей на рынке труда и открывает перед вами двери к лучшим шансам для профессионального роста. Следовательно, завершение учебы диплома не лишь пополняет ваше личное самосовершенствование, а также раскрывает вами новые перспективы для достижения и амбиций.
Posted by: zakaz-na-diplom.ru | May 7, 2024 2:41 AM
cp loli
==> eit.tw/04G9JV
==> url.epoch.tw/Z4RGq
Posted by: Bobbydreax | May 7, 2024 5:21 AM
Входные двери - купить по выгодной цене с доставкой. 1433 модели в проверенных интернет-магазинах: популярные новинки и лидеры продаж https://market-dverey.com/
Posted by: Henrydix | May 7, 2024 5:27 AM
Недавно я решил обновить лестницу в своем доме, выбрав кованые перила. Сайт a-kovka.ru предложил мне не только широкий выбор дизайнов, но и прозрачные цены. Меня порадовало, что стоимость полностью соответствовала качеству – перила оказались исключительно прочными и эстетически привлекательными. Цена оказалась вполне доступной, учитывая высокий уровень мастерства и использование качественных материалов. Это было важно для меня, так как я искал лучшее соотношение цены и качества. Рекомендую a-kovka.ru всем, кто ценит красоту и долговечность.
Добро пожаловать в кузницу "А-ковка" кованые перила для лестниц цена - ваш надежный партнер в создании красивых и функциональных перил для вашего дома. Мы находимся в Москве, всего в 91 километре от МКАД, и готовы предложить вам широкий выбор кованых изделий, выполненных опытными мастерами.
Наша цена доступна для любого покупателя, а качество наших изделий всегда остается на высоте. Мы используем современное оборудование и материалы высокого качества, чтобы каждый заказчик остался доволен результатом. Кованые перила не только придают вашему интерьеру изысканный вид, но и являются прочным и долговечным решением для вашего дома. Закажите перила в нашей кузнице и убедитесь сами в высоком качестве наших изделий!
Posted by: кованые перила цена за метр | May 7, 2024 6:13 AM
В нашем обществе, где диплом - это начало удачной карьеры в любой сфере, многие ищут максимально быстрый путь получения качественного образования. Необходимость наличия официального документа переоценить попросту невозможно. Ведь диплом открывает двери перед каждым человеком, который собирается вступить в сообщество профессионалов или учиться в университете.
В данном контексте мы предлагаем очень быстро получить этот важный документ. Вы имеете возможность заказать диплом, что становится удачным решением для человека, который не смог завершить образование или потерял документ. диплом изготавливается аккуратно, с особым вниманием к мельчайшим элементам. В итоге вы сможете получить документ, полностью соответствующий оригиналу.
Превосходство такого решения состоит не только в том, что вы максимально быстро получите диплом. Процесс организовывается удобно, с нашей поддержкой. Начиная от выбора подходящего образца до точного заполнения личных данных и доставки в любое место России — все под абсолютным контролем наших специалистов.
Для тех, кто ищет максимально быстрый способ получить необходимый документ, наша компания предлагает выгодное решение. Купить диплом - это значит избежать долгого обучения и сразу перейти к достижению собственных целей: к поступлению в университет или к началу трудовой карьеры.
https://diploman-russiyan.com/
Posted by: Lhanesaugs | May 7, 2024 2:03 PM
БК Зенит для андроид zenitbet1.com
Если Вы искали букмекерская контора zenit в сети интернет, то заходите на наш веб ресурс прямо сейчас. Зеркало, как правило, регулярно блокируют и необходимо его искать еще раз. С помощью сайта zenitbet1.com больше не будет сложности в поиске. Мы регулярно проверяем и обновляем ссылку на вход зеркало ЗенитБет. Возможно подписаться на рассылку и Вам на Email будет приходить новая информация о входе на сайт.
Posted by: Zenitbet1 | May 7, 2024 3:12 PM
In Etsy, Amazon, eBay, Shopify https://pint77.com Pinterest+SEO +II = high sales results
Posted by: Robertstefs | May 7, 2024 4:15 PM
Protect your rights with us!
Get professional legal advice from experienced lawyers.
Visit our lawyers website and schedule a meeting today.
Your safety and peace are our top priority!
Our website https://adm-tigin.ru
Posted by: Stevengrout | May 7, 2024 6:05 PM
семаглутид аптека - мунджаро, семаглутид цена +в москве
Posted by: Jeraldaceks | May 7, 2024 8:53 PM
https://market-dverey.com/
Posted by: Henrydix | May 8, 2024 12:39 AM
В нашем обществе, где диплом становится началом отличной карьеры в любой сфере, многие ищут максимально быстрый и простой путь получения качественного образования. Наличие документа об образовании переоценить невозможно. Ведь диплом открывает двери перед любым человеком, желающим начать профессиональную деятельность или учиться в университете.
Наша компания предлагает максимально быстро получить этот важный документ. Вы имеете возможность купить диплом, что становится выгодным решением для всех, кто не смог закончить обучение, потерял документ или желает исправить свои оценки. Все дипломы выпускаются с особой аккуратностью, вниманием к мельчайшим элементам. На выходе вы получите продукт, максимально соответствующий оригиналу.
Превосходство этого решения заключается не только в том, что можно быстро получить свой диплом. Процесс организовывается комфортно, с нашей поддержкой. Начав от выбора нужного образца диплома до консультаций по заполнению персональной информации и доставки по России — все под полным контролем опытных мастеров.
Всем, кто хочет найти быстрый и простой способ получения необходимого документа, наша компания предлагает выгодное решение. Приобрести диплом - это значит избежать долгого обучения и сразу перейти к достижению собственных целей: к поступлению в университет или к началу успешной карьеры.
http://www.diplomanc-russia24.com
Posted by: Timsothywok | May 8, 2024 1:43 AM
sweat drug test https://www.jotform.com/241063566693058 drug counseling degree
Posted by: MathewPoomy | May 8, 2024 6:47 AM
mizugigurabia.com
Posted by: Francisadurf | May 8, 2024 10:17 AM
В современном мире, где диплом - это начало удачной карьеры в любом направлении, многие пытаются найти максимально быстрый и простой путь получения качественного образования. Необходимость наличия официального документа об образовании сложно переоценить. Ведь именно диплом открывает двери перед любым человеком, желающим вступить в сообщество квалифицированных специалистов или продолжить обучение в ВУЗе.
Наша компания предлагает быстро получить любой необходимый документ. Вы можете заказать диплом нового или старого образца, и это будет удачным решением для человека, который не смог закончить образование, утратил документ или желает исправить плохие оценки. диплом изготавливается аккуратно, с особым вниманием ко всем элементам. В итоге вы получите продукт, максимально соответствующий оригиналу.
Преимущества такого решения заключаются не только в том, что вы оперативно получите свой диплом. Весь процесс организовывается комфортно, с профессиональной поддержкой. Начиная от выбора подходящего образца документа до точного заполнения персональной информации и доставки по России — все будет находиться под полным контролем наших мастеров.
Для тех, кто пытается найти оперативный способ получения необходимого документа, наша услуга предлагает отличное решение. Приобрести диплом - это значит избежать длительного обучения и сразу перейти к личным целям: к поступлению в университет или к началу успешной карьеры.
http://diplomvam.ru
Posted by: OLanesaugs | May 8, 2024 3:53 PM
Hi there, after reading this awesome post i am as well cheerful to share my knowledge here with mates.
https://jutsu.icu/
Posted by: Fobertfleld | May 8, 2024 4:30 PM
В нашем обществе, где диплом - это начало отличной карьеры в любом направлении, многие стараются найти максимально быстрый и простой путь получения образования. Факт наличия документа об образовании переоценить невозможно. Ведь диплом открывает дверь перед каждым человеком, который желает вступить в сообщество профессиональных специалистов или продолжить обучение в высшем учебном заведении.
Мы предлагаем очень быстро получить любой необходимый документ. Вы можете приобрести диплом старого или нового образца, и это является отличным решением для человека, который не смог закончить обучение или потерял документ. диплом изготавливается с особой тщательностью, вниманием к мельчайшим нюансам. В итоге вы получите 100% оригинальный документ.
Преимущества такого решения состоят не только в том, что можно быстро получить диплом. Процесс организован комфортно, с нашей поддержкой. Начав от выбора требуемого образца диплома до консультаций по заполнению личных данных и доставки по России — все находится под полным контролем наших мастеров.
Для тех, кто ищет оперативный способ получения требуемого документа, наша компания предлагает выгодное решение. Приобрести диплом - значит избежать длительного обучения и сразу перейти к достижению своих целей: к поступлению в университет или к началу успешной карьеры.
http://diplom-net.ru
Posted by: Gichardgrash | May 8, 2024 6:06 PM
Наши преподаватели и имеют богатый опыт обучения таким направлениям танца, как аргентинское танго, бачата, контемпорари, k-pop, Hight Heels, шафл. Обучаем танцу как детей, так и взрослых бачата для пар москва
Posted by: Davidren | May 9, 2024 12:26 AM
эскорт Москвы - досуг для взрослых объявления, эскорт услуги
Posted by: RickyDor | May 9, 2024 1:23 AM
В нашем мире, где диплом - это начало удачной карьеры в любой сфере, многие ищут максимально простой путь получения качественного образования. Необходимость наличия документа об образовании переоценить невозможно. Ведь диплом открывает двери перед людьми, стремящимися начать профессиональную деятельность или продолжить обучение в любом институте.
Мы предлагаем быстро получить этот необходимый документ. Вы сможете приобрести диплом, и это будет отличным решением для человека, который не смог завершить образование или потерял документ. Все дипломы выпускаются с особой тщательностью, вниманием к мельчайшим нюансам, чтобы в итоге получился документ, полностью соответствующий оригиналу.
Преимущество данного решения состоит не только в том, что можно оперативно получить свой диплом. Весь процесс организован удобно, с профессиональной поддержкой. От выбора подходящего образца до правильного заполнения персональных данных и доставки в любой регион России — все будет находиться под абсолютным контролем опытных специалистов.
Всем, кто хочет найти быстрый и простой способ получить требуемый документ, наша услуга предлагает отличное решение. Заказать диплом - это значит избежать долгого обучения и не теряя времени перейти к достижению своих целей, будь то поступление в ВУЗ или начало удачной карьеры.
http://vsediplomu.ru/
Posted by: StephenVog | May 9, 2024 2:47 AM
For the reason that the admin of this site is working, no uncertainty very quickly it will be renowned, due to its quality contents.
https://motflix.cc/
Posted by: Dichaelciree | May 9, 2024 3:46 AM
В нашем обществе, где диплом - это начало успешной карьеры в любом направлении, многие пытаются найти максимально быстрый путь получения качественного образования. Необходимость наличия документа об образовании переоценить просто невозможно. Ведь диплом открывает дверь перед каждым человеком, желающим начать трудовую деятельность или продолжить обучение в ВУЗе.
В данном контексте мы предлагаем очень быстро получить этот необходимый документ. Вы имеете возможность купить диплом, что является выгодным решением для человека, который не смог закончить обучение или утратил документ. Каждый диплом изготавливается с особой аккуратностью, вниманием ко всем элементам, чтобы на выходе получился документ, максимально соответствующий оригиналу.
Превосходство такого решения заключается не только в том, что вы сможете максимально быстро получить свой диплом. Весь процесс организовывается удобно и легко, с профессиональной поддержкой. Начав от выбора подходящего образца документа до консультаций по заполнению личных данных и доставки в любое место России — все будет находиться под абсолютным контролем качественных специалистов.
Для всех, кто ищет оперативный способ получения требуемого документа, наша услуга предлагает выгодное решение. Приобрести диплом - значит избежать продолжительного процесса обучения и не теряя времени перейти к личным целям, будь то поступление в университет или начало профессиональной карьеры.
http://diplomany.ru
Posted by: Gichardgrash | May 9, 2024 5:44 AM
вход - kraken19, кракен официальный сайт
Posted by: Stuarthef | May 9, 2024 6:05 AM
Автобусы Миллерово - Междугородние перевозки Ростов-на-Дону, Пассажирские перевозки Челябинск
Posted by: DonaldBog | May 9, 2024 6:49 AM
The simplest way to use Jaxx Lightweight Web Wallet Jaxxify jaxx wallet login
Posted by: Robertged | May 9, 2024 12:46 PM
В современном мире, где диплом - это начало успешной карьеры в любом направлении, многие ищут максимально быстрый и простой путь получения образования. Факт наличия официального документа сложно переоценить. Ведь именно он открывает дверь перед любым человеком, желающим начать трудовую деятельность или учиться в университете.
Наша компания предлагает оперативно получить этот необходимый документ. Вы имеете возможность купить диплом нового или старого образца, что является удачным решением для человека, который не смог завершить образование, потерял документ или хочет исправить свои оценки. дипломы производятся с особой аккуратностью, вниманием ко всем деталям, чтобы на выходе получился документ, 100% соответствующий оригиналу.
Преимущества подобного решения заключаются не только в том, что вы оперативно получите свой диплом. Весь процесс организовывается удобно и легко, с нашей поддержкой. Начиная от выбора подходящего образца документа до точного заполнения личных данных и доставки по стране — все находится под абсолютным контролем качественных специалистов.
Для тех, кто пытается найти оперативный способ получения необходимого документа, наша компания предлагает отличное решение. Приобрести диплом - это значит избежать долгого процесса обучения и не теряя времени переходить к достижению личных целей, будь то поступление в ВУЗ или начало профессиональной карьеры.
http://diplomexpress.ru
Posted by: Shanesaugs | May 9, 2024 3:26 PM
В современном мире, где диплом является началом удачной карьеры в любой сфере, многие пытаются найти максимально простой путь получения качественного образования. Наличие официального документа переоценить просто невозможно. Ведь диплом открывает двери перед любым человеком, который стремится начать профессиональную деятельность или учиться в любом университете.
Мы предлагаем максимально быстро получить этот необходимый документ. Вы можете приобрести диплом, и это является удачным решением для всех, кто не смог закончить обучение, потерял документ или желает исправить плохие оценки. дипломы производятся аккуратно, с особым вниманием к мельчайшим нюансам. В итоге вы получите документ, 100% соответствующий оригиналу.
Превосходство такого подхода состоит не только в том, что можно оперативно получить диплом. Процесс организовывается удобно, с профессиональной поддержкой. От выбора необходимого образца диплома до правильного заполнения личной информации и доставки по стране — все под полным контролем наших мастеров.
Всем, кто ищет оперативный способ получить требуемый документ, наша компания может предложить выгодное решение. Заказать диплом - значит избежать длительного процесса обучения и не теряя времени переходить к достижению личных целей, будь то поступление в университет или начало профессиональной карьеры.
http://99diplomov.ru
Posted by: StephenVog | May 9, 2024 5:46 PM
Hi this is somewhat of off topic but I was wondering if blogs use WYSIWYG editors or if you have to manually code with HTML. I'm starting a blog soon but have no coding experience so I wanted to get advice from someone with experience. Any help would be enormously appreciated!
www.diplom07.ru
Posted by: LewisJetty | May 9, 2024 8:38 PM
В нашем обществе, где диплом становится началом отличной карьеры в любом направлении, многие ищут максимально простой путь получения качественного образования. Важность наличия официального документа об образовании трудно переоценить. Ведь именно диплом открывает двери перед любым человеком, который хочет вступить в профессиональное сообщество или продолжить обучение в каком-либо ВУЗе.
В данном контексте мы предлагаем очень быстро получить любой необходимый документ. Вы имеете возможность заказать диплом, и это является выгодным решением для человека, который не смог закончить обучение, потерял документ или желает исправить плохие оценки. дипломы выпускаются с особой тщательностью, вниманием ко всем элементам, чтобы на выходе получился 100% оригинальный документ.
Преимущества такого решения заключаются не только в том, что вы сможете быстро получить диплом. Процесс организован комфортно, с нашей поддержкой. Начиная от выбора необходимого образца диплома до консультаций по заполнению личных данных и доставки по России — все находится под полным контролем опытных мастеров.
Для тех, кто ищет максимально быстрый способ получить необходимый документ, наша компания готова предложить выгодное решение. Заказать диплом - это значит избежать долгого обучения и не теряя времени переходить к достижению своих целей, будь то поступление в ВУЗ или начало трудовой карьеры.
http://diplom-msk.ru
Posted by: Dichaelciree | May 10, 2024 5:38 AM
В нашем обществе, где диплом становится началом успешной карьеры в любой области, многие пытаются найти максимально быстрый и простой путь получения образования. Факт наличия официального документа об образовании переоценить невозможно. Ведь диплом открывает дверь перед людьми, желающими вступить в профессиональное сообщество или продолжить обучение в высшем учебном заведении.
Предлагаем очень быстро получить любой необходимый документ. Вы сможете купить диплом нового или старого образца, и это будет выгодным решением для всех, кто не смог завершить обучение, утратил документ или желает исправить плохие оценки. Все дипломы изготавливаются с особой аккуратностью, вниманием к мельчайшим элементам, чтобы в результате получился полностью оригинальный документ.
Плюсы данного подхода заключаются не только в том, что вы сможете максимально быстро получить свой диплом. Весь процесс организовывается просто и легко, с профессиональной поддержкой. От выбора требуемого образца диплома до грамотного заполнения личных данных и доставки в любой регион страны — все находится под абсолютным контролем квалифицированных мастеров.
Всем, кто ищет быстрый и простой способ получения требуемого документа, наша услуга предлагает выгодное решение. Заказать диплом - значит избежать длительного процесса обучения и сразу переходить к достижению личных целей, будь то поступление в ВУЗ или старт карьеры.
diplom-gotovie.ru
Posted by: SDichaelciree | May 10, 2024 5:43 AM
по вызову девочки - Феи Москвы, девочки москвы
Posted by: Timothytox | May 10, 2024 10:15 AM
Трансфер Стерлитамак - Автобусы Пенза, Междугородние такси Бузулук
Posted by: Davidepito | May 10, 2024 10:40 AM
В наше время, когда диплом - это начало отличной карьеры в любом направлении, многие стараются найти максимально быстрый путь получения образования. Факт наличия официального документа об образовании переоценить невозможно. Ведь диплом открывает дверь перед каждым человеком, который желает начать профессиональную деятельность или продолжить обучение в любом университете.
В данном контексте мы предлагаем максимально быстро получить этот важный документ. Вы имеете возможность приобрести диплом нового или старого образца, и это является выгодным решением для человека, который не смог закончить обучение или утратил документ. Любой диплом изготавливается с особой аккуратностью, вниманием к мельчайшим элементам, чтобы в результате получился продукт, 100% соответствующий оригиналу.
Преимущества данного подхода состоят не только в том, что вы сможете быстро получить свой диплом. Процесс организовывается удобно, с нашей поддержкой. От выбора необходимого образца до консультации по заполнению персональных данных и доставки по России — все под абсолютным контролем опытных специалистов.
В итоге, для тех, кто пытается найти быстрый и простой способ получить необходимый документ, наша компания предлагает отличное решение. Приобрести диплом - значит избежать продолжительного обучения и сразу переходить к своим целям, будь то поступление в ВУЗ или старт профессиональной карьеры.
http://ab-diplom.ru
Posted by: EarnestPRINI | May 10, 2024 11:36 AM
Its like you read my mind! You appear to know so much about this, like you wrote the book in it or something. I think that you could do with a few pics to drive the message home a bit, but other than that, this is fantastic blog. An excellent read. I will definitely be back.
https://server-attestats.com /
Posted by: Fobertfleld | May 10, 2024 2:54 PM
В современном мире, где диплом - это начало удачной карьеры в любой области, многие ищут максимально простой путь получения качественного образования. Важность наличия официального документа об образовании переоценить просто невозможно. Ведь диплом открывает дверь перед любым человеком, который желает начать профессиональную деятельность или учиться в высшем учебном заведении.
В данном контексте мы предлагаем оперативно получить этот важный документ. Вы сможете заказать диплом старого или нового образца, что будет выгодным решением для человека, который не смог закончить образование, утратил документ или желает исправить плохие оценки. дипломы выпускаются с особой тщательностью, вниманием ко всем деталям. В результате вы получите полностью оригинальный документ.
Превосходство подобного подхода заключается не только в том, что можно оперативно получить диплом. Весь процесс организовывается просто и легко, с профессиональной поддержкой. Начав от выбора требуемого образца до правильного заполнения личной информации и доставки в любой регион страны — все под полным контролем наших специалистов.
В итоге, для всех, кто пытается найти быстрый способ получить необходимый документ, наша компания может предложить отличное решение. Приобрести диплом - это значит избежать долгого процесса обучения и сразу перейти к достижению своих целей, будь то поступление в университет или начало карьеры.
www.man-attestats24.com
Posted by: OLanesaugs | May 10, 2024 6:08 PM
Сегодня, когда диплом - это начало удачной карьеры в любой сфере, многие ищут максимально быстрый путь получения образования. Наличие официального документа сложно переоценить. Ведь диплом открывает двери перед любым человеком, который собирается начать профессиональную деятельность или учиться в каком-либо институте.
Предлагаем максимально быстро получить этот важный документ. Вы сможете купить диплом, что становится отличным решением для всех, кто не смог закончить образование, потерял документ или хочет исправить плохие оценки. Все дипломы изготавливаются аккуратно, с особым вниманием ко всем деталям. На выходе вы сможете получить документ, 100% соответствующий оригиналу.
Преимущества подобного подхода заключаются не только в том, что вы максимально быстро получите свой диплом. Процесс организовывается удобно, с профессиональной поддержкой. От выбора необходимого образца диплома до консультаций по заполнению личных данных и доставки по стране — все под полным контролем опытных мастеров.
Всем, кто хочет найти быстрый способ получить необходимый документ, наша компания может предложить отличное решение. Приобрести диплом - значит избежать долгого обучения и сразу перейти к достижению собственных целей, будь то поступление в университет или начало профессиональной карьеры.
http://vuzdiploma.ru
Posted by: StephenVog | May 10, 2024 6:13 PM
visit this site right here https://trezorwallet-app.org/2022/08/08/securely-store-multiple-cryptocurrencies-with-trezor-io/
Posted by: ThomasScavy | May 11, 2024 2:05 AM
https://nowosty.mirtesen.ru/blog/43395264005/Mozhno-li-stavit-kvadratnyie-nomera-na-mashinu-Kogda-oni-neobhod
Posted by: Robertged | May 11, 2024 2:50 AM
click here to find out more https://debank.at/2023/11/11/a-deep-dive-into-the-integration-of-metamask-and-debank-exploring-their-combined-features-and-functionalities/
Posted by: RichardPaype | May 11, 2024 5:09 AM
Hello dear friend, I would like to offer placement of your link (or links) on different platforms of the internet such as: forums, blogs, comments and much more. . .
Increase your Visibility Boost Your Seo Rank - Get Organic Traffic From Google. Ranking in Google isn’t hard. All you need is a healthy number of backlinks from referring domains that have authority and trust in Google’s eyes.
This Backlinks Service Benefits:
1. Easily get Google rankings
2. Get a lot of traffic from Google
3. You can earn from the website in different ways
4. Increase Domain Authority (DA)
Quality guaranteed !
PRICE - 20$
WebSite - https://goo.su/CHM5cJW
Posted by: Dudleymog | May 11, 2024 5:36 AM
В нашем обществе, где диплом является началом успешной карьеры в любом направлении, многие ищут максимально простой путь получения образования. Факт наличия официального документа об образовании трудно переоценить. Ведь диплом открывает дверь перед всеми, кто стремится вступить в сообщество квалифицированных специалистов или продолжить обучение в любом университете.
В данном контексте мы предлагаем оперативно получить этот важный документ. Вы сможете купить диплом, и это является удачным решением для человека, который не смог закончить обучение или потерял документ. дипломы выпускаются аккуратно, с максимальным вниманием ко всем деталям, чтобы в результате получился 100% оригинальный документ.
Преимущество подобного подхода заключается не только в том, что вы сможете оперативно получить диплом. Весь процесс организован просто и легко, с нашей поддержкой. От выбора подходящего образца диплома до грамотного заполнения персональных данных и доставки по стране — все находится под абсолютным контролем квалифицированных мастеров.
Всем, кто пытается найти быстрый способ получить необходимый документ, наша компания предлагает отличное решение. Купить диплом - значит избежать длительного процесса обучения и сразу перейти к достижению своих целей, будь то поступление в университет или начало профессиональной карьеры.
russa24-attestats.com
Posted by: Lhanesaugs | May 11, 2024 6:07 AM
В современном мире, где диплом является началом отличной карьеры в любой сфере, многие ищут максимально простой путь получения образования. Наличие документа об образовании переоценить невозможно. Ведь именно он открывает дверь перед людьми, стремящимися вступить в профессиональное сообщество или продолжить обучение в ВУЗе.
В данном контексте наша компания предлагает оперативно получить этот важный документ. Вы имеете возможность заказать диплом нового или старого образца, что становится удачным решением для всех, кто не смог завершить обучение, утратил документ или хочет исправить плохие оценки. Все дипломы выпускаются с особой аккуратностью, вниманием ко всем нюансам. В результате вы сможете получить 100% оригинальный документ.
Плюсы данного решения состоят не только в том, что вы сможете максимально быстро получить диплом. Процесс организован просто и легко, с нашей поддержкой. От выбора требуемого образца до грамотного заполнения персональных данных и доставки по стране — все под полным контролем качественных мастеров.
Всем, кто ищет максимально быстрый способ получить необходимый документ, наша компания предлагает отличное решение. Купить диплом - это значит избежать длительного процесса обучения и не теряя времени переходить к своим целям, будь то поступление в университет или начало карьеры.
www.saksx-attestats.ru
Posted by: DavdidPem | May 11, 2024 6:13 AM
https://glob.mirtesen.ru/blog/43410716608/V-Podmoskove-nachnut-vyidavat-avtomobilnyie-nomera-s-novyimi-kod
Posted by: VincentMiz | May 11, 2024 11:47 AM
Hmm is anyone else encountering problems with the pictures on this blog loading? I'm trying to determine if its a problem on my end or if it's the blog. Any feed-back would be greatly appreciated.
http://arusak-attestats.ru
Posted by: Gichardgrash | May 11, 2024 1:48 PM
linked here https://orbiter-finance-bridge.com/2023/11/18/preserving-capital-on-orbiter-finance-with-stop-loss-mechanisms/
Posted by: JoshuaElota | May 11, 2024 11:03 PM
get redirected here https://metamask-wallet-web3.com/2023/11/16/understanding-the-bnb-token-contract-address-in-metamask-a-beginners-guide/
Posted by: Patrickidomb | May 12, 2024 8:00 AM
web https://metamask-wallet.at/2023/11/16/supercharge-your-kcc-network-experience-with-metamask-everything-you-need-to-know/
Posted by: KennethHiext | May 12, 2024 8:41 AM
МСК проститутки devkiru.com
По теме проститутки за 45 Вы на верном пути. Наш надежный интернет сайт представляет превосходный отдых 18 плюс. Здесь представлены: индивидуалки, массажистки, элитные красавицы, частные интим-объявления. А также Вы можете найти желаемую девочку по параметрам: по станции метро, по возрасту, росту, цвету волос, стоимости. Всё для Вашего комфорта.
Posted by: devkiru.com | May 12, 2024 11:58 AM
go to website https://galxe-web3.com/2023/11/19/engage-with-the-hord-app-community-on-galxe-and-elevate-your-experience/
Posted by: Ronaldvex | May 12, 2024 3:01 PM
check my blog https://1inch-dex.com/2023/11/17/the-evolving-landscape-of-decentralized-finance-a-focus-on-the-1inch-app/
Posted by: CurtisThica | May 12, 2024 3:42 PM
look at this site https://1inch-app.org/2023/11/17/the-role-of-1inch-tokens-in-reducing-transaction-costs-in-the-crypto-market/
Posted by: IsaacNug | May 12, 2024 9:29 PM
i was reading this https://orbiter-finance.at/2023/11/19/orbiter-finances-cross-rollup-bridge-facilitating-seamless-asset-transfers-on-ethereum/
Posted by: Jamesglunk | May 13, 2024 2:17 AM
РєСЂСѓРёР· РІ СЏРїРѕРЅРёСЋ
Posted by: KevinGic | May 13, 2024 4:28 AM
круиз по персидскому заливу из дубай
Posted by: ArnoldVed | May 13, 2024 7:50 AM
круизы москва санкт петербург 2024
Posted by: CharlesCet | May 13, 2024 10:29 AM
kraken официальные ссылки - kraken darknet, kraken
Posted by: Michaeljoith | May 13, 2024 1:48 PM
Приглашаем моделей из Санкт-Петербурга для работы в студии с еженедельными выплатами заработай веб кам моделью в вебкам студии
Posted by: Danieldaurf | May 13, 2024 1:57 PM
kraken ссылка тор - kraken, kraken сайт
Posted by: Loganwreri | May 13, 2024 2:09 PM
kraken onion ссылка - kraken сайт зеркала, kraken onion ссылка
Posted by: Rodneyjep | May 13, 2024 2:12 PM
круизы на соловки 2024
Posted by: StanleyZef | May 13, 2024 2:32 PM
В нашем мире, где диплом - это начало успешной карьеры в любой сфере, многие ищут максимально простой путь получения образования. Наличие официального документа переоценить просто невозможно. Ведь диплом открывает дверь перед любым человеком, который стремится начать профессиональную деятельность или продолжить обучение в любом университете.
Наша компания предлагает оперативно получить этот важный документ. Вы имеете возможность заказать диплом нового или старого образца, что является отличным решением для всех, кто не смог закончить обучение или утратил документ. Каждый диплом изготавливается с особой тщательностью, вниманием ко всем элементам. В итоге вы сможете получить 100% оригинальный документ.
Превосходство этого решения состоит не только в том, что можно оперативно получить диплом. Весь процесс организован комфортно, с профессиональной поддержкой. От выбора требуемого образца до консультации по заполнению персональных данных и доставки по России — все будет находиться под полным контролем опытных специалистов.
Таким образом, для всех, кто пытается найти быстрый и простой способ получения требуемого документа, наша компания может предложить отличное решение. Приобрести диплом - это значит избежать долгого процесса обучения и сразу переходить к своим целям: к поступлению в ВУЗ или к началу удачной карьеры.
diploman-russia.ru
Posted by: Fobertfleld | May 13, 2024 5:20 PM
Thanks for sharing your thoughts. I really appreciate your efforts and I will be waiting for your further write ups thank you once again.
https://maps.google.hn/url?q=https://undress-ai.cc
Posted by: EarnestPRINI | May 13, 2024 5:58 PM
I was in reality itching to get some wager some money on some sports matches that are phenomenon fitting now. I wanted to let you guys recall that I did spot what I consider to be the trounce orientation in the USA.
If you fall short of to bring back in on the spirit, check it out: https://gamblinghub.pro/
Posted by: Wllkeimor | May 14, 2024 12:09 AM
Hi mates, how is all, and what you would like to say regarding this article, in my view its actually remarkable in support of me.
Гума люка для пральних машин Samsung (DC64-03203A)
Posted by: Ismaelanode | May 14, 2024 2:41 AM
interesting news
_________________
онлайн казино рублях
Posted by: Michaelbumma | May 14, 2024 2:41 AM
Профилегибочный станок ПТЭ 500
Posted by: Davidevaby | May 14, 2024 3:24 AM
Перепланировка помещений — это сложный и ответственный процесс, требующий профессионального подхода. Компания "КитСтрой" предоставляет полный спектр услуг по перепланировке помещений в Москве. Наши специалисты разрабатывают индивидуальные проекты, учитывая все строительные нормы и пожелания клиентов. Мы выполняем все этапы работ: от замеров и проектирования до согласования и реализации. Гарантируем высокое качество и надежность всех работ.
Перепланировка помещений с "КитСтрой" — это комфорт и функциональность вашего пространства.
Posted by: RileyFring | May 14, 2024 6:57 AM
как зайти на kraken - kraken ссылка tor, какой адрес кракен
Posted by: Stephenlem | May 14, 2024 8:53 AM
mega555 зеркало рабочее - https mega sb, почему не работает mega sb
Posted by: JosephMon | May 14, 2024 8:56 AM
Hi there,
My name is Mike from Monkey Digital,
Allow me to present to you a lifetime revenue opportunity of 35%
That's right, you can earn 35% of every order made by your affiliate for life.
Simply register with us, generate your affiliate links, and incorporate them on your website, and you are done. It takes only 5 minutes to set up everything, and the payouts are sent each month.
Click here to enroll with us today:
https://www.monkeydigital.org/affiliate-dashboard/
Think about it,
Every website owner requires the use of search engine optimization (SEO) for their website. This endeavor holds significant potential for both parties involved.
Thanks and regards
Mike James
Monkey Digital
Posted by: Mike James | May 14, 2024 9:41 AM
http blacksprut - blecksprut, маркетплейс блэкспрут
Posted by: Matthewbut | May 14, 2024 10:35 AM
mega как зайти - мега даркнет, мега официальный сайт
Posted by: StanleySok | May 14, 2024 12:27 PM
кракен зеркало - кракен даркнет, kraken зеркало
Posted by: Michaelsal | May 14, 2024 2:09 PM
Что бы выяснить более точно сколько стоит пластиковое окно мы рекомендуем воспользоваться бесплатной услугой вызова замерщика. Привезём образцы. Сделаем расчёт в 3-х вариантах цена окно пластиковое цена
Posted by: Ronaldswere | May 14, 2024 2:09 PM
Перепланировка помещения в Москве требует профессионального подхода и учета всех строительных норм. Специалисты компании "КитСтрой" помогут вам реализовать любые изменения в планировке вашего помещения. Мы разрабатываем индивидуальные проекты, учитываем все особенности и предоставляем полный комплекс услуг — от замеров до окончательной сдачи объекта.
Важным этапом является перепланировка помещения Москва, что позволяет создать функциональное и удобное пространство. Обратитесь к нам, чтобы получить качественный результат и соблюдение всех норм.
Posted by: BrianIdoma | May 14, 2024 3:19 PM
mega onion - mega sb как зайти на сайт, мега сб что это
Posted by: Ernestkig | May 14, 2024 4:05 PM
blog link atom wallet
Posted by: Robertpup | May 14, 2024 4:17 PM
дом под ключ -цена -каркасный -строительство -проект -казань -недорого -строить -кирпичный - построить кирпичный дом, проекты домов под ключ
Posted by: Stephenfeeva | May 14, 2024 8:52 PM
have a peek at this website sollet wallet
Posted by: DannyLog | May 14, 2024 10:36 PM
на этом сайте https://battlepass.ru/service/honkai
Posted by: DavidRah | May 15, 2024 12:49 AM
Among these, certain icons stand out for their ubiquitous presence and importance in our daily digital interactions. This article delves into a variety of such icons, including the email, safari (web browser), location, discord, settings, Christmas, messages, spotify, person, and home icons, exploring their significance and evolution person icon
Posted by: Leonardcap | May 15, 2024 3:37 AM
In our approach to minimizing vibration in printing rollers, the use of Vibromera's Balanset-1A facilitated immediate improvements, enhancing print quality. More on the applied solutions can be accessed on Vibromera's website https://vibromera.eu/example/modernization-of-an-old-balancing-machine-with-hard-bearing-supports/
Posted by: Jordanjoith | May 15, 2024 7:32 AM
Стоимость согласования перепланировки в Москве может варьироваться в зависимости от множества факторов: сложности проекта, площади помещения, необходимых согласований с различными инстанциями. Компания "КитСтрой" предлагает услуги по доступным ценам, обеспечивая прозрачное ценообразование и индивидуальный подход к каждому клиенту.
Для того чтобы узнать точную стоимость согласования перепланировки в Москве, обратитесь к нашим специалистам. Мы предоставим вам детальную смету и гарантируем качественное выполнение всех работ.
Posted by: как узаконить перепланировку | May 15, 2024 8:18 AM
Эй! Твой холодильник нуждается в ремонте? Обращайся в наш Гаггенау сервисный центр Москва. Мы сделаем все возможное, чтобы техника снова работала как новая!
Posted by: MichaelErymn | May 15, 2024 8:27 AM
kraken darknet market - kraken onion, kraken ссылка тор
Posted by: Henryglype | May 15, 2024 12:52 PM
круизы из Шанхая
Posted by: Brucetiree | May 15, 2024 1:00 PM
dark website https://mydarkmarket.com/ - tor market url darkmarket list
Posted by: NikeFlity | May 15, 2024 4:01 PM
круизы из сингапура
Posted by: Brucetiree | May 15, 2024 4:08 PM
pop over to these guys Free cc
Posted by: PeterWaife | May 15, 2024 4:47 PM
More about the author Free cards BidenCash
Posted by: TimothyGoant | May 15, 2024 5:33 PM
Bonuses Best cc shop
Posted by: RandyAnaky | May 15, 2024 7:30 PM
darkmarket list https://mydarkmarket.com/ - dark web search engines dark web market
Posted by: NikeFlity | May 15, 2024 8:06 PM
круизы из стамбула
Posted by: TerryFoemi | May 16, 2024 4:02 AM
Bs.gl - Блэкспрут зеркало, Блэкспрут зеркало
Posted by: TimothyIndet | May 16, 2024 4:03 AM
Телесериал «911 служба спасения» про доблестных и храбрых спасателей, служба которых тяжелая и несет опасность с каждым вызовом. Герои службы 911 все смелые и храбрые в каждой серии сериала спасают жизни людям. Все персонажи сериала разные по характеру, но это не мешает им дружить и работать слаженной командой Тут
Posted by: Irvinbug | May 16, 2024 4:06 AM
click here now bidencash
Posted by: WilliamBob | May 16, 2024 7:06 AM
таруса поленово расписание
Posted by: RonaldRaf | May 16, 2024 7:25 AM
find this Potplayer
Posted by: TimothyFat | May 16, 2024 8:59 AM
Onion сайты - Народный путеводитель Даркнета, Даркнет 2024
Posted by: Bryanbix | May 16, 2024 10:27 AM
Бронирование билетов на автобус Дебальцево - Ростов онлайн без предоплат. Актуальное расписание и цены на рейсы автобус шахтерск липецк
Posted by: KeithskiSt | May 16, 2024 2:12 PM
why not try these out Credit cards
Posted by: AndrewDex | May 16, 2024 2:31 PM
этот контент https://battlepass.ru/service/apex
Posted by: IrvingMig | May 16, 2024 4:22 PM
my response Best cc shop
Posted by: Gregorykathy | May 16, 2024 5:25 PM
try this out Potplayer download
Posted by: Frankexott | May 16, 2024 5:45 PM
Нефтегазовое и нефтехимическое оборудование. Данное оборудование используется для разведки газовых и нефтяных месторождений, переработки нефти и природных горючих газов. При его разработке специалисты К5 моделируют все характеристики технологического процесса с помощью программного обеспечения, что гарантирует качество и простоту эксплуатации поставляемых изделий Производство и продажа нефтегазового и нефтехимического оборудования (емкостное и теплообменное оборудование)
Posted by: Russelbreap | May 16, 2024 6:53 PM
Блэкспрут зеркало - Bs2site, Bs.gl
Posted by: RobinKew | May 16, 2024 7:54 PM
Список Tor сайтов - Даркнет 2024, Народный путеводитель Даркнета
Posted by: AdolphMaser | May 16, 2024 7:55 PM
mold remediation training https://www.jotform.com/241063566693058 online chemist pharmacy
Posted by: WilliamRob | May 16, 2024 11:27 PM
It is truly a well-researched piece of writing and excellent wording. I got so engaged in this post that I finished it in one go. Really impressed with your work and skill. Missguided Discount Codes
Posted by: Top Vouchers Code | May 17, 2024 5:45 AM
Простыми словами о том, что нужно знать прежде, чем заказать свидание на крыше свидание на крыше москва
Posted by: FrankCal | May 17, 2024 6:00 AM
Даркнет 2024 - Даркнет 2024, Onion сайты
Posted by: Georgeron | May 17, 2024 9:11 AM
В эпоху цифровых технологий, когда виртуальная реальность становится все более неотъемлемой частью нашей повседневной жизни, мир развлечений и азарта находит свое отражение в многочисленных онлайн площадках, предлагающих азартные игры и возможность погрузиться в атмосферу адреналина и выигрышей http://kzgbi-2.ru/forums.php?m=posts&q=7480&n=last#bottom
Posted by: Michaelowete | May 18, 2024 2:36 AM
dark web search engines https://mydarknetmarketlinks.com/ - dark web market links onion market
Posted by: NikeFlity | May 18, 2024 4:02 AM
В нашем обществе, где аттестат - это начало удачной карьеры в любом направлении, многие ищут максимально простой путь получения образования. Факт наличия официального документа сложно переоценить. Ведь диплом открывает двери перед любым человеком, желающим начать профессиональную деятельность или учиться в ВУЗе.
Наша компания предлагает максимально быстро получить этот важный документ. Вы сможете приобрести аттестат нового или старого образца, что будет выгодным решением для человека, который не смог завершить образование или потерял документ. Аттестат изготавливается аккуратно, с особым вниманием ко всем нюансам, чтобы в результате получился полностью оригинальный документ.
Преимущества такого решения состоят не только в том, что вы сможете быстро получить свой аттестат. Процесс организовывается удобно и легко, с нашей поддержкой. От выбора нужного образца до консультаций по заполнению личных данных и доставки в любой регион страны — все под абсолютным контролем опытных мастеров.
В результате, всем, кто пытается найти оперативный способ получить необходимый документ, наша компания готова предложить отличное решение. Купить аттестат - это значит избежать долгого процесса обучения и не теряя времени переходить к своим целям: к поступлению в университет или к началу удачной карьеры.
https://diplomans-rossians.com/
Posted by: Thomasfiz | May 18, 2024 8:32 AM
Проверенные сайты Даркнета - Даркнет поисковик, Проверенные сайты Даркнета
Posted by: Patricktut | May 18, 2024 10:56 AM
Даркнет 2024 - Даркнет поисковик, Список сайтов Даркнета
Posted by: AntonioIdomi | May 18, 2024 11:12 AM
Даркнет поисковик - Даркнет поисковик, Список Tor сайтов
Posted by: Jimmyvem | May 18, 2024 12:53 PM
Поможем получить гражданство Армении "под ключ".
Договор, гарантии. Консультация бесплатная!
Свяжитесь с нами в telegram: @migrapractice
Posted by: Josephnab | May 18, 2024 7:49 PM
Slightly off topic :)
It so happened that my sister found an interesting man here, and recently got married ^_^
(Moderator, don't troll!!!)
Is there are handsome people here! ;) I'm Maria, 28 years old.
I work as a model, successfull - I hope you do too! Although, if you are very good in bed, then you are out of the queue!)))
By the way, there was no sex for a long time, it is very difficult to find a decent one...
And no! I am not a prostitute! I prefer harmonious, warm and reliable relationships. I cook deliciously and not only ;) I have a degree in marketing.
My photo:
___
Added
The photo is broken, sorry(((
Check out my blog where you'll find lots of hot information about me:
https://auditorius.ru
Or write to me in telegram @Lolla_sm1_best ( start chat with your photo!!!)
Posted by: DanaEvima | May 18, 2024 9:42 PM
mega sb даркнет - m3ga вход, m3ga точка gl
Posted by: Kennethsluse | May 19, 2024 3:54 AM
маркетплейс блэкспрут - blacksprut вход, blecksprut
Posted by: ArturoCitty | May 19, 2024 4:19 AM
kraken ссылка tor - kraken onion ссылка, kraken сайт
Posted by: LarryCergo | May 19, 2024 4:30 AM
что такое площадка мега - официальная ссылка на mega sb, m3ga.at
Posted by: Jameszigue | May 19, 2024 6:37 AM
Лизинг всех видов ИТ, телекоммуникационного оборудования и средств связи. средства слежения и видеонаблюдения; системы хранения данных лизинг косметологического оборудования
Posted by: MichaelWoobe | May 19, 2024 6:43 AM
kraken11 - kraken ссылка, kraken ссылка
Posted by: VirgilIcoth | May 19, 2024 7:09 AM
darkmarket https://mydarknetmarketlinks.com/ - onion market darknet websites
Posted by: NikeFlity | May 19, 2024 8:53 AM
don't think anything
_________________
1xbet games официальный сайт
Posted by: Jameselall | May 19, 2024 10:15 AM
acne treatment job - acne medication rob acne treatment load
Posted by: Rxjwms | May 19, 2024 3:24 PM
Личный тренер, групповые занятия, безлимитный доступ, тренажерный зал, шкафчик в раздевалке! Оплата только за месяц фитнес центр киевская
Posted by: Williejat | May 19, 2024 5:06 PM
Сломался старый ноутбук, а новый срочно нужен для работы. Денег впритык, поэтому начал искать способы быстро занять средства. На телеграм-канале новые и малоизвестные МФО 2024 нашёл множество новых МФО, которые дают займы на карту без отказа. Понравилось, что можно получить деньги даже ночью и с плохой кредитной историей. Оформил заявку, деньги пришли моментально. Теперь у меня новый ноутбук и работа идёт без перебоев. Советую всем, кому нужны срочные деньги!
Posted by: MfoklaUtica | May 19, 2024 5:42 PM
не работает сайт m3ga gl - https m3ga at, https m3ga gl
Posted by: RussellBloop | May 19, 2024 8:13 PM
как зайти на kraken - официальное зеркало kraken, ссылка КРАКЕН не работает
Posted by: Stanleysaw | May 19, 2024 9:15 PM
сайт мега сб не работает - как зайти на мега, m3ga.at
Posted by: DavidVon | May 19, 2024 9:27 PM
asthma medication problem - inhalers for asthma express asthma medication busy
Posted by: Dtopxx | May 19, 2024 10:29 PM
маркетплейс блэкспрут - маркетплейс блэкспрут, blacksprut вход
Posted by: ThomasDot | May 19, 2024 10:48 PM
kraken сайт - kraken зеркало, kraken сайт
Posted by: Sammietig | May 19, 2024 11:28 PM
dark web search engine https://mydarknetmarketlinks.com/ - tor market links blackweb
Posted by: NikeFlity | May 19, 2024 11:40 PM
acne treatment propose - acne treatment represent acne medication greet
Posted by: Snlktj | May 20, 2024 2:40 AM
buy online
Posted by: JoshuaClies | May 20, 2024 3:00 AM
(palimpsests). In the XIII-XV centuries in
Posted by: EOTechhbu | May 20, 2024 4:00 AM
Кроме привычного фитнеса, мы устраиваем челленджи и соревнования среди сети, проводим открытые тренировки в центре и устраиваем сайкл-забеги в торговых центрах тренажерный зал войковская
Posted by: Melvinscesy | May 20, 2024 5:59 AM
Hello everybody, here every one is sharing these knowledge, therefore it's good to read this website, and I used to pay a visit this webpage everyday.
https://images.google.co.cr/url?q=https://blog.nudify.online
Posted by: Shanesaugs | May 20, 2024 1:32 PM
Bernedoodle Puppies For Sale In Georgia
Posted by: DennisHoche | May 20, 2024 2:17 PM
Delving into tall 3D reproduction with Straight Rail Printers
Straight rail printers are acquiring popularity among 3D publishing fans and pros for their exceptional speed, precision, and print quality. These printers utilize direct rail networks instead of conventional linear rods or perhaps belts for movement manipulation, leading to smoother movement, diminished oscillation, and heightened print speeds. By diminishing friction and backlash, linear rail printers can achieve higher accelerations and jerk configurations, allowing faster print speeds without compromising print excellence otherwise accuracy. Furthermore, straight rail printers are appropriate with a broad range of wire types and snout sizes, leading them to be flexible tools for specific methods. regardless of whether employed for rapid prototyping, small set manufacture, to expressive tasks, linear rail printers present unmatched speed and abilities for demanding 3D printing assignments.
Flying Bear LaserMan 40W
Learn the features of GT2560 printer boards and primary functions for superior print quality. 1592cce
Posted by: NicholeMoist | May 20, 2024 2:33 PM
Актуальные купоны на скидку iHerb совершенно бесплатно! ... Вы находитесь на странице iHerb, который на данный момент не работает айхерб промокод на первый заказ
Posted by: KeithHet | May 20, 2024 4:11 PM
Bernedoodle Tri Color Puppies For Sale
Posted by: DennisHoche | May 20, 2024 4:12 PM
Купить Мефедрон в Москве? САЙТ - KOKS.CC
Купить в Москве Мефедрон 24/7 САЙТ - https://koks.cc/
Posted by: Glenntikmus | May 20, 2024 4:21 PM
m3ga at - как зайти на mega, m3ga gl зеркало
Posted by: Williamcen | May 21, 2024 12:51 AM
Топ 10 лучших онлайн-казино, которые заняли первые места в рейтинге игр на наличные деньги, доверия и других значимых критериев 2024 топ казино
Posted by: WilliamEvets | May 21, 2024 12:54 AM
мега сб даркнет - mega работает, мега новая ссылка
Posted by: GlennNoiny | May 21, 2024 2:27 AM
blacksprut вход - blacksprut вход, blacksprut зеркало официальный
Posted by: MichaelCor | May 21, 2024 3:39 AM
kraken darknet - kraken darknet, кракен
Posted by: Waynetriva | May 21, 2024 4:26 AM
официальное зеркало kraken - тор kraken, КРАКЕН как зайти
Posted by: JeffreyMurry | May 21, 2024 5:27 AM
check jaxx wallet liberty
Posted by: Aaronstymn | May 21, 2024 6:16 AM
see this website sollet io
Posted by: AlfredoBoold | May 21, 2024 7:37 AM
More Help sollet solana
Posted by: Jessiebeaug | May 21, 2024 9:33 AM
click for info jaxx blockchain wallet
Posted by: RichardMub | May 21, 2024 10:09 AM
Добро пожаловать в наш Telegram-канал! Мы составили список малоизвестных МФО, которые сейчас предлагают уникальную акцию – первый займ бесплатно до 15 тысяч рублей. Все быстро и просто: минимальные требования – только паспорт и именная банковская карта. Узнайте больше о новые займы без отказа на карту и получите деньги уже сегодня. Присоединяйтесь к нам и воспользуйтесь выгодным предложением!
Posted by: JamesFup | May 21, 2024 11:44 AM
Создайте свою фотоисторию. Стильные фотокниги в два клика! Сделаем за 3 дня печать фотокниги в москве
Posted by: Peterprowl | May 21, 2024 1:42 PM
Наш Telegram-канал обновляется каждый день, предлагая вам самые свежие предложения от новых МФО. Мы расскажем вам, что такое онлайн займы и как их правильно использовать. Среди наших рекомендаций вы найдете займы новые онлайн с акциями под 0%. Даже если у вас плохая кредитная история, вы сможете получить займ быстро и без отказа. Присоединяйтесь к нам и узнавайте обо всех выгодных предложениях первыми!
Posted by: Dennisabumb | May 21, 2024 4:07 PM
Фотокниги с вашей историей. Закажите сегодня, и через несколько дней будете держать дорогие сердцу воспоминания в руках. Обложка arrow изготовление фотокниг
Posted by: FrancisPaB | May 21, 2024 4:28 PM
Ищете, где можно взять займ быстро и бесплатно? Заходите в наш Telegram-канал! Мы составили список малоизвестных МФО, которые предлагают первый займ бесплатно до 15 тысяч рублей. Минимальные требования – только паспорт и именная банковская карта. Узнайте больше о займы новые онлайн и получите деньги уже сегодня. Подписывайтесь на наш канал и не упустите выгодные акции!
Posted by: Verzaima | May 21, 2024 6:25 PM
resource Rufus download
Posted by: Jamestok | May 21, 2024 6:57 PM
prostatitis medications ministry - prostatitis treatment hire prostatitis pills moral
Posted by: Oitlzf | May 21, 2024 7:26 PM
оземпик купить спб наличие - трулисити россия, оземпик +на сколько хватает
Posted by: MichaelLiZ | May 21, 2024 7:50 PM
additional info Tor browser
Posted by: RobertJetib | May 21, 2024 8:37 PM
мунжаро купить +в спб - +что лучше оземпик +или саксенда, семаглутид купить +в минске
Posted by: Orlandonon | May 21, 2024 8:50 PM
uti antibiotics steady - uti treatment expression treatment for uti consult
Posted by: Llkpxo | May 21, 2024 11:05 PM
оземпик раствор +для инъекций цены - шприц ручка оземпик, купить оземпик 1
Posted by: Wesleynunda | May 22, 2024 12:34 AM
official site Osu download
Posted by: DouglasRooky | May 22, 2024 12:36 AM
Related Site Pal world download
Posted by: DennisFrila | May 22, 2024 12:39 AM
1Вин абсолютно не идеальное казино, да и как бк тоже имеет недостатки. Тем не менее, я остаюсь в казино и продолжаю играть. Трудности меня закалили, позволили приспособиться 1вин зеркало
Posted by: DanielRoups | May 22, 2024 12:49 AM
тор blacksprut - blacksprut официальный сайт, блэк спрут
Posted by: Phillipelomo | May 22, 2024 2:43 AM
оземпик +или саксенда - трулисити купить дешевле, цена виктоза
Posted by: RobertMiz | May 22, 2024 3:15 AM
This article will explore the legal steps that law firms should take when handling data breach investigations.
The Initial Response
One of the first steps that law firms should take when a data breach occurs is to conduct a thorough investigation. This may involve hiring a forensic expert to determine the scope of the breach and identify the source of the attack. It is important to contain the breach as quickly as possible to prevent further damage.
Law firms should also inform their clients about the breach in a timely manner. This not only helps to build trust with clients, but it is also a legal requirement in many jurisdictions. Failing to notify clients about a data breach can result in severe penalties and lawsuits.
Legal Obligations
Law firms have a legal obligation to protect their clients' information. This includes implementing security measures to prevent data breaches, as well as taking swift action in the event of a breach. Failure to meet these obligations can result in legal consequences, including fines and lawsuits.
Law firms should also be aware of the various regulatory requirements that may apply in the event of a data breach. For example, in the United States, law firms may be subject to state data breach notification laws, as well as federal regulations such as the Health Insurance Portability and Accountability Act (HIPAA) and the Gramm-Leach-Bliley Act.
Working with Law Enforcement
When a data breach occurs, law firms should consider involving law enforcement agencies in the investigation. This can help to identify the perpetrators of the breach and hold them accountable for their actions. Law enforcement agencies may also be able to provide valuable resources and expertise to assist in the investigation.
Working with law enforcement can also help law firms to build a stronger case if they decide to pursue legal action against the perpetrators of the breach. This can help to deter future attacks and protect both the law firm and its clients from further harm.
Data breaches can have severe consequences for law firms, including legal, financial, and reputational damage. By taking swift and decisive action in the event of a breach, law firms can protect themselves and their clients from these risks. This article has outlined some of the key legal steps that law firms should take when handling data breach investigations. By following these steps, law firms can minimize the impact of a breach and demonstrate their commitment to protecting client information.
Remember, prevention is always better than cure – so implementing robust security measures can help to prevent data breaches before they occur. Stay vigilant, stay informed, and stay safe.
Investigate Further: https://medium.com/@scorecred10/understanding-military-discrimination-a1274a4717ce
When it comes to managing data within a corporation, having an effective data retention policy in place is crucial. This policy outlines the guidelines and procedures for retaining and disposing of data in a secure and compliant manner. With the rise of data breaches and privacy concerns, corporations must prioritize data retention to protect sensitive information and comply with regulations.
Posted by: Antonhab | May 22, 2024 3:29 AM
dandruff remedies homemade https://www.jotform.com/241063462161043 cigarette smoke remediation
Posted by: Patrickvaw | May 22, 2024 4:12 AM
Дом для отдыха в Черногории - House rental in Zabljak, Дом в горах Черногории в аренду
Posted by: LoganIrrib | May 22, 2024 5:18 AM
have a peek here galaxy swapper download
Posted by: JerryReami | May 22, 2024 6:50 AM
this page Fl studio download
Posted by: Ollieved | May 22, 2024 8:35 AM
Онлайн-заказ на печать фотографий, фотосувениров и фотокниг, производство интерьерной и полиграфической продукции фотоуслуги печать фотографий
Posted by: WalterTex | May 22, 2024 8:49 AM
Интерьерная печать, печать на холсте, накат на пенокартон, модульные картины, печать на текстильных материалах, с доставкой, оформить онлайн заказ через интернет прямо с нашего сайта накатка на пенокартон фото
Posted by: RobertWekly | May 22, 2024 9:04 AM
Один из лучших буков. Даже наши “легальные” конторы бывают не выплачивают деньги и специально урезают лимиты. 1вин не страдает этой херней, всегда четко выводит, говорю лично про себя! Надеюсь так и будет дальше 1вин казино
Posted by: EnriqueBub | May 22, 2024 6:00 PM
her explanation galaxy swapper
Posted by: JoshuaKar | May 22, 2024 7:24 PM
купить инстаграм аккаунт алматы - купить инстаграм аккаунт для рекламы, купить бизнес аккаунт в инстаграм
Posted by: Ralphisort | May 22, 2024 9:41 PM
bs2web - bs2site, blacksprut зеркала
Posted by: DavidGumma | May 22, 2024 10:50 PM
Осуществляем уборку квартир, домов, офисов, уборку после ремонта, генеральную и поддерживающую, а также мытье окон уборка квартир в минске
Posted by: Elmerhar | May 22, 2024 11:27 PM
магазин аккаунтов вконтакте - likest купоны купить дешево, купить инстаграм аккаунт москва
Posted by: WilliamPoike | May 23, 2024 5:29 AM